Stjarnvísindafélag Íslands hefur nýlega opnað sérstaka YouTube-síðu með viðtölum við sex íslenska raunvísindamenn í opnum aðgangi:
Vonast er til að með tíð og tíma bætist við fleiri viðtöl og annað efni. Ég reikna jafnframt með að fljótlega verði hægt að tengjast þessari síðu í gegnum heimasíðu félagsins.
Í staðinn fyrir að skrifa nýja færslu um Björn Gunnlaugsson hef ég ákveðið birta skrá yfir allt efni sem ég hef tekið saman um þann merka mann og verk hans. Listinn er fyrir neðan myndina og hægt er að nálgast hverja grein með því að smella á viðeigandi titil.
Heimsmynd Björns er sett fram í hinu mikla ljóði hans, Njólu, og ítarlega umfjöllun um hana er að finna í grein minni Björn Gunnlaugsson og náttúruspekin í Njólu frá 2003.
Björn Gunnlaugsson (1788-1876) árið 1859. Hann var þá 71 árs. Teikningin er eftir Sigurð Guðmundsson málara.
Njóla, hið merka heimsmyndarljóð Björns Gunnlaugssonar, kom fyrst út árið 1842. Hér má sjá forsíðu 2. útgáfu frá 1853. Verkið kom svo út í þriðja sinn 1884. Mynd: Wikipedia.
Björn lauk við að mæla Ísland árið 1844 og fyrsta úgáfan af hinu þekkta korti hans kom á prenti 1849.
* Stjarneðlisfræði og heimsfræði á Íslandi: Efnisyfirlit *
Skagfirðingurinn Sturla Einarsson (1879-1974) var fyrsti Íslendingurinn sem hlaut formlega háskólagráðu í stjörnufræði. Hann lauk A.B.-prófi í greininni frá Minnesótaháskóla í Minneapólis árið 1905 og varði síðan doktorsritgerð (Ph.D.) í stjörnufræði við Kaliforníuháskóla í Berkeley 1913. Sturla starfaði aldrei á Íslandi, enda fluttist hann fjögurra ára gamall með foreldrum sínum til Vesturheims þar sem hann bjó til æviloka. Hann telst því vera Vestur-Íslendingur.
Sturla Einarsson stjörnufræðingur í kringum 1930. Mynd: Mary Lea Shane Archives of the Lick Observatory.
Hér verður fjallað stuttlega um Sturlu og verk hans. Upplýsingarnar um nám hans og störf tók ég að mestu saman fyrir langa löngu, eða á árunum 1993-94. Þær eru að verulegu leyti fengnar í gegnum bréfaskipti við bandarísku stjarnvísindamennina Donald E. Osterbrock (1924-2007), John G. Phillips (1917-2001) og Harold F. Weaver (1917-2017) sem og son Sturlu, Alfred W. Einarsson (1915-2009), sem á sínum tíma var prófessor í eðlisfræði við San José State University. Þessir heiðursmenn eru nú allir látnir, en þótt seint sé, þakka ég þeim kærlega fyrir hjálpina.
Auk annarra tilfallandi heimilda hef ég jafnframt stuðst við eftirfarandi minningargreinar og æviskrár:
Áður en lengra er haldið er við hæfi að hlusta á stutt viðtal sem Finnbogi Guðmundsson, þá kennari við Háskólann í Manitóba og síðar landsbókavörður, tók við Sturlu árið 1955.
Sturla fæddist á Grófargili í Langholti í Skagafirði hinn 9. desember 1879. Faðir hans var Jóhann Einarsson (1853-1917), sonur hjónanna Einars Magnússonar bónda í Krossanesi og konu hans Eufemíu Gísladóttur sagnaritara Konráðssonar. Móðir Sturlu var Elín Benónísdóttir (1850-1930) bónda á Beinakeldu í Húnaþingi og konu hans Ingiríðar Árnadóttur.
Tiltölulega nýleg mynd af Grófargili. Gamli bærinn er náttúrulega löngu horfinn. Myndin er úr skýrslunni Fornleifaskráning vegna aðalskipulags á Langholti, Skagafirði eftir Bryndísi Zoëga og Guðnýju Zoëga (des. 2004, bls. 9).
Aðrir synir þeirra Einars og Eufemíu og bræður Jóhanns voru Indriði hagfræðingur og leikritaskáld og séra Gísli í Stafholti.
Eftir að Jóhann og Elín höfðu stundað búskap á Grófargili í fimm ár fluttust þau árið 1882 að Brekku hjá Víðimýri. Árið eftir sigldu þau svo til Vesturheims og gerðust bandarískir ríkisborgarar. Þá var Sturla fjögurra ára og að bandarískum sið var hann ekki lengur Jóhannsson heldur Einarsson.
Fyrsta árið í Bandaríkjunum vann Jóhann sem vinnumaður hjá ýmsum bændum í Norður-Dakóta, en 1884 fluttist fjölskyldan búferlum til borgarinnar Duluth í Minnesóta þar sem tvö börn bættust fljótlega í hópinn, þau Nanna (1884-1969) og Baldur (1886-1962). Nokkru eftir komuna til Duluth setti Jóhann þar upp lítið mjólkurbú, sem hann rak í hátt á annan áratug. Skömmu upp úr aldamótunum 1900 fór búið hins vegar á hausinn og eftir það vann Jóhann hjá vini sínum Kristjáni Jónssyni (Chris Johnson) sem hafði yfirumsjón með Forest Hill kirkjugarðinum í Duluth. Þegar Jóhann lést árið 1917 orti Stephan G. Stephansson eftir hann erfiljóð, sem lesa má hér.
Hluti borgarinnar Duluth í Minnesóta í kringum 1905. Mynd: Shorpy.com.
Sturla fór í barnaskóla sjö ára gamall, einu ári á eftir jafnöldrum sínum. Hann kunni þá ekkert í ensku, því á heimilinu var eingöngu töluð íslenska. Af þeim sökum þurfti hann að vera þrjú ár í fyrsta bekk. Eftir það gekk allt betur og árið 1901 útskrifaðist hann úr menntaskóla, tuttugu og eins árs að aldri. Með skólanum vann hann alla daga sem mjólkursendill hjá föður sínum og einnig í sumarfríum.
Strax að loknum menntaskóla hóf Sturla nám við viðskiptaskóla í Duluth, en átti þar stutta dvöl. Hugur hans stóð til háskólanáms og með góðri aðstoð áðurnefnds Kristjáns Jónssonar tókst honum að komast í raunvísindanám við Minnesótaháskóla í Minneapólis haustið 1901.
Sturla, ungur að árum. Ljósmyndari óþekktur.
Eins og önnur forn menningarsamfélög höfðu hinir fjölmörgu ættflokkar frumbyggja Norður-Ameríku sínar eigin hugmyndir um stjörnuhimininn og gang himintungla og studdust við þær með ýmsum hætti, meðal annars við akuryrkju og veiðar. Þekking á evrópskri stjörnufræði mun hins vegar hafa borist til Vesturheims með breskum landnemum á sextándu öld og á sautjándu öldinni var hún kennd í fyrstu skólum innflytjenda. Um svipað leyti var farið að gefa út almanök. Á seinni hluta átjándu aldar voru fyrstu litlu athugunarstöðvarnar reistar og fór þeim fjölgandi eftir því sem leið á nítjándu öldina. Vart er þó hægt að tala um kerfisbundnar rannsóknir í stjörnufræði fyrr en um og upp úr 1840, þegar W.C. Bond (1789-1859) kom upp fyrstu stjörnuathugunarstöð Harvardháskóla og Bandaríkjastjórn stofnaði sérstaka athugunarstöð sem rekin var af bandaríska flotanum í Washington D.C.
Hopkins stjörnuathugunarstöðin í Williams College í Massachusetts mun vera elsta varðveitta stjörnuathugunarstöðin í Bandaríkjunum, enda reist á árunum 1836-1838. Í júlí 1988 hýsti skólinn IAU-ráðstefnu um stjarnvísindakennslu og myndin sýnir þátttakendur fyrir framan stöðina. Ef grannt er skoðað má greina færsluhöfund í mannfjöldanum. Inni í byggingunni eru ýmis gömul tæki, þar á meðal aðalsjónauki stöðvarinnar, sjö þumlunga linsusjónauki, sem hinn þekkti A. Clark (1804-1887) smíðaði árið 1851 og mun vera einn elsti, ef ekki elsti sjónauki sem hann smíðaði og enn er varðveittur.
Ekkert er um það vitað hvað réði því að Sturla ákvað að leggja fyrir sig stjörnufræði. Hitt er ljóst, að á fyrstu áratugum ævi hans var mikill uppgangur í stjarnvísindum í Bandaríkjunum og á þeim tíma var lagður sá grunnur sem átti eftir að gera Bandaríkin að stórveldi á því sviði á fyrri hluta tuttugustu aldar. Sem kunnugt er hafa þau haldið þeirri stöðu alla tíð síðan.
Ein helsta ástæða þessarar hröðu þróunar var smíði sífellt stærri og betri sjónauka, sem komið var fyrir í nýjum og heppilega staðsettum athugunarstöðvum. Ólíkt því sem gerðist í Evrópu kom fjármagnið til framkvæmdanna að mestu frá auðugum einstaklingum, sem vildu halda nafni sínu og minningu á lofti. Þar fóru fremstir í flokki þeir J. Lick (1796-1876), P. Lowell (1855-1916), C. Yerkes (1837-1905), J.D. Hooker (1838-1911), J.D. Rockefeller (1839-1937) og A. Carnegie (1835-1919).
Að sjáfsögðu komu fjölmargir aðrir að þessari uppbyggingu, en sá sem hafði hvað mest áhrif á þróunina var stjarneðlisfræðingurinn G.E. Hale (1868-1938), sem ekki var aðeins framúrskarandi vísindamaður heldur ótrúlega laginn við sannfæra auðmenn um að leggja fram fjármagn til stjarnvísindarannsókna. Hinn mikli 200 þumlunga (5 m) sjónauki á Palomarfjalli er við hann kenndur.
Myndin sýnir staðsetningu þekktustu stjörnuathugunarstöðva Bandaríkjanna á árunum frá 1887 til 1928. Þær eru í tímaröð: Lick Observatory (1888), Lowell Observatory (1894), Yerkes Observatory (1897), Mount Wilson Observatory (1904) og Palomar Observatory (1928). Kortið er fengið að láni hjá vefsíðunni America’s Historic Observatories. Sjá einnig Wikipediugreinarnar Observatory, List of largest optical reflecting telescopes og List of largest optical refracting telescopes.
Lítið sem ekkert er vitað um dvöl Sturlu við Minnesótaháskóla, nema hvað námið gekk vel. Hann lagði fyrst og fremst stund á stjörnufræði með eðlisfræði og stærðfræði sem aukagreinar. Aðalkennari hans við stjörnufræðideildina var F.P. Leavenworth (1858-1928) og síðustu tvo veturna var Sturla aðstoðarmaður hans, samhliða náminu. Á sumrinn vann hann fyrir sér sem sendill fyrir slátrara í Duluth. Hann lauk A.B.-prófi vorið 1905 með góðum vitnisburði. Hann var þá 25 ára.
Minnesótaháskóli í Minneapólis eins og hann leit út á námsárum Sturlu. Kennsla í stjörnufræði, eðlisfræði og efnafræði mun hafa farið fram í Jones Hall, kassalaga húsinu lengst til vinstri á myndinni. Mynd: Gamalt póstkort.
Aðal stjörnufræðikennari Sturlu í Minnesota, prófessor Francis P. Leavenworth, árið 1900. Mynd: Wikipedia.
Stjörnuathugunarstöð Minnesótaháskóla á námsárum Sturlu. Hún var tekin í notkun 1891 og undir hvelfingunni var 10,5 þumlunga (26,7 cm) linsusjónauki frá fyrirtækinu Warner & Swasey en í flata hluta stöðvarinnar („The Transit House“) var hágöngukíkir. Hvelfingin var síðar sett upp á þak sérhannaðar byggingar, sem nú hýsir eðlisfræði og stjörnufræði háskólans (frekari upplýsingar má nálgast hér; athugið að umfjöllunin nær yfir þrjár síður). Sjá einnig myndirnar hér fyrir neðan.
Linsusjónauki athugunarstöðvarinnar, 10,5 þumlungaar að þvermáli.
Tvær myndir af hágöngukíki stöðvarinnar.
Sturla Einarsson nýútskrifaður frá Minnesótaháskóla vorið 1905. Ljósmyndari óþekktur.
Góður árangur Sturlu í A.B.-náminu varð til þess að haustið 1905 komst hann að sem framhaldsnemi hjá prófessor Armin O. Leuschner (1868-1953) við stjörnufræðideild hins þekkta Kaliforníuháskóla í Berkeley. Deildin var þá til húsa í byggingum, sem gengu undir nafninu Stjörnuathugunarstöð stúdenta (The Students' Observatory, síðar Leuschner Observatory). Fyrstu tvö árin í Berkeley mun Sturla hafa fengið þar lítið herbergi til að búa í. Auk þess réði Leuschner hann strax sem aðstoðarmann (assistant), starf sem Sturla stundaði samhliða náminu. Launin og önnur fríðindi hafa eflaust skipt sköpum fyrir fjárhag hans og gert honum kleift að ljúka doktorsnáminu.
Aðalkennari Sturlu og leiðbeinandi í doktorsnámi, Armin O. Leuschner árið 1930. Teikning eftir P. Van Valkenburgh.
Upphaflega stjörnuathugunarstöðin í Berkeley (The Students' Observatory) árið 1905. Auk nokurra sjónauka og annars tækjabúnaðar var þarna fyrirlestrarsalur, herbergi til að vinna að gagnaúrvinnslu og úreikningum og skrifstofuaðstaða fyrir kennara. Fyrstu byggingarnar voru teknar í notkun árið 1887, en með árunum bættust fleiri við. Árið 1951 var nafninu breytt í Leuschner Observatory og fjórtán árum síðar var stöðin svo lögð niður í þáverandi mynd, flutt til Lafayette í Kaliforníu og rekstrarforminu breytt. Þar er stöðin enn. Frá 1959 hafa stjarnvísindamenn skólans hins vegar haft aðsetur í Campbell Hall. Mynd: Gamalt póstkort.
Leuschner-stöðin árið 1961, fjórum árum áður en hún var flutt til Lafayette. Mynd: IAU News-Bulletin 8, 1961, bls. 1.
Rauða örin á kortinu sýnir staðsetningu stjörnuathugunarstöðvarinnar á háskólalóðinni í Berkeley árið 1911. Kortið er fengið að láni hjá þessari vefsíðu og færsluhöfundur býst ekki við að aðrir en þeir, sem þekkja til í Berkeley nútímans, hafi af því full not. Þar sem stöðin stóð er nú grænt svæði, Observatory Hill, ásamt minningarskildi sem sýndur er á myndinni fyrir neðan:
Í lok nítjándu aldar voru sjónaukar stöðvarinnar tveir. Annars vegar pólstilltur sex þumlunga (15 cm) linsusjónauki með 8,5 feta (2,6 m) brennivídd og hins vegar þriggja þumlunga (8 cm) hágöngukíkir af Davidson gerð. Einnig átti stöðin nokkra sextunga, klukkur af ýmsum gerðum, jarðskjálftmæla og rófsjá.
Árið áður en Sturla hóf nám við deildina hafði verið bætt þar við nýjum sjónaukum og byggt yfir þá, þar á meðal átta þumlunga (20 cm) Newtonssjónauka, smíðaður af Brashear, og 5 þumlunga (13 cm) linsusjónauka með 6,33 feta (1,9 m) brennivídd. Auk þess höfðu eldri byggingar verið endurnýjaðar og sumar stækkaðar. Mikilvægt er að hafa í huga að allir sjónaukarnir voru fyrst og fremst hugsaðir sem kennslusjónaukar.
Þennan átta þumlunga Newtonssjónauka frá Brashear fékk fékk Konunglega kanadíska stjarnvísindafélagið að gjöf árið 1904. Hann gæti verið svipaður að gerð og sá sem Berkeley-stöðin eignaðist sama ár. Því miður hef ég ekki enn fundið neinar myndir af tækjabúnaði stöðvarinnar sjálfrar.
Þegar hin nýja aðstaða var tekin í notkun 1904 hélt W.W. Campell (1862-1938), þáverandi stjórnandi Lick stjörnuathugunarstöðvarinnar, stutt ávarp og sagði þar meðal annars nokkur orð, sem eiga jafn vel við í dag og fyrir rúmri öld (bls. 66-67):
In these days of great things one frequently hears the opinion expressed that for useful investigational work in astronomy powerful telescopes are demanded. It is true that recent advances in our science have been due in large part to the possession of powerful and expensive equipment. But the directors of observatories possessing such equipment are wisely restricting their programmes of work to those problems which cannot be solved equally well with small instruments; and it would be a grievous mistake to assume that the small telescope in suitable hands is not able to render good account of itself.
Eins og áður sagði réði Leuschner Sturlu sem aðstoðarmann við stöðina strax eftir komuna þangað árið 1905. Við það fjölgaði starfsliðinu úr tveimur í þrjá, því 1903 hafði R.T. Crawford (1876-1958) verið ráðinn kennari við stjörnufræðideildina. Starfsmannafjöldinn óx síðan hægt og sígandi á næstu áratugum. Hvað Sturlu varðar má geta þess að hann hlaut framgang í starfsheitið fyrirlesari (instructor/lecturer) árið 1910, varð doktor (Ph.D.) 1913, lector (assistant professor) 1918, dósent (associate professor) 1920 og loks fullgildur prófessor árið 1928. Hann vann síðan samfellt við stjörnufræðideildina í Berkeley til starfsloka árið 1950.
Armin O. Leuschner
Leuschner, leiðbeinandi Sturlu í doktorsnámi, var einn þekktasti stjörnufræðingur síns tíma í Vesturheimi. Hann lagði grunnin að rannsóknum við stjörnufræðideildina í Berkeley og tókst að gera hana að bestu deild á því sviði í Bandaríkjunum. Það gerði hann meðal annars með því að koma á náinni samvinnu við Lick stjörnuathugunarstöðina á Hamiltonfjalli. Einnig má geta þess, að hann átti mikinn þátt í að leggja grunn að rannsóknum í eðlisfræði í Berkeley í byrjun tuttugustu aldar, líkt og stjarneðlisfræðingurinn G.E. Hale gerði við Throop verkfræðiskólann í Pasadena, sem síðar varð að Tækniháskóla Kaliforníu (Caltech).
Þótt Leuschner hafi komið víða við í rannsóknum sínum var sérsvið hans aflfræði himintungla, einkum kennilegar rannsóknir á brautum smáhnatta (halastjarna og smástirna) í sólkerfinu. Brautir þessara hnatta verða yfirleitt fyrir talsverðum þyngdartruflunum frá nálægum reikistjörnum (all ítarlega umfjöllum um þetta efni er að finna í færslunni um stjörnufræðingurinn Steinþór Sigurðsson [1904-1947]).
Þekktastur er Leuschner fyrir sérstaka aðferð (Leuschner’s Short Method) til að ákvarða grunnstærðir (orbital elements) fyrir svokallaða snertibraut (osculating orbit) hnattar, það er að segja bestu Keplersbrautina, sem fellur að niðurstöðum mælinga á stöðu hnattarins á þremur mismunandi tímum. Aðferð hans var veruleg einföldun á nálgunaraðferð, sem P.-S. Laplace (1749-1827) hafði sett fram árið 1799.
Á fyrstu áratugunum eftir að Leuschner kynnti þessa nýju aðferð var hún mikið notuð af bandarískum stjörnufræðingum, ekki síst í Kaliforníu. Sem dæmi má nefna að Sturla Einarsson notaði hana í svo til öllum sínum brautarreikningum (nánar um það hér á eftir).
Bestu og skipulegustu umfjöllunin um aðferð Leuschners er þó ekki að finna í ofantöldum greinum, heldur í eftirfarandi kennslubók:
Lick athugunarstöðin
Ein helsta ástæða þess að framhaldsnámið við stjörnufræðideildina í Berkeley var talið jafn gott og raun ber vitni voru náin tengsl deildarinnar við Lick stjörnuathugunarstöðina. Hún var fyrsta stöðin í heiminum sem reist var á háum fjallstindi og á sínum tíma voru athugunarskilyrði þar einstaklega góð. Stöðin var og búin stórum og vönduðum sjónaukum, sem ætlaðir voru til grunnrannsókna á himingeimnum.
Lick stjörnuathugunarstöðin á Hamiltonfjalli í Kaliforníu árið 1900. Hún er nefnd eftir athafnamanninum J. Lick (1796-1876) sem hvílir undir stóra linsusjónaukanum í stærstu hvelfingunni. Myndin er fengin að láni hjá Kubitz, A., 2013: The Lick Observatory on Mount Hamilton: Historic Outpost of Science! Sjá einnig vefsíðurnar Historical Resources, Historical Telescopes og Historical Collections.
Þótt Lick-stöðin væri innan vébanda Kaliforníuháskóla var hún formlega sjálfstæð stofnun með eigin stjórn og starfslið. Samvinnan við stjörnufræðideildina í Berkeley hófst árið 1898 með samkomulagi milli Leuschners og þáverandi forstöðumanns Lick-stöðvarinnar, hins merka stjarnvísindamanns J.E. Keelers, þess efnis að vísindamenn stöðvarinnar kæmu reglulega í heimsókn til Berkeley og héldu þar fyrirlestra um nýjungar í rannsóknum. Að auki var ákveðið að bæði framhaldsnemar og kennarar frá Berkeley gætu dvalið um lengri eða skemmri tíma við stöðina við rannsóknir. Þetta gerði það meðal annars að verkum að á fyrstu áratugum tuttugustu aldar útskrifaði stjörnufræðideildin í Berkeley fjölda framúrskarandi doktora, sem margir áttu eftir að gegna mikilvægu hlutverki við uppbyggingu stjarnvísinda víðsvegar um Bandaríkin.
Á dögum Sturlu voru stærstu og þekktustu sjónaukar Lick-stöðvarinnar tveir, báðir þrjátíu og sex þumlungar (91 cm) að þvermáli og pólstilltir. Þeir voru hinn 17 metra langi Lick linsusjónauki frá 1888 og Crossley spegilsjónaukinn sem settur var upp árið 1905.
Lick linsusjónaukinn eins og hann leit út árið 1910 (sjá einnig hér). Teikning: Wikipedia.
Árið 1909 dvaldi Sturla ásamt samstúdent sínum R.K. Young (1886-1973) um tíma við athugunarstöðina á Hamiltonfjalli við rannsóknir á sjónlínuhraða fjarlægra sólstjarna með svokallaðri Mills litsjá, sem tengd var við Lick linsusjónaukann. Þar lærðu þeir einning að túlka önnur litróf undir stjórn þáverandi forstöðumanns Lick-stöðvarinnar W.W. Campbells og litrófsfræðingsins K. Burns (1881-1958). Ég veit ekki til að sjónlínuhraða-mælingarnar hafi verið birtar.
Mynd af Mills litsjánni frá 1898. Þarna er hún tengd við stóra Lick linsusjónaukann. Gripurinn er nefndur eftir D.O. Mills, hollvini athugunarstöðvarinnar. Mynd: Wikipedia.
Vormisserið 1929 tók Sturla, þá nýorðinn prófessor, sér leyfi frá kennslu og fluttist tímabundið upp á Hamiltonfjall með konu sína og börn (fjallað verður um fjölskylduna hér á eftir). Frá þeim tíma liggur eftir hann grein um mælingar á stöðu halastjörnunnar 1929a Schwassmann-Wachman með Crossley spegilsjónaukanum. Að auki er getið um athuganir hans í grein eftir A.S. Young.
Crossley spegilsjónaukinn eins og hann leit út árið 1905. Hann er kenndur við enska athafnamanninn E. Crossley (1845-1905) sem gaf stöðinni sjónaukann (sjá einnig hér og hér). Mynd: Lick Observatory.
Í lok þessa kafla er við hæfi að nefna að nú um stundir eru umsvif Kaliforníuháskóla á sviði stjarnvísinda heldur viðameiri en þau voru á dögum Sturlu Einarssonar.
Innan sviga: Eini Íslendingurinn, annar en Sturla, sem ég veit til að hafi tengst Lick athugunarstöðinni með einhverjum hætti er stjarneðlisfræðingurinn Steinn Sigurðsson (f. 1965) sem nú er prófessor við Ríkisháskólann í Pennsylvaníu. Hann mun hafa verið nýdoktor á Lick frá 1991 til 1994. Hér má finna viðtal við Stein frá 1995.
Kennilegi stjarneðlisfræðingurinn Steinn Sigurðsson. Myndin er fengin að láni úr fréttinni Sigurdsson elected as fellow of the American Physical Society frá 2021.
Rannsóknarnámið í Berkeley 1905-1913
Svo heppilega vill til að á doktorsskírteini Sturlu Einarssonar frá 1913 eru talin upp öll þau námskeið sem hann fékk viðurkennd í rannsóknarnáminu:
Af listanum má sjá að mikil áhersla hefur verið lögð á aflfræði himintungla í náminu, einkum þó brautarreikninga, enda var það helsta sérsvið Leuschners. (Eins og áður var bent á er all ítarlega umfjöllun um þessi fræði að finna í færslu um Steinþór Sigurðsson).
Á námsárum Sturlu og síðar voru helstu grundvallarritin á þessu sviði þýsku doðrantarnir,
Þegar fram liðu stundir var þó nær eingöngu stuðst við verk bandarískra höfunda í kennslunni í Berkeley, eins og til dæmis:
Hér má jafnframt lesa fróðlegar greinar um þær flækjur sem komið gátu upp í brautarreikningum á þessum tíma:
Sjá einnig grein danska stjörnufræðingsins J.V. Hansens (annars af aðalkennurum Steinþórs Sigurðssonar í Kaupmannahöfn) sem hún skrifaði meðan hún stundaði rannsóknir við Lickstöðina á dögum seinni heimsstyrjaldarinnar:
Á námsárum Sturlu var fjöldi framhaldsnema í stjörnufræði þegar orðinn óvenju mikill, miðað við það sem áður var. Þar á meðal voru nokkrar konur.
Mynd af sex stjörnufræðistúdentum í Berkeley árið 1908. Efri röð frá vinstri: Sturla Einarsson, E.A. Fath (1880-1959) og W.F. Meyer (1880-1948). Í fremri röðinni standa þær A.E. Glancy (1883-1975), S. Morgan og A. Joy. Myndin er úr greininni Anna Estelle Glancy (1883-1975). Sjá einnig í þessu sambandi grein S.M. Humphreys frá 2021: Celestial Observers: First Sixteen Berkeley Women Doctoral Graduates in Astronomy 1913-1952.
Ljósmynd Glancys af halastjörnunni c 1908 Morehouse, tekin frá Lick athugunarstöðinni 14. nóvember 1908 (sjá t.d. Glancy, A.E., 1908: Photographs of Comet C 1908 (Morehouse)). Niðri í Berkeley reiknuðu þeir Sturla og Meyer hins vegar bæði brautarstika snertibrauta og stutt stjörnualmanak fyrir stjörnuna (Einarsson, S & Meyer, W.F., 1908: Second elements and ephemeris of Comet C 1908 (Morehouse)).
Stjörnufræðideildin í Berkeley var ekki aðeins önnum kafin við kennslu og rannsóknir, heldur sinnti hún einnig alþýðufræðslu. Það má til dæmis sjá af eftirfarandi frétt úr blaðinu San Francisco Call, hinn 24. ágúst 1907:
Opinbert boð til almennings um að skoða halastjörnuna Daniel D 1907 í gegnum sjónauka Berkeley-stöðvarinnar. Stúdentarnir sem minnst er á í fréttinni eru þau Sturla Einarsson og A.E. Glancy. Sjá Crawford, R.T., Einarsson, S. & Glancy, A.E., 1907: Elements and ephemeris of Comet D 1907 (Daniel). Einnig eftirfarandi greinar eftir J.C. Duncan (1882-1967): Duncan, J.C., 1907a: Note on Comet d 1907 (Daniel)) og Duncan, J.C., 1907b: Photographic observations of Comet D 1907 (Daniel) & Polariscopic observations of Comet D 1907 (Daniel).
Eins og áður hefur komið fram var Sturla ráðinn aðstoðarmaður við athugunarstöðina strax og hann hóf framhaldsnám árið 1905. Eftir fimm ár hóf hann að kenna námskeið í nytjastjörnufræði (Practical Astronomy), við stjörnufræðideildina og fékk þá starfsheitið fyrirlesari (instructor/lecturer) sem hann hélt til 1918. Þetta hefur sennilega valdið því að það tók hann átta ár að ljúka doktorsprófi (1913). Þremur árum eftir prófið varð hann lektor (assistant professor), síðan dósent (associate professor) 1920 og loks prófessor 1928.
Kennilega hluta námsins hjá Sturlu hefur þegar verið lýst, en „verklegi hlutinn“ í námsefni hans og samstúdenta var aðalega í því fólginn að nota nýlegar athuganir annarra stjörnufræðinga á halastjörnum (og stundum smástirnum) til að reikna út staðsetningu þeirra á himinkúlunni nokkrar vikur eða mánuði fram í tímann (þ.e. reikna stutt stjörnualmanak með gildum á stjörnulengd (α), stjörnubreidd (δ) og eiginhreyfingu (μ) halastjarnanna). Þessu fylgdu ávallt útreikningar á tilheyrandi snertibrautum. Sjá nánari umfjöllun um slíka vinnu í færslunni um Steinþór Sigurðsson.
Í ritaskrá Sturlu má sjá að á tímabilinu 1905-1913 hefur hann, auk doktorsritgerðarinnar, birt (oftast ásamt öðrum) einar tuttugu og þrjár vísindagreinar. Á árunum 1914-1928 voru þær sjö og eftir að hann varð prófessor 1928 aðeins ein. Ástæðan mun vera sú að eftir 1920 tóku kennsla og stjórnunarstörf svo til allan hans tíma.
Akkilles, fyrsta smástirnið á braut Júpíters, fannst í ársbyrjun 1906, þegar Sturla var á öðru misseri í framhaldsnáminu í Berkeley. Því sem næst samstundis stakk sænski stjörnufræðingurinn C.V.L. Charlier (1862-1934) upp á því, að smástirnið væri bundið við Lagrangepunktinn L4 á braut Júpíters og því væri æskilegt að kanna það svæði nánar og einnig svæðið í kringum L5, og jafnvel tilsvarandi svæði á braut Satúrnusar. Um haustið fannst svo Patróklos við L5, síðan Hektor við L4 í ársbyrjun 1907 og loks Nestor við L4 vorið 1908. Fljótlega var farið að kenna þessi smástirni við Tróju, en hið fimmta, Príamos, fannst þó ekki fyrr en 1917 og þá við L5. Í dag skiptir fjöldi þekkra Trójusmástirna þúsundum.
Einfaldar skýringarmyndir af stöðu helstu smástirnahópa í sólkerfinu. Efri myndin sýnir bæði smástirnabeltið milli Mars og Júpíters og Trójusmástirnin á braut Júpíters. Sú neðri sýnir tveggja hnatta kerfið Sól-Júpíter og hina fimm Lagrange punkta þess, auk Trójusmástirnanna. Þríhyrningarnir sem tengja stöðugu jafnvægispunktana L4 og L5 við Júpíter og sólina eru jafnhliða. Bæði Akkilles og Hektor eru á yfirráðasvæði punktsins L4. Sjá ítarlega umfjöllun um þetta efni í færslunni um stjörnufræðinginn Steinþór Sigurðsson. Myndirnar eru af veraldarvefnum.
Uppgötvun Trójusmástirnanna vaki strax mikla athygli í Evrópu og sums staðar í Bandaríkjunum, en stjörnufræðingarnir í Berkeley virðast lítið hafa sinnt þeim, allt þar til Sturla hóf að vinna að doktorsritgerð sinni, sem hann svo varði í byrjun maí 1913:
Í ritgerðinni notar hann áðurnefnda aðferð Leuschners til að reikna út brautir Hektors og Akkillesar um sólina. Með samanburði við útreikninga dansk/sænska stjörnufræðingsins S.E. Strömgrens (1870-1947) á braut Hektors á árunum 1908-1912 fullyrðir Sturla að niðurstöður sínar, fengnar með aðferð Leuschners, gefi mun nákvæmari lýsingu á braut Hektors en sú reikniaðferð, sem Strömgrein notaði. Því til sönnunar vísar hann á mælingar á stöðu Hektors á hvelfingunni.
Fyrir utan tilvitnanir í Leuschner og Strömgren getur Sturla aðeins um einn stjörnufræðing til viðbótar í ritgerðinni. Það er bandarísk/enski vísindamaðurinn E.W. Brown (1866-1938), prófessor við Yaleháskóla, sem skrifaði nokkrar athyglisverðar greinar um smástirnabrautir árið 1911.
Mér vitanlega var doktorsritgerð Sturlu aldrei gefin út prentuð, en Leuschner minnist á niðurstöður hans í yfirlitsgrein frá 1922, Celestial mechanics: A survey of the status of the determinationof the general perturbations of the minor planets (sjá bls. 9-10, 54 og 61). Þá er vísað í PASP-grein Sturlu frá 1913 í ensku Wikipediugreininni um Trójusmástirnin (tilvitnun nr. 13).
Listi yfir doktorsritgerðir í raunvísindum frá Berkeley árið 1913. Þær eru allar í stjörnufræði. Úr Science-greininni Doctorates Conferred by American Universities, bls. 266. Auk Sturlu og A.E. Glancy eru á listanum þau E.S Haynes (1880-1956), sem síðar varð prófessor við Háskólann í Missouri, C.C. Kiess (1887-1967), P.W. Merrill (1887-1961) og E.P. Waterman (1882-1967). Karlarnir fjórir fengu fljótlega stöður við hæfi í Bandaríkjunum, en konurnar tvær ekki. Þær brugðu því á það ráð að halda áfram rannsóknum í Argentínu, eins og lesa má um í grein S. Paolantonio frá 2018: Two Women Astronomers at the Argentine National Observatory: Dr. Anna Estelle Glancy and Dr. Emma Phoebe Waterman. Báðar sneru þær þó aftur til Bandaríkjanna. Sjá í því sambandi greinarnar The First Lady of Optics og More Than a Century Ago, Astronomer Phoebe Waterman Defied Her Doubters.
Innan sviga: Eins og þegar hefur komið fram fylgdist S.E. Strömgren, prófessor í stjörnufræði við Kaupmannahafnarháskóla og forstöðumaður Stjörnuathugunarstöðvar-innar á Østervold, grannt með ferðum Hektors um árabil. Nemandi hans og síðar stjörnumeistari Østervoldsstöðvarinnar, J. Vinter Hansen (1890-1960), gerði hið sama hvað Akkilles varðar. Þessi áhugi á Trójusmásirnum Júpíters varð til þess að nemandi þeirra, Steinþór Sigurðsson (1904-1947), valdi útreikninga á braut Akkillesar sem magistersverkefni við Kaupmannahafnarháskóla. Verkefnið varði hann 1929 og gaf ritgerðina síðan út endurbætta í Astronomische Nachrichten árið 1933 (sjá nánar í færslunni stjörnufræðingurinn Steinþór Sigurðsson):
Frá vinstri: S.E. Strömgren prófessor, J. Vinter Hansen stjörnumeistari og Steinþór Sigurðsson stjörnufræðingur.
Þótt Sturla hafi stundað rannsóknir af kappi á námsárunum, dró smám saman úr þeim með tímanum og eftir að hann varð fastráðinn prófessor, árið 1928, virðist hann að mestu hafa hætt slíkri iðju og snúið sér alfarið að kennslu og stjórnunarstörfum. Meginverkefni hans sem kennara við stjörnufræðideildina í Berkeley var að kenna verðandi stjörnufræðingum, byggingaverkfræðinemum og öðrum sem áhuga höfðu svokallaða nytjastjörnufræði (Practical Astronomy). Í þeirri grein var einkum fjallað um himinkúlufræði, ákvörðun tímans, staðarákvarðanir og landmælingar, auk siglingafræði og notkun tilheyrandi mælitækja. Einnig kom hann, eins og aðrir kennarar deildarinnar, að kennslu grunnnámskeiðs deildarinnar í almennri stjörnufræði. Þá kenndi hann bandarískum sjóliðsforingjaefnum siglingafræði í báðum heimsstyrjöldum við miklar vinsældir. Hann þótti einstaklega góður kennari og að sögn samtímamanna lét hann sér mjög annt um nemendur sína. Færsluhöfundi hefur ekki enn tekist að grafa upp hvaða bækur Sturla notaði við kennsluna, en eftirfarandi kennslubækur gefa góða lýsingu á því sem féll undir hatt nytjastjörnufræði á þeim tíma:
Ég hef ekki fundið neinar myndir af Sturlu við kennslu, en hér eru í staðinn tvær hópmyndir, þar sem sjá má hann í mannþröng (fylgið rauðu örvunum):
Þátttakendur á þingi Stjarnvísindafélags Bandaríkjanna (AAS) í Berkeley í ágúst 1915. Í fremstu röð sitja frá vinstri: Óþekktur, S.D. Townley (1867-1946), R.T. Cawford (1876-1958), H.D. Curtis (1872-1942), óþekktur og óþekktur. Í annarri röð, sitjandi á stólum, eru frá vinstri: R.G. Aitken (1864-1951), R.H. Tucker (1859-1952), E.A. Fath (1880-1959), E.B. Campbell (eiginkona W.W. Campbells), F. Schlesinger (1871-1943), W.W. Campbell (1862-1938), G.E. Hale (1868-1938) og A.O. Leuschner. Á enda raðarinnar, sitjandi á jörðinni, er J. Stebbins (1878-1966; sköllóttur). Fyrir aftan hinn sitjandi hóp stendur H. Shapley (1885-1972), sjötti frá vinstri. Hinum megin stendur Sturla Einarsson, áttundi frá hægri (örin bendir á hann). Mynd: Meetings of the AAS: 1908-1915.
Starfsmenn stjörnufræðideildarinnar í Berkeley fyrir utan Stjörnuathugunarstöð stúdenta (The Students' Observatory) í Berkeley vorið 1923. Í öftustu röð standa frá vinstri: C.D. Shane (1895-1983), H.B. Kaster, R.T. Crawford (1876-1958), E.W. Brown (1866-1938; gestur), A.O. Leuschner, R.H. Sciobereti (1886-1974) og V.F. Lenzen (1890-1975). Sitjandi í miðröðinni eru frá vinstri: H. Thiele (1878-1946), A. Williams (1884-1961), E. Wilkinson, óþekkt, K. Prescott (1901-1980), M.L.H. Shane (1897-1977), Sturla Einarsson (örin bendir á hann), J. Pearce (1893-1988), W.F. Meyer og Th. Buck (1881-1969). Í fremstu röðinni sitja frá vinstri: Óþekktur, J.D. Shea, B.C. Wong (1890-1947) and J.F. Pobanz. Mynd: Mary Lea Shane Archives of the Lick Observatory.
Stjörnufræðingurinn L.H. Aller (1913-2003) var B.A. nemi í stjörnufræði í Berkeley á fjórða áratugi tuttugustu aldar. Í sjálfsævisöguágripinu An Asronomical Rescue, sem birt var 1995, segir hann meðal annars eftirfarandi um dvölina þar (bls. 4):
I [...] found myself in an astronomical department steeped in 19th-century traditions. Although William F Meyer taught a course on practical aspects of astronomical spectroscopy, C Donald Shane presented modern ideas on such topics as stellar atmospheres, and later Robert J Trumpler covered topics in statistical astronomy and galactic structure, the big push was on orbit theory. Celestial mechanics and orbit theory have an elegance that was almost totally obscured by the tedious massaging of equations to render them suitable for logarithmic calculations. Mechanical calculating machines had appeared but were totally despised by people like Russell Tracy Crawford. The department was severely ingrown. Until Trumpler arrived there was nobody who had not come up through the ranks of the Berkeley Astronomical Department. Although Leuschner was a terrible lecturer, he was also one of the most inspiring teachers I've ever had. He would suggest new ideas and approaches in such a way as to fire the enthusiasm of even this dyed-in the-wool spectroscopist. We students came to regard the astronomy department as a kind of family, in which Leuschner was the patriarch, Sturla Einarsson was the kindly old prof on whose shoulder you could cry, and Donald Shane was the Dutch uncle who saw that we were always aware of the hard realities of life.
Eins og þarna segir, var nepótismi lengi allsráðandi við stjörnufræðideildina og á dögum Leuschners fengu nær eingöngu fyrrum nemendur hans fastráðningu þar. Áðurnefndur R.J. Trumpler (1886-1956) var eina undantekningin, enda hafði hann starfað við Lick-stöðina frá 1918 og kenndi oft nemendum við Berkeley. Þetta breyttist fyrst meðan Sturla var forseti stjörnufræðideildarinnar á árunum 1946-1950. Á þeim tíma tókst honum að lokka tvo framúrskarandi stjarneðlisfræðinga fra Yerkes athugunarstöðinni til Berkeley, fyrst L.G. Henyey (1910-1970) árið 1947 og síðan O. Struve (1897-1963) árið 1950. Um þetta segir D.E. Osterbrock í tölvubréfi til undirritaðs, 15. desember 1993:
Einarsson was only in this position [chairman] a few years, but perhaps his most important contribution was that he recruted Otto Struwe, then director of Yerkes, to come to Berkeley to succeed him when he retired (in 1950). This was the first step (actually the second, the first was Einarsson’s hiring Louis G. Henyey as the first astrophysicist on the Berkeley faculty in 1947) in changing Berkeley over from its rather old-fashioned ways under Leuschner (and Crawford) to the very strong, astrophysics-oriented department it is today.
Stjarneðlisfræðingarnir L.G.Heyney til vinstri og O. Struve.
Sturla tók að sér mörg önnur stjórnunarstörf, bæði utan skóla sem innan. Meðal annars var hann formaður (president) hins þekkta félags Astronomical Society of the Pacific (ASP) árið 1934 (tók við af E. Hubble) og starfaði árum saman (1950-1968) sem ritari-gjaldkeri (secretary-treasurer) félagsins. Til gamans má finna hér uppgjör hans fyrir árið 1953.
Eins og sagði í upphafi voru foreldrar Sturlu bóndinn og síðar kirkjugarðsvörðurinn Jóhann Einarsson (1853-1917) og kona hans Elín Benónísdóttir (1850-1930). Systkini hans voru Nanna (1884-1969) og Baldur (1886-1962).
Sumarið 1914 gekk Sturla að eiga fyrri konu sína, Anna Rodman Kidder (1890-1940). Í nokkur ár þar á undan hafði hún verið framhaldanemi við stjörnufræðideildina og aðstoðarmaður við athugunarstöðina, líkt og Sturla hafði áður verið. Eins og þá tíðkaðist hætti hún námi við giftinguna, en náði þó að ljúka meistaraprófi í stjörnufræði árið 1913 með ritgerðinni A Comparison of Photographic with Theoretical Positions of Six Minor Planets.
Til gamans set ég hér inn skemmtilega frétt af stjörnufræðingunum við Berkeley frá 1913, þar sem minnst er á þau bæði, Önnu og Sturlu:
Úr San Jose Mercury-News, 6. okt. 1913: Comet lost for half a century seen again. Hér má svo lesa nánar um halastjörnurnar 20D/Westphal, 29P/Neujmin og B 1913 Metcalf.
Þau Anna og Sturla eignuðust fjögur börn: Alfred Worchester (1915-2009) eðlisfræðing, Elizabeth (Cook) (f. 1917), Margaret (Dechant) (f. 1920) og John Rodman (f. 1921) verkfræðing. Anna lést árið 1940, þá aðeins fimmtug, en Sturla lifði mun lengur. Hann var orðinn 94 ára gamall þegar hann dó 1974. Þá voru barnabörnin orðin sex og eitt barnabarnabarn komið í heiminn.
Sturla giftist seinni konu sinni, Thea C. Hustvet (f. 1902), árið 1945. Hún mun hafa verið viðskiptaskólagengin og leiddi það meðal annars til þess að hún aðstoðaði mann sinn í störfum hans fyrir ASP. Eftir að hann hætti sem gjaldkeri árið 1968, varð hún aðstoðar-ritari-gjaldkeri félagsins í nokkur ár.
Sturla ásamt seinni eiginkonu sinni Thea Einarsson (fædd Hustvet) árið 1966. Mynd: Mary Lea Shane Archives of the Lick Observatory.
Að sögn samferðamanna lifði Sturla ávallt hamingjusömu fjölskyldulífi og lagði mikla áherslu á að sinna henni vel.
Eftir að seinni heimsstyrjöldin skall á lokaðist fljótlega fyrir aðgang íslenskra stúdenta að háskólum í Evrópu. Margir brugðu þá á það ráð að fara til Bandaríkjanna til háskólanáms, meðal annars við Kaliforníuháskóla í Berkeley. Hópur Íslendinga þar var orðinn svo stór haustið 1942 að ákveðið var að stofna sérstakt félag, Félag íslenzkra stúdenta í Berkeley, „til að halda hópinn og ræða sameiginleg áhugmál [auk þess að] kynna Ísland og Íslendinga meðal þeirra íbúa Vesturheims, sem hafa áhuga á íslenzkum málum.“ Verndarar félagsins voru séra Steingrímur O. Thorláksson og frú og heiðursfélagar þau Sigríður Benónýs og Sturla Einarsson. Um Sturlu segir í fréttinni:
Heiðursfélaga hafa stúdentarnir kjörið prófessor Sturlu Einarsson [...] sem kennir stjörnu- og siglingafræði. [Hann] er eini íslenzki prófessorinn við University of California og hefir jafnan greitt götu íslenzku stúdentanna og reynst þeim hinn bezti vinur.
Þessi mynd af Sturlu birtist í greininni Meðal íslenzkra námsmanna í Kaliforníu í Vísi, 16. apríl 1944, bls. 1. Sennilega stendur hann þarna við einn af sjónaukunum í Stjörnuathugunarstöð stúdenta. Í sömu grein (bls. 4) má einnig sjá eftirfarandi mynd:
Sturla ræðir við nokkra íslenska stúdenta í Berkeley árið 1944. Næst honum situr Bjarni Jónsson stærðfræðingur (1920-2016). Bjarni var þarna á fyrsta ári í doktorsnámi í stærðfræði við skólann, eftir að hafa lokið þar B.A. prófi árið áður.
Haustið 1998 skrifaði Halldór Þorsteinsson bókavörður og skólastjóri ágæta opnugrein í Lesbók Morgunblaðsins um dvöl sína í Berkeley á sríðsárunum: Námstími í Berkeley. Um Sturlu lætur hann þessi orð falla:
Auk okkar íslensku háskólastúdentanna starfaði vestur-íslenskur prófessor í stjörnufræði við Kaliforníuháskóla, Sturla Einarsson, sem reyndist okkur hollur ráðgjafi og góður vinur.
Af því sem þegar hefur verið tínt til má ráða að Sturla Einarsson hefur verið í miklum metum, ekki aðeins meðal íslenskra stúdenta í Berkeley á dögum seinni heimsstyrjaldar-innar, heldur einnig meðal allra þeirra nemenda, sem voru svo lánsamir að njóta kennslu hans á fyrri hluta tuttugustu aldar.
Þar sem ég þekkti Sturlu ekki persónulega og hitti hann aldrei, er vel við hæfi að ljúka þessari umfjöllun með ummælum nokkurra samstarfsmanna hans í Berkeley um líf hans og störf:
Í minningargrein frá 1975 segir prófessor J.G. Phillips eftirfarandi (bls. 23):
While Sturla Einarsson made significant research contributions on the orbits of comets and asteroids, he will be remembered by generations of Berkeley students as a dedicated teacher. His personal interest in their welfare and careers was legendary. During his entire career at Berkeley he was immersed in University affairs, serving on innumerable faculty and university commities and boards during the Sproul heyday when the Univerity of California was undergoing its explosive growth.
Nítján árum síðar skrifar Phillips svo í bréfi til undirritaðs (23. janúar 1994):
[Sturla Einarsson] was of the old school, with a courtly manner not frequently found among astronomers today. Along with Cunningham he was the last of the Berkeley group made famous by Schlessinger [probably Phillips ment to write Leuschner?] for their expertise in positional astronomy and calculation of orbital elements; fields thought by us of the younger generation to be “hopelessly out of date”. Little did we know that American astronomy would soon be greatly embarassed when satellites started orbiting the Earth and so few of us had bothered with orbits. Yet despite this gulf between our research interests, Sturla Einarsson was always extremely solicitous of the welfare of us younger astronomers, helping out when he could. When he retired he insisted on giving up his office, and instead set up a desk in a very draughty transit house. […] I will always cherish the memory of Einarsson, as an example of a breed that has, unfortunately, become very rare.
Að lokum birti ég hér umsögn prófessoranna H.F. Weavers, L.E. Cunninghams og C.D. Shanes um Sturlu í minningargrein frá 1980 (bls. 80):
The quiet, solid success of Einarsson's long and productive life stemmed from ability, perseverance, and the boldness to seize opportunity when presented, combined with humor, honesty, and a strong sense of community. Parts of his biography read like incidents in a novel set in an earlier, simpler America that was vigorous, interesting, and full of opportunity. Many look at such a time with considerable nostalgia.
Sturla Einarsson á efri árum. Mynd úr minningargrein J.G. Phillips frá 1975.
Ritaskrá Sturlu má finna hér.
* Stjarneðlisfræði og heimsfræði á Íslandi: Efnisyfirlit *
Eftirfarandi skrá fylgir færslu 3c1 um stjörnufræðinginn Sturlu Einarsson.
Sturla Einarsson stjörnufræðingur (1879-1974) árið 1946, þá 67 ára gamall. Teikning eftir P. Van Valkenburgh.
* Stjarneðlisfræði og heimsfræði á Íslandi: Efnisyfirlit *
Ef við undanskiljum Vestur-Íslendinginn Sturlu Einarsson (1879-1974; Ph.D. frá Berkeley 1915), sem fluttist fjögurra ára gamall með foreldrum sínum til Vesturheims árið 1883, voru fyrstu Íslendingarnir, sem luku formlegu háskólaprófi með stjörnufræði sem aðalgrein þeir Steinþór Sigurðsson (1904-1947; mag. scient. frá Kaupmannahafnarháskóla 1929) og Trausti Einarsson (1907-1984; dr. phil. frá Háskólanum í Göttingen 1934). Eftir heimkomuna sneru þeir sér báðir að öðrum viðfangsefnum en stjörnufræði, enda voru engir innviðir þá til á Íslandi, sem gerðu þeim kleift að leggja stund á fræðigrein sína.
Þess ber að geta, að allir þeir sem útskrifuðust með lokapróf í stærðfræði, eðlisfræði eða efnafræði frá Kaupmannahafnarháskóla á fyrri helmingi tuttugustu aldar voru einnig vel menntaðir í grunnatriðum stjörnufræðinnar og gátu því kennt hana í íslenskum menntaskólum. Í þeim hópi voru meðal annarra þeir Þorkell Þorkelsson eðlisfræðingur (1876-1961), Ólafur Dan Daníelsson stærðfræðingur (1877-1957), Sigurkarl Stefánsson stærðfræðingur (1902-1995), Guðmundur Arnlaugsson stærðfræðingur (1913-1996) og Björn Bjarnason stærðfræðingur (1919-1999).
Það var ekki fyrr en Þorsteinn Sæmundsson (1935-2023; Ph.D. frá Lúndúnaháskóla 1962) hóf störf við Eðlisfræðistofnun Háskólans árið 1963, sem fyrsti vísirinn að rannsóknum á afmörkuðu sviði nútíma stjarnvísinda skaut rótum hér á landi. Er þar átt við fræðin um samband sólar og jarðar.
Í því, sem hér fer á eftir, er ætlunin að fjalla nánar um stjörnufræðinginn Steinþór Sigurðsson og það umhverfi náms og fræða, sem hann hrærðist í á Kaupmannahafnar-árunum 1923 til 1929.
Steinþór Sigurðsson stjörnufræðingur.
Áður en hann fór til Kaupmannahafnar stundaði Steinþór nám við Menntaskólann í Reykjavík, þar sem hann mun hafa skarað fram úr í raunvísindum, einkum þó eðlisfræði. Lærimeistararnir voru heldur ekki af verri endanum: Ólafur Dan Daníelsson kenndi honum stærðfræði og stjörnufræði og Þorkell Þorkelsson eðlisfræði.
Hugur Steinþórs hefur snemma hneigst að stjörnufræði, eins og sjá má á ummælum sem Jón Eyþórsson veðurfræðingur hefur eftir Hákoni Bjarnasyni skógræktarstjóra í grein sinni um Steinþór í Andvara árið 1954 (bls. 8):
Á fyrstu árum sínum í menntaskóla smíðaði Steinþór stjörnukíki í félagi við Magnús Magnússon, nú símaverkfræðing. Þeir slípuðu sjálfir holspegil í hann. Yfirleitt þekkti Steinþór allar mögulegar stjörnur á himninum, frá því ég man eftir, og reyndi að kenna okkur margt í stjörnufræði.
Einn helsti vinur Steinþórs á skólaárunum var Sigurkarl Stefánsson, síðar stærðfræðingur og samkennari hans við Menntaskólann í Reykjavík og Háskóla Íslands. Þeir urðu stútentar frá stærðfræðideild vorið 1923 og héldu báðir til Kaupmannahafnar að því loknu til frekara náms. Þá var Steinþór aðeins nítján ára gamall.
Stúdentar frá MR vorið 1923. Í hópnum er þriðji árgangurinn, sem útskrifaðist frá stærðfræðideild MR. Þarna má sjá marga þekkta Íslendinga, m.a. Steinþór Sigurðsson (þriðji frá hægri í næst öftustu röð) og vini hans, Magnús Magnússon, síðar verkfræðing (annar frá hægri í sömu röð og Steinþór) og Sigurkarl Stefánsson, síðar stærðfræðing (fjórði frá vinstri í öftustu röð). Ljósmynd: Sigríður Zoëga.
Eins og áður kom fram, verður hér fyrst og fremst fjallað um stjörnufræðinám Steinþórs og það vísindalega umhverfi, sem hann hrærðist í við Háskólann í Kaupmannahöfn á árunum 1923 til 1929. Í næstu færslu (4a2) verður svo rætt lauslega um störf hans á Íslandi að loknu háskólaprófi. Einnig má lesa um helstu þættina í ævi og störfum Steinþórs eftir heimkomuna í ritum eins og Verkfræðingatali og Kennaratali og ekki síst í minningargreinunum, sem tengt er á hér að neðan. Þá má nálgast frekari upplýsingar um prentuð verk Steinþórs í meðfylgjandi ritaskrá.
Steinþór fór til náms í Kaupmannahöfn sumarið 1923 og Sigurkarl fylgdi í kjölfarið nokkrum mánuðum seinna. Fyrstu tvö til þrjú árin sóttu þeir sömu fyrirlestrana, en á seinni hluta skildu leiðir, þegar Sigurkarl fór að lesa undir kandídatspróf í stærðfræði.
Steinþór ætlaði í fyrstu að leggja fyrir sig eðlisfræði, en það breytist smám saman vegna vaxandi áhuga hans á stjörnufræði. Hann lauk því magistersprófi (mag.scient.) í þeirri grein 1929, rúmu ári eftir að Sigurkarl hlaut sína kandídatsgráðu (cand.scient).
Ein besta heimildin, sem ég hef fundið um stjörnufræðinámið í Kaupmannahöfn á þessum tíma, er viðtal sem þau hjónin L. Hoddeson vísindasagnfræðingur og G. Baym eðlisfræðingur áttu við hinn þekkta stjarneðlisfræðing, Bengt Strömgren (1908-1987) árið 1976. Hann var sonur aðalkennara Steinþórs, prófessors Elis Strömgren (1870-1947), og þeir Steinþór og Bengt þekktust vel, þar sem þeir voru samtímis við nám í stjörnufræði við Háskólann. Í viðtalinu segir Bengt meðal annars:
[The job opportunities in astronomy in Denmark at that time were] very limited. Actually, the philosophy was that those who took their degree in mathematics, physics, chemistry or astronomy mostly prepared themselves for jobs as high school teachers, and the whole curriculum was worked out so that they would be competent. The standards were high in the Danish high schools, so this was a very good education. In astronomy, there were other opportunities. For instance, you could join the Geodetic Institute. But the main job was in high school, and to discourage people from choosing the scientific career they had determined then that the salaries of the assistants [at the Observatory] were just a shade lower than you got as a salary when you entered high school teaching. It wasn't looked down upon, but it certainly wasn't encouraged.
Bengt Strömgren um það leyti sem hann lauk doktorsprófi árið 1929, þá tuttugu og eins árs. Þremur árum fyrr hafði þessi stutta frétt birst um hann í Lesbók Morgunblaðsins: Yngsti vísindamaður í heimi. Lesa má um þá feðga, Elis og Bengt, hjá S.O. Rebsdorf, 2004: The Father, the Son, and the Stars: Bengt Strömgren and the History of Danish Twentieth Century Astronomy.
Grunnnámið í stjörnufræði við Háskólann byggðist á fyrirlestrum Elis Strömgren og á námsárum Steinþórs var stuðst við kennslubók frá 1908 eftir Hans Geelmuyden (1844-1920) í Osló. Prófessor Strömgren þótti bókin góð til síns brúks, en þegar líða tók á þriðja áratuginn tók að bera á óánægju stúdenta með námsefnið. Eftir miklar kvartanir þeirra samdi Elis því nýja bók ásamt syni sínum Bengt og gaf út árið 1931. Fyrri hlutinn var í aðalatriðum byggður á gömlu bókinni, en Bengt bætti við nýjum köflum um stjarneðlisfræði þess tíma. Bókin var fljótlega endurskoðuð og þýdd á þýsku. Hún kom í nýrri útgáfu 1945 og var notuð við Kaupmannahafnarháskóla vel fram yfir miðja öldina.
Síða 58 í þýsku útgáfunni af bók þeirra Elis og Bengts frá 1933. Þar er fjallað um leiðréttingar á himinkúluhnitum stjörnunnar S vegna ljósbrots í andrúmsloftinu.
Fjöllistaskólinn við Silfurtorg í Kaupmannahöfn í upphafi tuttugustu aldar. Þar fór grunnkennslan í eðlisvísindum og stærðfræði fram fyrstu þrjú árin, bæði fyrir verkfræði- og raunvísindanema. Meðal annars las Steinþór þar stærðfræði hjá Haraldi Bohr (1887-1951) og Jóhannesi Mollerup (1872-1937). Notast var við Lærebog i Matematisk Analyse eftir þá félaga, sem kom út í fjórum bindum á árunum 1920-1923 og margir eldri Íslendingar kannast við. Mér er ekki kunnugt hverjir kenndu Steinþóri efnafræði, en um eðlisfræðikennsluna á fyrri hluta sáu aðallega þeir Edward S. Johansen (1879-1954) og Júlíus Hartmann (1881-1951). Ljósmynd: Niels Bohr Arkive.
Stofnun Kaupmannahafnarháskóla í kennilegri eðlisfræði (síðar Niels Bohr stofnunin) á þriðja áratugi tuttugustu aldar. Þangað hefur Steinþór mjög líklega sótt tíma í kennilegri eðlisfræði á seinni hluta námsins og nær örugglega framkvæmt verklegar æfingar í kjallaranum. Niels Bohr (1885-1962) var þá hættur allri venjulegri kennslu og á árunum 1924-26 hélt Hendrik A. Kramers (1894-1952) því fyrirlestrana í kennilegri eðlisfræði. Werner Heisenberg (1901-1976) sá síðan um kennsluna frá hausti 1926 til ársloka 1927, þegar Oskar Klein (1894-1977) tók við og kenndi til 1932. Hans M. Hansen (1886-1956) hélt hins vegar fyrirlestra um ljósfræði á þessum árum og sá um verklegu kennsluna á seinni hluta. Ljósmynd: Niels Bohr Archive.
Um stjörnufræðina gildir hið sama og aðrar fræðigreinar, að á tímabilinu frá siðaskiptum (um 1550) fram á þriðja áratug tuttugustu aldar sóttu Íslendingar þekkingu sína á himingeimnum nær eingöngu til Háskólans í Kaupmannahöfn. Það er því mikilvægt, að hinn danski bakgrunnur sé hafður í huga, þegar fjallað er um vísindasögu Íslendinga fyrr á öldum. Í því sambandi má til dæmis benda lesendum á eftirfarandi yfirlitsrit um sögu stjörnufræðinnar í Danmörku, einkum þó við Kaupmannahafnarháskóla:
Á námsárum Steinþórs fór seinnihlutanámið í stjörnufræði fyrst og fremst fram í Stjörnuathugunarstöðinni á Østervold þar sem megináhersla var lögð á sígildar stjarnmælingar og útreikninga á brautum himintungla. Bengt Strömgren hafði eftirfarandi að segja um stöðuna í áðurnefndu viðtali:
There was very little in the way of a development in astronomy [in Copenhagen] at that time. The number of positions in the whole country was very small. There was no equipment to speak of. It was all antiquated, and what was done was that the activities were in the theoretical field, as a consequence. My father's work was in dynamical astronomy, and in the period we are now talking about my work was in theoretical astrophysics. We really didn't have very much to go on at all in that period. The Niels Bohr Institute was the exception.
Með síðustu setningunni er Bengt að vísa til þess, að á þessum árum var unnið að tímamótarannsóknum í skammtafræði við stofnun Háskólans í kennilegri eðlisfræði. Þangað sótti hann sjálfur mikinn innbástur og sem kunnugt er var hann meðal hinna fyrstu í hópi stjarnvísindamanna til að beitta niðurstöðum nýju skammtafræðinnar í rannsóknum í stjarneðlisfræði.
Vesturhlið Stjörnuathugunarstöðvar Kaupmannahafnarháskóla árið 1935. Þarna var stjörnufræðideildin til húsa á árunum 1861-1996. Á tímabilinu 1642-1861 hafði athugunarstöð skólans hins vegar verið á efstu hæð Sívalaturns. Mynd: Wikipedia.
Tiltölulega nýleg mynd af suðurhlið Stjörnuathugunarstöðvarinnar á Østervold. Á námsárum Steinþórs bjó Elis Strömgren prófessor og fjölskylda hans í vesturvængnum og stjörnumeistarinn Julie Marie Vinter Hansen í austurvængnum. Fyrir framan húsið stendur stytta af Tycho Brahe (1546-1601) eftir myndhöggvarann H.W. Bissen. Hún var sett upp árið 1876. Stjörnuathugunum var hætt við stöðina árið 1953 og þær fluttar til Brorfelde. Margir stjörnufræðinganna voru þó þar með skrifstofur allt til ársins 1996, þegar aðstaðan var flutt annað. Þessi fallega bygging hýsir því ekki lengur stjörnufræðina við Háskólann og þar hefur Stofnunin í kennslufræði náttúruvísindagreina nú aðsetur. Ljósmynd: O. Bruchez.
Þegar Steinþór var við nám, skorti talsvert á að tækjabúnaður athugunarstöðvarinnar væri eins og best verður á kosið. Um miðjan tíunda áratug nítjándu aldar hafði fyrsta linsusjónauka stöðvarinnar verið skipt út fyrir annan nýrri (sá gamli er nú í vörslu Steno safnsins í Árósum; sjá grein J.B. Skrivers frá 2008: Det bedste af det bedste, bls. 4-7). Repsold fyrirtækið í Þýskalandi hannaði og smíðaði nýja sjónaukann, svokallaðan tvöfaldan linsusjónauka, sem nota mátti bæði til ljósmyndatöku og athugana með berum augum.
Tvöfaldi pólstillti Repsold sjónaukinn í hvelfingu Østervoldsstöðvarinnar. Þvermál safnglers efri sjónaukans er 36 cm og brennivíddin 4.9 m. Hvíta hylkið á enda neðri sjónaukans er stæði fyrir 15 cm ljósmyndaplötur, sem voru úr gleri. Þvermál safnglersins er 20 cm og brennivíddin 4.8 m. Ljósmyndabúnaðurinn eyðilagðist fyrir slysni árið 1909 og þrátt fyrir tilraunir til viðgerða tókst ekki að hefja viðunandi myndatökur aftur fyrr en á þriðja áratugi tuttugustu aldar. Í millitíðinni voru því flestar stjörnuathuganir gerðar með því að kíkja í 36 cm sjónaukann með berum augum. Rétt er þó að hafa í huga, að tveir stjörnufræðingar komu ávallt að öllum slíkum athugunum, og jafnframt að úrvinnslu mæligagna. Þetta gerði það að verkum, að útgefnar niðurstöður reyndust óvenju nákvæmar og jafnvel til muna betri en sambærilegar niðurstöður frá mörgum öðrum athugunarstöðvum í Evrópu og Bandaríkjunum. – Eftir því sem ég best veit er Repsold sjónaukinn enn á sínum stað í hvelfingunni, þótt byggingin sé nú aðsetur annarra en stjörnufræðinga. Ljósmynd: O.J. Joensen.
Sitt hvoru megin við hvelfingu töfalda linsusjónaukans voru minni herbergi, annars vegar með hágöngukíki (meridianinstrument), hins vegar með þvergöngukíki (passage-instrument).
Hágöngukíkir athugunarsöðvarinnar mun hafa verið settur upp árið 1861. Hann var smíðaður hjá fyrirtækinu Pistor & Martins, brennivíddin var 6 fet og þvermál hringanna 3 fet. Sjónaukinn er nú geymdur á Kroppedal-safninu á Norður Sjálandi. Myndin er fengin að láni úr öðru bindi verksins, Dansk astronomi gennem firehundrede år, bls. 199.
Í stöðinni var einnig þvergöngukíkir frá Repsold, sem settur var upp 1902. Optískur ás hans var brotinn um 90 gráður og því horft í hann frá hlið. Ég hef því miður ekki fundið mynd af þessum sjónauka, en hér er í staðinn mynd af einum álíka frá svipuðum tíma. Sá er af Bamberg gerð og var í eigu Greenwich stjörnuathugunarstöðvarinnar.
Úti í garðinum framan við suðurhlið aðalbyggingarinnar var lítill skáli, sem hýsti svokallaðann Zöllner ljósmæli til mælinga á birtu fastastjarna.
Ég hef ekki enn fundið mynd af Zöllner mæli stöðvarinnar, en þessi mynd er af einum slíkum í Pulkovo stjörnuathugunarstöðinni í Rússlandi. Mælirinn er tækið, sem fest er á enda pólstillta linsusjónaukans. Sjá einnig: Sterken, C. & K.B. Staubermann, 2000: Karl Friedrich Zöllner and the historical dimension of astronomical photometry. Mynd: Getty images.
Starfslið stöðvarinnar var fámennt á námsárum Steinþórs. Auk forstöðumannsins Elis Strömgren prófessors (1870-1947) og stjörnumeistarans J.M. Vinter Hansens (1890-1960) voru þar tveir aðstoðarstjörnufræðingar á hverjum tíma [Jens Johannsen (f. 1897) í tvö ár 1923-1925; Jens P. Møller (1899-1944) alls í tuttugu ár 1924-1944; Axel V. Nielsen (1902-1970) árið 1926 og Bengt Strömgren (1908-1987) í níu ár 1927-1936]. Erna Mackeprang var reiknimeistari og ritari. Ýmsir aðrir komu við sögu, svo sem lausráðnir aðstoðarmenn við útreikninga, athuganir og fleira, meðal annars stúdentar í framhaldsnámi.
Prófessor Svante Elis Strömgren (til vinstri) og Julie Marie Vinter Hansen stjörnumeistari Østervoldsstöðvarinnar. Myndirnar eru frá því um miðjan þriðja áratug tuttugustu aldar, þegar Steinþór Sigurðsson var þar við nám.
Elis Strömgren prófessor og forstöðumaður
Svíinn Svante Elis Strömgren stundaði nám í stjörnufræði við Háskólann í Lundi undir handleiðslu prófessors Axels Möller (1830-1896). Verk hans frá 1896, Berechnung der Bahn des Kometen 1890 II, var tekið gilt til doktorsvarnar árið 1898, en eins og nafnið gefur til kynna fjallar það um braut halastjörnunnar 1890 II (nú skráð sem C/1890 F1 Brooks). Verkið var mikilvægt framlag til halastjörnufræða, eins og komið verður að síðar.
Að loknu doktorsprófi kenndi Strömgren við Lundarháskóla í þrjú ár (1898-1901), gerðist síðan starfsmaður á ritstjórnarskrifstofu hins merka tímarits Astronomische Nachrichten í Kiel í sex ár og var jafnframt Privatdozent í stjörnufræði við Kielarháskóla 1904-1907. Árið 1907 var hann skipaður prófessor í stjörnufræði við Háskólann í Kaupmannahöfn og jafnframt forstöðumaður stjörnuturnsins þar. Því starfi gengdi hann með miklum sóma til sjötíu ára aldurs, árið 1940.
Sérgrein Strömgrens var aflfræði himintungla og auk stjörnuathugana urðu rannsóknir á því sviði því meginviðfangsefni stjörnufræðinga við Kaupmannahafnarháskóla á hans dögum. Hann kenndi stærðfræði- og raunvísindanemun á fyrri hluta stjörnufræði á árunum 1907 til 1940, þar á meðal allnokkrum Íslendingum. Hann var og aðalkennari Steinþórs Siguðssonar til magistersprófs.
Strömgren var vel þekktur á alþjóðavettvangi og hafði um sig stórt net samstarfsmanna, bæði heima og erlendis, einkum þó á hinum Norðurlöndunum og í Þýskalandi. Eins og áður hefur komið fram varð sonur hans, Bengt Strömgren, einn þekktasti stjarnvísinda-maður tuttugustu aldarinnar.
Strömgren feðgarnir árið 1925 á skrifstofu Elis í stjörnuathugunarstöðinni. Frá vinstri talið: Elis, Erik (1909-1993), síðar þekktur geðlæknir í Danmörku, og loks hinn 17 ára gamli Bengt.
J.M. Vinter Hansen stjörnumeistari
Á dögum Steinþórs í Kaupmannahöfn var Julie Marie Vinter Hansen næst æðsti yfirmaður stjörnuathugunarstöðvarinnar og sá að verulegu leyti um daglegan rekstur hennar. Hún hafði lokið magistersprófi í stjörnufræði hjá Strömgren árið 1917, fyrst kvenna í Danmörku, og var ráðin sem aðstoðarstjörnufræðingur við athugunarstöðina 1919. Áður hafði hún starfað þar við stjarnfræðilega útreikninga. Stjörnumeistari varð hún 1922, einnig fyrst kvenna í Danmörku.
Vinter Hansen átti mikilvægan þátt í þjálfun Steinþórs sem stjörnufræðings, enda var hún sérfræðingur í útreikningum á brautum halastjarna og smástirna, auk þess sem hún sinnti margs konar stjarnmælingum. Hún var vel þekkt á alþjóðavettvangi fyrir brautarreikninga og á árum seinni heimsstyrjaldarinnar starfaði hún við Lick stjörnuathugnarstöðina í Kaliforníu. Eftir heimkomuna, 1945, tók hún við sínu fyrra starfi við Østervoldsstöðina, auk þess sem hún sinnti ýmsum störfum fyrir samfélag stjarnvísindamanna, bæði heima og erlendis
Fröken Júlía Vinter Hansen á aðalþingi Alþjóðasambands stjarnvísindamanna (IAU) í Zürich haustið 1948. Hún hafði þá fyrir skömmu tekið við ritstjórninni á fréttaskeytum sambandsins af Elis Strömgren, sem ritstýrði þeim á árunum 1922-1947. Vinter Hansen hélt utan um þessa mikilvægu þjónustu til dauðadags, 1960, en fimm árum síðar voru höfuðstöðvarnar fluttar frá Østervold til Harvard. Sjá nánar í grein Vinter Hansens frá 1955, The International Astronomical News Service, og á núverandi vefsíðu fréttastofunnar.
Eins og þegar hefur komið fram hafði prófessor Strömgren formlega yfirumsjón með allri kennslu í stjörnufræði við Kaupmannahafnarháskóla og kenndi lengi mest af efninu sjálfur. Hann sá um grunnnámskeiðið fyrir stærðfræði- og raunvísindanema og leiðbeindi jafnframt stúdentum í framhaldsnámi. Sem forstöðumaður stjörnuathugunarstöðvarinnar tók hann allar ákvarðanir um rannsóknarsvið, skipulagði verkefni og tók oftar en ekki sjálfur þátt í athugunum og útreikningum. Daglegur rekstur og umsjón með aðstoðarstjörnufræðingunum, nemendum og tækjabúnaði var hins vegar í höndum Vinter Hansen, sem sjálf var afkastamikill og einkar fær vísindamaður.
Strömgren var stjörnufræðingur af gamla skólanum og ekki sérlega hrifinn af hinni nýju stjörnufræði, stjarneðlisfræðinni, sem á þessum tíma var í miklum vexti víða í Evrópu og Bandaríkjunum. Áherslan í rannsóknum Østervoldsstöðvarinnar á hans dögum var því á sviði sígildrar stjörnufræði, eins og nánar verður komið inn á hér á eftir. Það var ekki fyrr en Bengt sonur hans hafði lokið doktorsprófi árið 1929, sem stjarneðlisfræðin hóf innreið sína við Kaupmannahafnarháskóla fyrir alvöru.
Á fyrri hluta tuttugustu aldar sinnti Østervoldsstöðin því mestmegnis hefðbundnum stjarnmælingum og útreikningum. Fylgst var með göngu reikistjarna og tungla þeirra, sólmyrkvum og tunglmyrkvum, sem og stjörnumyrkvum. Sértök áhersla var lögð á að mæla birtu breytistjarna og finna staðsetningu og eiginhreyfingu smástirna og halastjarna með það að markmiði að ákvarða brautir þeirra.
Á kennilega sviðinu var þriggja hnatta vandamálið ásamt tilheyrandi brautarreikningum meginviðfangsefni Strömgrens og þar með stöðvarinnar. Segja má, að á fyrstu áratugum tuttugustu aldar hafi Østervoldsstöðin fyrst og fremst verið þekkt í alþjóðlegu fræðasamfélagi stjarnvísindamanna fyrir framlag sitt á því sviði.
Hvað Steinþór Sigurðsson varðar, er fyrst getið um rannsóknir hans við athugunarstöðina í greinum í Nordisk Astronomisk Tidsskrift árið 1926. Þar eru meðal annars skráðar athuganir hans á birtu breytistjarnanna S Ursae majoris, S Cephei og S Boötis frá árinu 1924. Mér finnst einna athyglisverðast, að Steinþór framkvæmdi þessar mælingar strax á öðru misseri sínu við Háskólann, þá nýorðinn tvítugur.
Steinþór Sigurðsson sumarið 1927 (?), þegar hann var þáttakandi í einum af rannsóknarleiðöngrum landfræðingsins Níelsar Nielsen til Íslands. Myndin er úr grein A. Noe-Nygaards frá 1988: Dansk-íslenskar náttúrurannsóknir á Íslandi milli 1920 og 1940, bls.128.
Í næstu fjórum undirköflum verður rætt stuttlega um helstu viðfangsefni Østervolds-stöðvarinnar á dögum Strömgrens og Vinter Hansens og það sem færsluhöfundi hefur tekist að grafa upp um aðkomu Steinþórs að þeim.
1. Stjarnmælingar af ýmsu tagi
Flestar af mælingum athugunarstöðvarinnar tengdust alþjóðlegu samstarfi stjörnu-fræðinga. Mæliniðurstöðurnar voru oftast birtar á töfluformi í norræna tímaritinu Nordisk Astronomisk Tidsskrift (NAT) og/eða hinu þýska Astronomische Nachrichten (AN) sem H.C. Schumacher (1780-1850), aðalkennari Björns Gunnlaugssonar (1788-1876), hafði komið á fót árið 1821. Þessar niðurstöður voru síðan notaðar víða um heim, ásamt hliðstæðum mælingum annarra stjörnuathugunarstöðva, meðal annars til þess að ákvarða brautir himintungla eða birtuferla breytistjarna. Slíkar lokaniðurstöður birtu stjörnufræðingar tengdir Østervoldsstöðinni svo venjulega í Astronomische Nachrichten.
Myndir af dæmigerðum viðfangsefnum stjörnufræðinganna á Østervold. Til vinstri má sjá halastjörnuna 32P/Comas Solà (= Comas Solà 1926 f) á miðri mynd M. Clarks frá 2005. – Til hægri bendir örin (>) á Trójusmástirnið 624 Hektor (mynd úr Wikipedíu, tekin 2009).
1.1 Breytistjörnur
Til þess að eitthvert gagn sé að, þarf að fylgjast kefisbundið með breytistjörnum í langan tíma, jafnvel árum saman, áður en hægt er að ákvarða birtuferla þeirra með nægjanlegri nákvæmni. Fjöldi breytistjarna er mikill og venjulegar stjörnuathugunarstöðvar hafa hvorki mannafla né nægan athugunartíma til slíkra langtímamælinga. Því var snemma brugðið á það ráð að fá áhugastjörnufræðinga til aðstoðar með því að stofna sérstök félög stjörnufræðinga og áhugastjörnufræðinga um breytistjörnuathuganir.
Á fyrri hluta tuttugustu aldar varð smám saman ljóst að taka þurfti breytistjörnur, ekki síst svokallaðar sveiflustjörnur, með í reikninginn í kennilegum líkönum um þróun sólstjarna. Þótt Elis Strömgren væri ekki sérlega áhugasamur um slík fræði, rann honum blóðið til skyldunnar og tók að sér að skipuleggja norræna samvinnu um breytistjörnuathuganir árið 1920. Hann hafði sjálfur yfirumsjón með með þessu starfi, fyrst einn, en síðar í samvinnu við fyrrum nemanda sinn, Axel V. Nielsen í Árósum.
Tvær ljósmyndir af ónefndri breytistjörnu, teknar með talsverðu millibili. Þær eru fengnar að láni af vefsíðu AAVSO: Variables: What Are They and Why Observe Them? Breytingin á birtu þessarar stjörnu (í miðjum hringnum) sést vel með samanburði við stjörnurnar umhverfis hana á hvelfingunni.
Eins og áður hefur komið fram, voru fyrstu skráðu stjörnuathuganir Steinþórs ákvörðun á birtu þriggja breytistjarna, þ.e. S Ursae majoris, S Cephei og S Boötis, sem allar teljast til svokallaðra Mírustjarna. Mælingarnar voru gerðar á mismunandi tímum á tímabilinu frá febrúar 1924 til apríl 1925 og birtar í eftirfarandi greinum í Nordisk Astronomisk Tidsskrift:
Lokaniðurstöðurnar úr framangreindum mælingum birtust svo hér:
1.2 Staðsetning stjarna á hvelfingunni
Á Østervoldsstöðinni var fylgst vel með hreyfingum smáhnatta í sólkerfinu til að fá sem nákvæmastar upplýsingar um brautir þeirra. Helstu viðfangsefnin voru halastjörnur, en einnig tungl reikistjarnanna sem og smástirni, bæði í smástirnabeltinu milli Mars og Júpíters og eins í Trójuþyrpingunum tveimur á braut Júpíters. Á námsárum Steinþórs stjórnaði Vinter Hansen þessum athugunum og sá um birtingu mæliniðurstaða. Mælingarnar voru yfirleitt framkvæmdar með tvöfalda Repsold sjónaukanum, sem áður var nefndur.
Einföld teikning af smástirnabeltinu milli reikistjarnanna Mars og Júpíters, en jafnframt má sjá votta fyrir Trójusmástirnum á braut Júpíters. NASA birti myndina í tilefni heimsóknar geimfarsins Dögunar til smástirnanna Vestu og Ceresar á árunum 2011 til 2018.
Steinþór tók þátt í slíkum mælingum í mars og apríl árið 1926. Verkefni hans var að finna tímabundna staðsetningu smástirnanna Vestu, Þalíu, Amalþeu, Kaldeu og Eleonoru á hvelfingunni og gefa stöðuna upp í miðbaugshnitum (þ.e. stjörnulegd α og stjörnubreidd δ). Niðurstöðurnar voru síðan birtar í eftirfarandi grein í Astronomische Nachrichten:
1.3 Stjörnumyrkvar
Með stjörnumyrkvum er átt við þá atburði, þegar einn himinhnöttur gengur fyrir annan og hylur hann um tíma. Oftast er hugtakið þó notað, þegar tungl hylur fastastjörnur eða hnetti sólkerfisins (nema sólina, þá er talað um sólmyrkva) á leið sinni um dýrahringinn. Lengi vel var einkum fylgst með stjörnumyrkvum til að ákvarða tunglbrautina með sem mestri nákvæmni, en nú á dögum er ekki lögð minni áhersla á mælingar til að fylgjast með möndulsnúningi jarðar, mæla sýndarþvermál stjarna og leita að tvístirnum.
Steinþór tók þátt í nákvæmnismælingum af þessu tagi á tímabilinu frá apríl 1928 til júní 1929, en þá gekk tunglið fyrir reikistjörnuna Venus og fjölmargar fastastjörnur í dýrahringnum, meðal annars λ Cancri, κ Virginis, 103 Tauri og γ Virginis. Þar fékk hann meðal annars tækifæri til að vinna með Bengt Strömgren og voru niðurstöður þeirra fyrst birtar í Nordisk Astronomisk Tidsskrift:
Aðrir unnu síðan úr þessum mælingum og birtu lokaniðurstöður í Astronomische Nachrichten:
Tunglið myrkvaði reikistjörnuna Venus einu sinni sem oftar hinn 7. desember 2015. Sjá myndband af atburðinum hér. Myndbandið var tekið í Texas og hér er það fengið að láni hjá YouTube. Myndbandið gefur einhverja hugmynd um samskonar atburð, sem þeir Steinþór og Bengt Strömgren fylgdust með og mældu á Østervoldsstöðinni 4. júní 1925.
2. Útreikningar á brautum himintungla
Lesendum sem vilja kynna sér sögu vísindanna um aflfræði himintungla og tilheyrandi brautarreikninga, er bent á eftirfarandi ritsmíðar og heimildirnar sem þar er vísað á:
Á námsárum Steinþórs voru tölvur ekki enn komnar til sögunnar og brautarreikningar voru því mikil og tímafrek vinna. Mér skilst, að á þessum árum hafi, auk blýantsreikninga með hjálp lógariþmataflna, einkum verið notast við handsnúna Archimedes reiknivél svipaðri þeirri, sem sjá má á myndinni hér fyrir neðan. Þegar líða tók á fjórða áratuginn mun Østervoldsstöðin loks hafa fengið rafknúna útgáfu af þessari tegund. Tölvureikningar hófust hins vegar ekki fyrr en á sjöunda áratugnum.
Myndin sýnir handsnúna Archimedes reiknivél af svokallaðri D gerð. Í þessu myndbandi er útskýrt, hvernig vélin var notuð (sjá einnig hér). Á Østervold var jafnframt stuðst við margs konar stjarnfræðitöflur og ekki síst lógariþmatöflur, sem allt fram á tölvuöld voru mjög mikilvægt hjálpartæki við flókna og tímafreka útreikninga.
Lítið var um reiknitæknilegar nýjungar í brautarreikningum á þriðja áratugnum, nema hvað Bengt Strömgren þróaði sína eigin aðferð árið 1929, sem átti eftir að njóta talverðrar hylli (sjá í því sambandi greinar hans, Formeln und Tafeln zur Bestimmung parabolischer Bahnen og Formeln zur genaeherten Stoerungsrechnung in Bahnelementen. Angewandt auf die Planeten 633 Zelima, 956 [1921 IW], 979 Ilsewa, 1035 Amata und 1049 [1925RB]). Að öðru leyti voru notaðar margreyndar aðferðir af því taginu sem lýst er í eftirfarandi ritum:
Þetta voru helstu heimildirnar um stjarnfræðilega útreikninga, sem reiknimeistarar stjörnuathugunarstöðva í Evrópu studdust við á fyrstu áratugum tuttugustu aldar.
Þegar mikið var að gera við útreikninga á Østervoldsstöðinni tóku allir, sem vettlingi gátu valdið, þátt í vinnunni, þar á meðal forstöðumaðurinn, stjörnumeistarinn, aðstoðarstjörnufræðingar, stúdentar og aðrir hjálparkokkar. Það má því teljast nokkuð öruggt, að Steinþór hefur tekið þátt öllum helstu reikniverkefnum stöðvarinnar, þótt hans sé ekki ávallt getið, frekar en annarra aðstoðarmanna, þegar niðurstöðurnar voru birtar.
Á þessum árum var Østervoldsstöðin í raun einskonar reiknimiðstöð fyrir raunvísindadeildir Háskólans. Meðal annars sáu starfsmenn hennar um margvíslega flókna útreikninga í atómeðlisfræði fyrir kennilegu eðlisfræðingana á Blegdamsvej.
2.1 Tveggja hnatta vandamálið
Allt frá því í lok sautjándu aldar hafa brautarreikningar stjarnvísindamanna hvílt á þem trygga grunni, sem Ísak Newton (1642-1727) lagði með frægri stærðfræðilegri lausn sinni á svokölluðu tveggja hnatta vandamáli. Viðfangsefnið er að finna brautir tveggja hnatta undir gagnkvæmum þyngdaráhrifum í óendanlegum evklíðskum heimi sem er að öðru leyti tómur. Newton sýndi fram á brautirnar eru einslaga keilusnið þar sem massamiðja kerfisins er í sameiginlegum brennipunkti (sjá háskólakennslubækur í stjörnufræði og/eða aflfræði).
Í sólkerfinu vill svo heppilega til, að sólin er í algjöru aðalhlutverki. Þegar kanna þarf hreyfingu mun minni hnattar um sólu, til dæmis reikistjörnu, smástirnis eða halastjörnu, er það því góð fyrsta nálgun að gera ráð fyrir, að brautin sé keilusnið með sólina í brennipunkti. Í nákvæmari reikningum þarf í framhaldinu að taka tillit til truflana vegna þyngdaráhrifa annarra nálægra hnatta (og afstæðiskenningarinnar, ef svo ber undir). Þegar um tungl reikistjarna er að ræða, er sama aðferð notuð, en nú með viðkomandi reikistjörnu í hlutverki sólar.
Á myndinni er sólin sett í sameiginlegan brennipunkt þriggja keilusniða. Sjá má, að í sólnánd er fleygbogi (parabóla) góð nálgun, bæði við gleiðboga (hýperbólu) og sporbaug (ellipsu). Hins vegar vex munurinn ört eftir því sem fjær dregur sólu.
Eftir að tekist hefur að ákvarða nægilega marga punkta á ferli hnattar á hvelfingunni með stjarnmælingum (fræðilega duga þrír punktar, en oft eru notaðir fleiri), taka hinir flóknu brautarreikningar við. Nær undantekningalaust eru leiðréttar mæliniðurstöður skráðar í kúluhnitum, t.d. hornunum α og δ fyrir hvern punkt. Út frá frá þessum sex (eða fleiri) hornum eru hinar svokölluðu grunnstærðir brautarinnar ákvarðaðar með reikningum, þar sem gert er ráð fyrir að brautin sé keilusnið í þrívíðu rúmi með sólina í brennipunkti – í stjörnufræði eru slíkar keilusniðsbrautir oft kallaðar Keplersbrautir. Grunnstærðirnar eru sex, eins og sýnt er á eftirfarandi mynd fyrir sporbaugsbraut.
Grunnstærðir brautar (stundum kallaðar brautarstikar) ótruflaðs hnattar (celestial body), þ.e. reikistjörnu, smástirnis eða halastjörnu á Keplersbraut um sólina. Á myndinni táknar gráa svæðið (plane of reference) jarðbrautarplanið með sólina sem miðju. Rauða línan til hægri (reference direction) stefnir á vorpunktinn γ. Svarti ferillinn sem afmarkar gula svæðið er braut (orbit) hnattarins um sólina. Í þessu tilviki gengur hnötturinn eftir sporbaug með sólina í öðrum brennipunktinum. Fjólubláa línan tengir saman sólina og sólnándarpunkt hnattarins. Skurðlína jarðbrautarplansins og brautarplans hnattarins sést greinilega á myndinni. Braut hnattarins sker jarðbrautarplanið í tveimur gagnstæðum punktum: rishnútnum (ascending node) og sighnútnum (descending node). - Grunnstærðir brautarinnar eru sex og eru fjórar þeirra sýndar á myndinni, þ.e. hornin Ω = sólbaugslengd rishnúts (longitude of ascending node), i = brautarhalli (inclination), ω = stöðuhorn sólnándar (argument of periapsis) og ν = rétt brautarhorn (true anomaly). Síðustu tvær grunnstærðirnar eru svo e = miðskekkja (eccentricity) og a = hálfur langás (semimajor axis) sporbaugsbrautarinnar. Að auki er oft notast við stærðina M = meðalbrautarhorn (mean anomaly). (Til gamans má geta þess, að í aflfræði eru Ω, i og ω kölluð Eulershorn (gula) brautarplansins.) Mynd: Wikipedia.
Grunnstærðirnar ákvarða svokallaða snertibraut (osculating orbit) hnattarins, það er bestu Keplersbrautina, sem fellur að mæliniðurstöðunum. Út frá henni og með því að taka tillit til truflandi áhrifa nálægra himintungla, einkum reikistjarna, má síðan finna betri nálgun á braut hnattarins, bæði fram og aftur í tíma. Þetta þarf venjulega að endurtaka aftur og aftur, jafnvel árum og áratugum saman, og lengur ef um smáhnetti er að ræða. Allt fram á tölvuöld var þessi reiknivinna ekki aðeins flókin, heldur ákaflega tímafrek.
2.2 Brautir halastjarna og smástirna
Á stórum hluta nítjándu aldar og fyrstu áratugum þeirrar tuttugustu var brautarhreyfing smástirna og halastjarna eitt helsta viðfangsefni margra athugunarstöðva í Evrópu og Bandaríkjunum. Þessir smáhnettir gátu orðið fyrir verulegum truflunum frá reikistjörnum á leið sinni um sólkerfið, einkum þó risunum miklu, Júpíter og Satúrnusi.
Doktorsritgerð Strömgrens í Lundi fjallaði einmitt um áhrif slíkra truflana á braut halastjörnunnar 1890 II, sem nú er skráð sem C/1890 F1 Brooks. Árið 1890 sýndu fjölmargar mælingar og viðamiklir reikningar Strömgrens, að stjarnan væri á opinni gleiðbogabraut (e > 1). Með því reikna aftur í tímann og taka tillit til truflandi áhrifa reikistjarnanna tókst honum hins vegar að sýna fram á, að miðskekkja brautarinnar hefði áður verið minni og farið vaxandi á árunum 1886 til 1890. Reikningar á brautum nokkurra annarra halastjarna sýndu sömu hegðun og árið 1914 setti Strömgren fram þá tilgátu, að þessar stjörnur hefðu upphaflega verið á mjög ílöngum og lokuðum sporbaugsbrautum (e < 1) og þar með tilheyrt sólkerfinu. Truflandi þyngdarhrif stóru reikistjarnanna hefðu svo smám saman breytt flestum brautanna úr lokuðum í opnar (e ≥ 1).
Í kjölfar þessara mikilvægu niðurstaða Strömgrens var fljótlega farið að skipta meginhluta halastjarna í tvo flokka, annars vegar halastjörnur með stuttan umferðartíma og hins vegar langan. Innan nokkurra áratuga urðu þær að lokum til þess, að Hollendingurinn J.H. Oort (1900-1992) setti fram hina vel þekktu tilgátu sína, að svo til allar halastjörnur með langan (eða óendanlegan) umferðartíma eigi uppruna sinn í kúlulaga hjúpi umhverfis sólkerfið, hjúpi sem nú er við hann kenndur og kallaður Oortsskýið.
Nokkrum árum eftir að Strömgren tók við stjórn Østervoldsstöðvarinnar ákvað hann að stórauka áhersluna á venjulega brautarreikninga. Þar kann að hafa ráðið nokkru, að í upphafi fyrri heimsstyrjaldarinnar tók hann að sér að miðla fréttum af nýuppgötvuðum fyrirbærum á stjörnuhimninum til alþjóðasamfélags stjarnvísindamanna. Eins og áður hefur komið fram var fréttaþjónusta IAU svo sett undir hans stjórn árið 1922. Það gerði það meðal annars að verkum, að stjörnufræðingarnir á Østervold voru yfirleitt með þeim fyrstu til að frétta af nýjum halastjörnum og fundi nýrra smástirna.
Frá stöðinni tók því að berast stöðugur straumur fréttaskeyta og greina með grunnstærðum og öðrum niðurstöðum fyrir brautir halastjarna og smástirna, ekki síst eftir að Vinter Hansen kom til sögunnar. Gott yfirlit um alla þá vinnu má finna í eftirfarandi grein, sem á ekki síður við um smástirni en halastjörnur:
Þarna kemur meðal annars fram, að þegar mikið var að gera tóku allir starfsmenn stöðvarinnar þátt í að sannreyna innsendar upplýsingar og ganga frá nauðsynlegum útreikningum í tengslum við fréttirnar. Það virðist því nokkuð ljóst, að Steinþór hefur, oftar en einu sinni, komið að slíkum reikningum. Í því sambandi má til dæmis benda á fréttaskeytið/kortið frá 19. nóvember 1926 með upplýsingum um hina þá nýfundnu halastjörnu Comas Solà 1926 f (stundum kölluð öðrum nöfnum, en nú nær eingöngu 32P/Comas Solà). Þar má meðal annars sjá niðurstöður stjörnufræðingsins A.C.D. Crommelins (1865-1939) fyrir grunnstærðir brautarinnar:
Fljótlega eftir að fréttirnar um halastjörnuna bárust til Kaupmannahafnar var Steinþór búinn að semja stutta grein, Komet Comas Solà (1926 f), þar sem hann notaði grunnstærðir Crommelins til að teikna skemmtilega rúmsjármynd (sjá einnig hér) af brautinni:
Rúmsjármynd af braut halastjörnunnar Comas Solà 1926 f ásamt brautum jarðar (innst) og Mars. Myndin er úr grein Steinþórs Sigurðssonar frá 1927: Komet Comas Solà (1926 f). Þótt grunnstærðirnar séu frá Crommelin komnar er ljóst, að Steinþór hefur sjálfur reiknað staðsetningar, bæði halastjörnu og jarðar, í hinum sérmerktu punktum. Heldur nýrri upplýsingar um þessa tilteknu halastjörnu má finna hér.
Til gamans má geta þess, að af einhverjum mér ókunnum ástæðum var þessi tiltekna halastjarna í miklu uppáhaldi hjá Vinter Hansen:
Einnig er rétt að nefna það hér, að rannsóknir á brautum Trójusmástirna var sérstakt gæluverkefni þeirra Strömgrens og Vinter Hansens. Það er án efa ein helsta ástæða þess, að magistersritgerð Steinþórs snerist um eitt þeirra, Akkilles, eins og ítarlega verður fjallað um undir lok færslunnar.
3. Lausnir á þriggja hnatta vandamálinu
Þriggja hnatta vandamálið er aðeins eitt hinna svokölluðu fjölhnatta vandamála í sígildri stjarneðlisfræði. Tveggja hnatta vandamálið tilheyrir einnig þeim flokki, en hefur þá sérstöðu, að til er almenn stærðfræðileg lausn á endanlegu og lokuðu formi, nokkuð sem ekki er um að ræða, ef hnettirnir eru þrír eða fleiri. Í þeim tilvikum þarf nær undantekningalaust að nota sérstakar nálgunaraðferðir og/eða tölulega reikninga við leit að brautarlausnum. Ef aðstæður krefjast þess að beita þurfi almennu afstæðiskenningunni, verða reikningarnir margfalt flóknari, jafnvel þegar aðeins er um tvo hnetti að ræða.
Þekktasta þriggja hnatta þrautin fjallar um kerfið tungl, jörð og sól, þar sem viðfangsefnið er fyrst og fremst gangur tunglsins um jörðina undir truflandi þyngdaráhrifum sólarinnar. Newton glímdi fyrstur manna við þetta erfiða verkefni í Principíu árið 1687, enda skipti það hann miklu, að þyngdarlögmálið gæfi fullnægjandi skýringu á hreyfingu tunglsins á stjörnuhimninum. Þrátt fyrir að innleiða truflanareikning til að lýsa áhrifum sólarinnar, tókst honum aðeins að leysa vandamálið að hluta og það var ekki fyrr um miðja átjándu öld, sem þeir A.C. Clairaut (1713-1765), J. d’Alembert (1717-1783) og L. Euler (1707-1783) fundu viðunandi lausn(ir), byggða(r) á þyngdarlögmálinu.
Á árunum í kringum 1770 tókst þeim Euler og J.-L. Lagrange (1736-1813) að finna nokkrar sérstækar lausnir á þriggja hnatta þrautinni. Þeirra þekktust er lausnin á svokölluðu takmörkuðu þriggja hnatta vandamáli, þar sem smáögn með hverfandi massa hreyfist í brautarplani „tvístirnis“. Í planinu eru fimm jafnvægispunktar, nú eingöngu kenndir við Lagrange, þótt Euler hafi reyndar fundið þrjá hina fyrstu. Á myndinni hér fyrir neðan eru punktarnir táknaðir með L1, L2, L3, L4, og L5.
Hvítu hringlaga svæðin á myndinni tákna tvo hnetti með með massa M og m, þar sem M/m = 10. Hnettirnir snúast undir gagnkvæmun þyngdaráhrifum um sameiginlega massamiðju. Myndin er teiknuð í viðmiðunarkerfi, sem snýst með hnöttunum og sýnir jafnmættislínur virka þyngdarmættisins í grunnplaninu. Lagrangepunktarnir eru merktir sérstaklega. Rauði þríhyrningurinn er ávallt jafnhliða á myndum sem þessum, óháð massahlutfalli hnattanna. L1, L2 og L3 eru óstöðugir jafnvægispunktar, en L4 og L5 eru hins vegar stöðugir vegna Coriolis-hrifa, en aðeins ef M/m > 25 (sjá nánar hér). Teikningin er fengin að láni af vefsíðu D.D. Noltes.
Lagrange og flestir sporgöngumenn hans töldu, að þessi stærðfræðilega lausn hefði lítið sem ekkert vísindalegt gildi, þar sem kerfi af þessu tagi kæmu hvergi fyrir í náttúrunni. Það viðhorf breyttist þó smám saman meðal stærðfræðinga, fyrst með hinni frægu verðlaunaritgerð H. Poincarés (1854-1912) um þriggja hnatta vandamálið árið 1890, og síðan með þriggja binda tímamótaverkinu um aflfræði himintungla, sem hann gaf út á árunum 1892-1899. Meðal stjörnufræðinga jókst áhuginn á lausn Lagranges hins vegar verulega með uppgötvun fyrstu fjögurra Trójusmástirnanna á árunum 1906 til 1908.
Í ritgerð Poincarés frá 1890 kemur fram (bls. 6), að hann taldi nær útilokað að hægt væri að finna almenna lausn á þriggja hnatta þrautinni með þáverandi stærðfræðitækni. Það hefði því væntanlega komið honum á óvart, hefði hann lifað, að árið 1912 sýndi finnski stærðfræðingurin K.F. Sundman (1873-1949) fram á, að slík lausn væri til á formi samleitinnar, en óendanlegrar, raðar.
Þessari niðurstöðu Sundmans var upphaflega tekið með miklum fögnuði í hópi stjörnufræðinga, en fljótlega kom í ljós, að samleitni raðarinnar var svo hæg, að hún reyndist ónothæf til stjarnfræðilegara útreikninga. Sem dæmi má nefna, að til þess að ákvarða breytingu á stöðu og hraða hnattanna í þriggja hnatta kerfi á tiltölulega stuttu tímabili með nægjanlegri nákvæmni, þarf að taka að minnsta kosti fyrstu 108.000.000 liði raðarinnar með í reikninginn.
Hér eru svo til viðbótar nokkur fróðleg yfirlitsverk um sögu þriggja hnatta vandamálsins:
3.1 Kaupmannahafnar-verkefnið
Eins og margir aðrir stjörnufræðingar virðist Elis Strömgren hafa fengið áhuga á takmörkuðu þriggja hnatta þrautinni fljótlega eftir að tilkynnt var um fund fyrsta Trójusmástirnisins, Akkilesar, árið 1906. Einnig er ljóst, að hann var undir áhrifum frá hinum þekktu fræðimönnum J.A.H. Gyldén (1841-1896) í Stokkhólmi og C.V.L. Charlier (1862-1934) í Lundi, sem báðir höfðu fengist við þriggja hnatta vandamálið, líkt og margir aðrir stjörnufræðingar og stærðfræðingar á dögum Poincarés. Sjálfur nefnir Strömgren, að Englendingurinn G.H. Darwin (1845-1912) hafi haft veruleg áhrif á verk hans á þessu sviði og einnig hafi greinar, sem forveri hans í Kaupmannahöfn, T.N. Thiele (1838-1910), samdi ásamt aðstoðarmanni sínum, C. J. Burrau (1867-1944), hvatt hann til dáða.
Aðeins nokkrum árum eftir embættistöku Strömgrens í Kaupmannahöfn árið 1907, kom hann af stað skipulagðri vinnu fjölda stjörnufræðinga og aðstoðarmanna að verkefni, sem fjlótlega fékk viðurnefnið Kaupmannahafnar-verkefnið (The Copenhagen Problem) meðal sérfræðinga. Verkefnið stóð yfir í rúma tvo áratugi og að því komu samtals í kringum sextíu manns, að stúdentum meðtöldum. Það var enn í fullum gangi á námsárum Steinþórs Sigurðssonar í Kaupmannahöfn, en ekki hef ég fundið neinar beinar heimildir um að hann hafi tekið þátt í reikningunum. Það kann þó vel að vera, því ekki var til siðs á þeim árum að geta sérstaklega um framlög stúndenta í verkefnum sem þessum, þar sem litið var á slíka vinnu sem hluta af námi þeirra. Þó er rétt að nefna, að í magistersprófinu í nóvember 1929 þurfti Steinþór að halda opinberan fyrirlestur um efnið „Numeriske Beregninger over Trelegemeproblemet“.
Kaupmannahafnar-verkefnið snerist um það að nota tölulega reikninga til að finna sem flestar lotubundnar brautarlausnir af svokallaðri fyrstu tegund (premiére sorte) hins takmarkaða þriggja hnatta vandamáls fyrir tilfellið M = m. Almenna flokkunin er frá Poincaré komin (sjá grein 39 í fyrsta bindi Les méthodes nouvelles og ritgerð Poincarés frá 1884: Sur certaines solutions particulières du problème des trois corps): Fyrsta tegundin nær yfir lotubundnar brautir smáhnatta (með hverfandi massa) um tvo massamikla hnetti (M og m) á hringlaga brautum um sameinginlega massamiðju (sjá myndina hér að framan). Í tegund tvö (deuxiéme sorte) eru brautir stóru hnattanna sporbaugar og í þeirri þriðju (troisiéme sorte) aftur hringir, en brautarplön smáhnattanna halla miðað við tvístirnisplanið.)
Nokkrar áhugaverðar greinar um lotubundnar lausnir frá lokum nítjándu aldar:
Yfirlit yfir þær lotubundnu brautir á takmarkaða þriggja hnatta vandamálinu, sem reiknaðar voru við Østervoldsstöðina undir stjórn Strömgrens á tímabilinu 1913 til 1925. Sérhver lokaður ferill er braut smáhnattar (með hverfandi massa) í þyngdarsviði tvístirnis þar sem hnettirnir eru jafnþungir (M = m) og á hringlaga brautum um sameiginlega massamiðju. Smáhnettirnir hreyfast í brautarplani tvístirnisins. Myndin er úr grein E. Strömgrens frá 1933: Connaissance actuelle des orbites dans le probléme des trois corps.
Nokkrar gagnlegar yfirlitsgreinar um Kaupmannahafnar-verkefnið:
4. Ákvörðun tímans og almanaksreikningar
Á námsárum Steinþórs sá Østervoldsstöðin um svokallaða tímaþjónustu í Danmörku. Tíminn var mældur með vandaðri Riefler pendúlklukku, sem keypt hafði verið árið 1903, og tímamerki send til valinna viðtakenda á skipulagðan hátt. Réttur gangur klukkunnar var og reglulega sannreyndur með tilheyrandi stjörnuathugunum.
Til vinstri: Vörulistamynd af Riefler klukku af þeirri gerð, sem notuð var sem tímastaðall við Østervoldsstöðina frá 1903. Byrjað var að framleiða þessa tegund árið 1902. – Til hægri: Þrátt fyrir að stjörnufræðideild Háskólans hafi flutt frá Østervold árið 1996, prýðir mynd af gömlu stöðinni enn forsíðu danska háskólaalmanaksins.
Grunnupplýsingarnar, sem notaðar voru við almanaksreikningana á Østervold, munu hafa borist þangað á formi stjörnualmanaka (sjá einnig hér) frá Englandi í gegnum Svíþjóð. Samt var gífurlegt vinna fólgin í útreikningum og yfirferð danska almanaksins og allir, sem vettlingi gátu valdið á stöðinni, voru virkjaðir meðan á henni stóð. Þar á meðal voru framhaldsnemar og Steinþór mun því án efa hafa tekið einhvern þátt í verkinu á námsárunum, og það líklega oftar en einu sinni.
Í þessu sambandi er rétt að minna á, að á árunum 1861 til 1922 var íslenska almanakið einnig reiknað á Østervoldsstöðinni (fyrir þann tíma, þ.e. 1837 til 1860, var það reiknað í Sívalaturni). Um það leyti, sem Steinþór var í 5. bekk í MR (1921-1922), urðu hins vegar þau tímamót, að almanaksreikningarnir fluttust til Íslands og voru það kennarar hans þar, Ólafur Dan Daníelsson og Þorkell Þorkelsson, sem tóku þá að sér. Fyrsta almanakið sem þeir reiknuðu var fyrir árið 1923.
Til vinstri: Forsíða Almanaksins 1922, síðasta íslenska almanaksins sem reiknað var við Østervoldsstöðina í Kaupmannahöfn. – Til hægri: Forsíða Almanaksins 1923, fyrsta íslenska almanaksins, sem íslenskir fræðimenn reiknuðu eftir að reglugerð var sett um útgáfu þess í febrúar 1922. Hér má fræðast nánar um sögu Íslandsalmanaksins. Sjá einnig: Jón Ragnar Stefánsson, 1998: Reiknimeistarar Almanaksins. Í bókinni Leifur Ásgeirsson: Minningarrit, bls. 144-150.
Árið 1926 hefur sérstakan sess í sögu alþýðufræðslu í stjarnvísindum hér á landi. Þá voru, nær samtímis, gefnar út tvær bækur eftir íslenska höfunda um stjörnufræði og heimsfræði, hinar fyrstu frá því verk Sigurðar Þórólfssonar kennara og búfræðings (1869-1929), Á öðrum hnöttum, kom á prenti 1915.
Höfundar nýju bókanna voru þeir Ágúst H. Bjarnason heimspekiprófessor (1875-1952) og Ásgeir Magnússon kennari og fréttastjóri (1886-1969). Báðir voru þeir áhugamenn um raunvísindi, líkt og forveri þeirra, Sigurður, og höfðu skrifað talsvert um slík efni í tímarit, ekki síst Ágúst.
Næstu árin hélt Ásgeir áfram, ásamt ýmsum öðrum, að kynna löndum sínum nýjungar í stjarnvísindum með greinaskrifum. Ágúst skrifaði hins vegar nýja og heldur víðfeðmari bók, Heimsmynd vísindanna, sem út kom 1931 (sjá einkum bls. 62-138). Á síðustu árunum fyrir stríð bættist Björn Franzson (1906-1974) svo í hóp bókarhöfunda með Efnisheiminum (1938), þar sem rætt er um stjarnvísindi í kaflanum „Hinn mikli heimur“ (bls. 164-185). Um þessi efni var svo ekki fjallað aftur á bókarformi fyrr en kennarinn og rithöfundurinn Hjörtur Halldórsson (1908-1977) þýddi og gaf út Uppruna og eðli alheimsins (1951) eftir F. Hoyle (1915-2001) með formálsorðum eftir Trausta Einarsson.
Á þessum tíma sögðu dómar um ný fræðslurit oft heilmikið um stöðu mála á viðkomandi fræðasviði, bæði hér heima og erlendis. Þegar bækur þeirra Ásgeirs og Ágústs komu út, var Steinþór enn við nám í Kaupmannahöfn og þar sem þær fjölluðu um sérsvið hans, stjörnufræði, hefur hann talið sér skylt að birta umsagnir um þær báðar. Aðrir sögðu einnig sitt álit, eins og fram kemur hér á eftir.
Forsíður bóka þeirra Ágústs H. Bjarnasonar of Ásgeirs Magnússonar, sem út komu árið 1926. Báðar fengu þær góðar viðtökur meðal almennings og voru talsvert lesnar, ekki síst Himingeimur Ágústs.
Vetrarbraut
Bók Ásgeirs er safn greina, sem hann hafði áður birt í tímaritunum Verði og Iðunni á árunum 1924 til 1926. Honum tekst að gefa bærilega (en frekar sundurlausa) lýsingu á þáverandi stöðu þekkingar á sólstjörnum og öðrum fyrirbærum í Vetrarbrautinni, efni sem hann hefur aflað sér með lestri erlendra alþýðurita. Því miður vitum við ekki hver ritin voru, því Ásgeir getur ekki heimilda. Hins vegar nefnir hann í niðurlagsorðum (bls. 165), að hann hafi leitað til dr. Ólafs Daníelssonar um ýmislegt „sem máli skipti“.
Himingeimurinn
Að stofni til er bók Ágústs hefðbundið sögu- og fræðslurit um stjörnufræði og heimsmynd hennar. Höfundurinn styðst einkum við gömul þýsk alþýðurit, svokallaðar Kosmosbækur frá árunum 1888 til 1917 (ritin eru talin upp á bls. 8). Þá vísar hann neðanmáls (bls. 179) í sjöundu útgáfuna af hinu þekkta verki, Newcomb-Engelmann’s Populäre Astronomie (1922). Í lokin er svo stuttur eftirmáli um Einstein og kenningar hans. Þar styðst Ágúst aðallega við skrif B. Russells, sem birtust í The ABC of Relativity (1925) og vitnar jafnframt í Space, Time and Gravitation (1920) eftir A.S. Eddington. – Aftast í formálsorðum (bls. 7) er stúd. mag. Steinþóri Sigurðssyni þakkað fyrir yfirferð á handriti.
Steinþór mun fljótlega hafa kosið að fara í rannsóknarnám (mag. scient.), sem vel fram yfir miðja tuttugustu öld var einskonar millistig milli venjulegs kandídatsnáms (cand. scient.) og „stóru doktorsritgerðarinnar“ (dr. phil.) eins og þeirri, sem Ólafur Dan Daníelsson hafði varið 1909, fimm árum eftir magisterspróf sitt í stærðfræði. Sigurkarl Stefánsson valdi aftur á móti kandídatsnámið og útskrifaðist því rúmu ári á undan Steinþóri.
Höfundi þessara orða er ekki að fullu ljóst, hvaða námskeið Steinþór tók á seinnihluta við Háskólann. Í minningargrein sinni í Alþýðublaðinu segir Sigurkarl hins vegar, að með stjörnufræðináminu hafi hann einnig tekið námskeið í landmælingum og ljósmyndagerð. Í æviskrám er þess jafnframt getið, að á námsárunum 1925 til 1929 hafi Steinþór sjálfur kennt á (árlegu?) námskeiði um mælingar með sextungi (Kursus i Sekstantobservation).
Þegar að hápunkti rannsóknarvinnunnar kom, fékk Steinþór það lokaverkefni að nota allar þekktar mælingar til að finna bestu nálgunina á braut Akkillesar um sólina á árunum 1906 til 1929 með því að taka tillit til þyngdartruflana frá reikistjörnunum Júpíter og Satúrnusi. Jafnframt að ákvarða í lokin nýjustu snertibraut smástirnisins með sem mestri nákvæmni (sjá nánar um hugtakið snertibraut í undirkafla 2.1). Heiti magistersverkefnisins var Baneforbedring for Planet af Jupitergruppen (588) Achilles. Ekki er vitað, hvenær Steinþór hóf vinnu við reikningana fyrir alvöru, en sennilega hefur það þó verið haustið 1928, því í byrjun október sama ár birtist eftirfarandi frétt í vikublaðinu Reykvíkingi:
Frétt úr Reykvíkingi, 4. október 1928, bls. 571. Athugið að í lok annarrar efnisgreinar kemur fyrir orðið flötur, en sennilega á að standa þar töflur.
Örstutt forsaga
Um miðjan febrúar 1906 þekktu stjörnufræðingar alls 587 smástirni, öll í smástirnabeltinu milli Mars og Júpíters. Þegar þýski stjörnufræðingurinn M. Wolf (1863-1932) fann nýtt smástirni hinn 22. febrúar, gaf hann því bráðabirgðaheitið 1906 TG, en síðar fékk það númerið 588 og nafnið Akkilles. Það reyndist vera utan smástirnabeltisins og nálægt Júpíter. Fljótlega eftir uppgötvun Wolfs stakk sænski stjörnufræðingurinn C.V.L. Charlier (1862-1934) svo upp á því, að smástirnið væri bundið við Lagrangepunktinn L4 á braut Júpíters og því væri æskilegt að kanna það svæði nánar og einnig svæðið í kringum L5, og jafnvel tilsvarandi svæði á braut Satúrnusar.
Túlkun Charliers vakti strax mikla athygli og margir stjörnufræðingar brugðust við áskorun hans og beindu sjónaukum sínum að Lagrangepunktunum tveimur. Ekki leið á löngu þar til eitt nýtt smástirni fannst við L5 (Patróklos) og tvö við L4 (Hektor og Nestor). Um svipað leyti var farið að tala um þessa smáhnetti sem Trójusmástirni. Talsverður tími leið þar til þau næstu fundust og árið 1929 voru þau aðeins orðin fjórtán. Í dag skiptir fjöldi skráðra Trójusmástirna þúsundum.
Teikningar úr grein M. Connors, The discovery and naming of Trojan asteroids, frá 2004 (figure 1). Hún á að sýna stöðu allra þekktra Trójusmástirna Júpíters, hinn 1. september árið 2031. Hnitakerfið XYZ hefur upphafspunkt í sólinni, XY er brautarplan Júpíters og Z-ásinn er hornréttur á það. Júpíter er ávallt á Y-ásnum, þ.a. XY planið snýst um Z-ásinn með hornhraða Júpíters, hér „rangsælis“ um sólu. Einingin á ásunum er stjarnfræðieiningin AU. Í þessu hnitakerfi gengur smástirnið Akkilles um Lagrangepunktinn L4. Í kyrrstöðukerfi sólarinnar snýst Akkilles hins vegar um sólina, því sem næst á sömu braut og Júpíter, en að meðaltali u.þ.b. 60 gráður á undan honum. Þetta má kanna betur á stillanlegum myndum á vefsíðu Sky & Telescope, 588 Achilles (sjá undirsíður). Það var þessi braut Akkillesar, sem Steinþór var að glíma við á sínum tíma með aðstoð handsnúinnar reiknivélar og lógariþmataflna.
Meðal þeirra stjörnufræðinga, sem fengu áhuga á Trójusmástirnunum strax í upphafi, voru þeir Elis Strömgren og bandaríski stjörnufræðingurinn, A.O. Leuschner (1868-1953). Prófessor Leuschner er nefndur hér sérstaklega, af þeirri einföldu ástæðu, að hann var leiðbeinandi Vestur-Íslendingsins Sturlu Einarssonar í doktorsnámi hans við Kaliforníuháskóla í Berkeley. Líkt og Strömgren var Leuschner sérfræðingur í aflfræði himintungla með tilheyrandi brautarreikningum og þeir Strömgren áttu í nokkrum samskiptum tengdum því sviði. Leuschner er meðal annars þekktur fyrir sérstaka nálgunaraðferð í brautarreikningum, sem við hann er kennd:
Það var þessi aðferð, sem Sturla notaði í doktorsverkefni sínu til að ákvarða snertibrautir Trójusmástirnanna Hektors og Akkillesar um sólina:
Þótt ég efist um, að það verði nokkurn tímann að veruleika, væri það út af fyrir sig verðugt viðfangsefni fyrir meistaranema í vísindasögu að bera saman aðferðir og niðurstöður þeirra Sturlu og Steinþórs fyrir braut Akkillesar.
Glíma Steinþórs við Akkilles
Viðfangsefni Steinþórs var hluti af stærra verkefni í umsjón þeirra Strömgrens og Vinter Hansens, sem snerist um það að nota þekktar mæliniðurstöður til að reikna ítrekað út fyrri hreyfingar og síðan snertibrautir nokkurra Trjójusmástirna um sólu.Tilgangurinn var fyrst og fremst sá að koma í veg fyrir að hnettirnir týndust, enda eru truflanir á brautunum smástirnanna verulegar vegna nærveru Júpíters og Satúrnusar. Verkefnið tengdist jafnframt tölulegum reikningum Strömgrens og samstarfsmanna hans á lotubundnum lausnum á takmarkaða þriggja hnatta vandamálinu (sjá undirkafla 3.1).
Til að fá örlitla hugmynd um vandamálið, sem Steinþór þurfti að leysa, er gagnlegt að skoða eftirfarandi mynd: ´
Á þessari teikningu frá 1960 er gerð tilraun til að sýna meðalbrautir tveggja dæmigerðra Trójusmástirna Júpíters í viðmiðunarkerfi, þar sem bæði sólin og Júpíter eru í kyrrstöðu (og þar með Lagrangepunktarnir L4 og L5). Takið sérstaklega eftir því, að snúningsstefna smástirnabrautanna er eins um báða punktana. Í kyrrstöðukerfi sólarinnar snýst Júpíter (ásamt L4 og L5) hins vegar „rangsælis“ eftir nær hringlaga sporbaug um sólina (L4 fer fremstur, þá kemur Júpíter og L5 rekur lestina). Samkvæmt þessu eru brautir Trójusmástirnanna um sólina því truflaðir sporbaugar, sem liggja nærri sporbaug Júpíters. Frá Júpíter séð nálgast smástirnin ýmist eða fjarlægjast með sveiflukendum hætti. Sjá nánari umfjöllun í grein S.B. Nicholsons frá 1961: The Trojan Asteroids, þaðan sem myndin er fengin að láni (bls. 4). Athugið að þessi niðurstaða er frá því fyrir tölvuöld. Nýlegir tölvureikningar sýna, að brautir margra Trójusmástirna geta almennt verið talsvert flóknari en hér er sýnt.
Eina leiðin til öðlast sem nákvæmastar upplýsingar um framtíðarhreyfingar smástirna og halastjarna er að safna sem flestum mæligögnum um stöðu þeirra og hraða og beita síðan viðeigandi truflanareikningi. Í verkefni sínu notaði Steinþór vel þekkta reikniaðferð, sem kennd er við þýska stjörnufræðinginn J.F. Encke (1791-1865) til að reikna út braut Akkillesar á tímabilinu 1906 til 1929 og áætla snertibrautina í lok tímabilsins með því að taka tillit til truflana frá Júpíter og Satúrnusi. Við þá vinnu studdist hann einnig að hluta við reikniaðferðir úr bók G. Strackes frá 1929, Bahnbestimmung der Planeten und Kometen.
Við þessa viðamiklu og tímafreku útreikningana notaði Steinþór öll tiltæk mæligögn um Akkilles frá athugunarstöðvum í Evrópu, Bandaríkjunum og Alsír, en í magistersritgerðinni sjálfri (frá 1929) studdist hann aðallega við gögn og greinar Vinter Hansens frá fyrri tíð:
Eftir að hafa fengið magistersritgerðina metna hæfa og lokið öllum prófum í nóvember 1929, hélt Steinþór til Íslands, þar sem hann ætlaði, samhliða öðrum störfum, að ganga frá grein í Astronomische Nachricthen um helstu niðurstöður ritgerðarinnar. Það dróst þó á langinn, því í millitíðinni bárust honum tvær greinar með nýjum upplýsingum, sem hann ákvað í samráði við Strömgren að nota til frekari reikninga og uppfærslu á fyrri niðurstöðum:
Þetta varð til þess, ásamt önnum við kennslu og önnur störf, að lokaútgáfan tafðist um heil þrjú ár:
Hér má svo sjá lokaniðurstöðu Steinþórs fyrir snertibraut Akkillesar:
Snertibraut (= tímabundinn Keplerssporbaugur) Akkillesar í byrjun janúar 1929. Úr grein Steinþórs frá 1933, Über die Bewegung des Planeten der Jupitergruppe 588 Achilles in der Zeit von 1906 bis 1929, bls. 292. Grunnstærðirnar í fremri dálknum eru miðaðar við stöðu vorpunkts í upphafi ársins 1925 (sjá mynd og meðfylgjandi texta í undirkafla 2.1 hér að framan). Í aftari dálknum er a hálfur langás sporbaugsins, μ er meðaltalið af eiginhreyfingu Akkillesar í bogasekúndum á sólarhring og φ er hornið á milli skammáss brautarinnar og línu, sem tengir annan endapunkt hans við stöðu sólar í brennipunkti. Miðskekkja sporbaugsins er svo gefin með jöfnunni e = sinφ = 0,15. – Vegna truflana frá öðrum hnöttum, einkum Júpíter og Satúrnusi og eins vegna pólveltu og pólriðu jarðarinnar, breytast þessar stærðir lítillega með tíma. Hér eru þær miðaðar við stöðuna eins og hún var í ársbyrjun 1925. – Nýjustu tölur má hins vegar finna hér og einnig mynd af snertibrautinni eins og hún er í dag.
Rétt er að geta þess, að þótt áhugi stjörnufræðinga á Trójusmástirnum Júpíters hafi almennt farið minnkandi með árunum eru þau enn til rannsóknar hjá nokkrum öflugum hópum vísindamanna. Þar á meðal eru einstaklingar, sem rannsaka ringl í sólkerfinu og einnig þeir, sem leita að vísbendingum um uppruna sólkerfisins og þróun þess. Í því sambandi má nefna, að þegar þessi orð eru rituð er geimfarið Lucy á leið til smástirnaþyrpingarinnar við L4. Geimfarinu var skotið á loft árið 2021 og áætlað er að það verði við L4 haustið 2027. Eftir stutta dvöl þar heldur Lucy til baka framhjá jörðinni og yfir til L5 þar sem áætluð koma er árið 2033. Hér má sjá stutt kynningarmyndband um þennan langa vísindaleiðangur.
Að lokum er hér til fróðleiks ljósrit af upplýsingum um magisterspróf Steinþórs í stjörnufræði, sem birtar voru í Árbók Kaupmannahafnarháskóla 1929-1930:
Úr Aarbog for Københavns Universitet 1929-1930, bls. 92-93.
Steinþór Sigurðsson magister fluttist hingað alkominn í árslok 1929 og hafði þá þegar fengið kennarastöðu í stærðfræði og eðlisfræði við Gagnfræðaskólann á Akureyri, sem um það leyti var verið að breyta í Menntaskóla (MA). Þar kom hann í stað Pálma Hannessonar (1898-1956), sem fluttst hafði suður til að taka við embætti rektors við Menntaskólann í Reykjavík.
Fjallað var um lokapróf og heimkomu Steinþórs í flestum íslenskum dagblöðum: Myndin sýnir tvö dæmi um fréttafluttninginn. – Til vinstri: Frétt í Vísi, 20. nóv. 1929, bls. 3. – Til hægri: Frétt í Degi, 7. des 1929, bls. 206.
Sigurkarl Stefánsson getur þess í minningargrein sinni í TVFÍ, að við námslok haustið 1929 hafi Steinþóri gefist kostur á fastri stöðu sem stjörnufræðingur erlendis, sem hann þáði ekki. Það fylgdi þó ekki sögunni, hvar það hafi verið. Sigurður, sonur Steinþórs, segist hins vegar hafa heyrt það í æsku, að umrædd staða hafi verið í Hollandi. Sigurkarl greinir einnig frá því, að Danir hafi þá boðist til þess „að hér heima yrði komið á fót obseratorii Íslendingum að kostnaðarlausu, ef Steinþór veitti þvi forstöðu og rekstur þess yrði kostaður af ríkissjóði Íslands“. Steinþór mun hafa talið slíkar hugmyndir óraunhæfar og kom þeim aldrei á framfæri við stjórnvöld hér heima.
Í þessu sambandi má til gamans nefna, að árið 1929 voru liðin 124 ár frá því Vísindafélagið danska lagði niður stjörnuathugunarstöðina að Lambhúsum við Bessastaði. Jafnframt, að heil 68 ár áttu enn eftir að líða frá heimkomu Steinþórs og þar til Íslendingar fengu loks aðild að Norræna stjörnusjónaukanum á La Palma, og þá eftir sjö ára baráttu íslenskra stjarnvísindamanna við eigin yfirvöld.
Þrátt fyrir að halda áfram að búa niðurstöður sínar úr magistersverkefninu undir prentun í Astronomische Nachrichten, virðist Steinþór fljótlega hafa sagt skilið við stjörnufræðina og í staðinn snúið sér að öðrum viðfangsefnum. Auk kennslu við Menntaskólann á Akureyri (1930-1935), Menntaskólann í Reykjavík (frá 1935), Verkfræðideild Háskóla Íslands (frá 1940) og fleiri opinberra starfa, bar þar einna helst á náttúrurannsóknum af ýmsu tagi. Hér má meðal annars nefna landmælingar, landkönnun og jarðfræði, þar á meðal jökla- og jarðhitarannsóknir sem og vangaveltur í líffræði. Svipmyndum af þessum viðfangsefnum hans verður brugðið upp í næsta hluta, færslunni 4a2.
Í lokin er rétt að geta þess, að eftir að heim var komið skrifaði Steinþór nokkrar alþýðlegar greinar, meðal annars um vísindaleg efni (sjá nánar í ritaskrá hans), auk þess að vera tíður gestur í hinu þá tiltölulega nýtilkomnu útvarpi landsmanna. Þar á meðal voru þrjár greinar um stjarnvísindaleg efni og tvær, sem hann birti í minningu forvera síns, Björns Gunnlaugssonar:
Landkönnuðurinn Steinþór Sigurðsson á Vatnajökli árið 1946. Við vinstri fót hans grillir í skíði, en sem kunnugt er var Steinþór einn af frumherjum skíðaíþrótta á Íslandi. Myndin er hluti af annarri stærri, sem birtist í Morgunblaðsgreininni, Foringi var fallinn, 2. mars, árið 2000.
* Stjarneðlisfræði og heimsfræði á Íslandi: Efnisyfirlit *
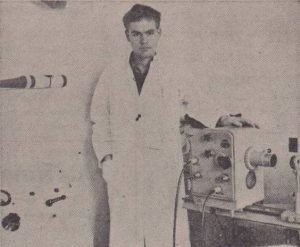
Magnús Magnússon var orðinn rúmlega fertugur, þegar ég hitti hann fyrst. Það var á kynningarfundi fyrir nýnema í Verkfræðideild Háskóla Íslands, haustið 1967. Hann mun þá hafa verið fráfarandi deildarforseti og sem slíkur fræddi hann okkur um þær fáu námsleiðir sem í boði voru við deildina á þeim tíma. Hann kom mér fyrir sjónir sem vingjarnlegur og geðþekkur maður og ég fann strax fyrir þeim velvilja í garð stúdenta, sem ég átti eftir að kynnast mun betur síðar.
Sem aðstoðarmaður og síðar sumarstúdent við Raunvísindastofnun Háskólans á árunum 1968 til 1970 hitti ég Magnús nokkrum sinnum á göngunum. Samskipti okkar voru þó ekki mikil í þau skipti, enda var hann þá forstöðumaður stofnunarinnar og sífellt á þönum. Síðar átti ég þó því láni að fagna að njóta kennslu hans og að lokum verða samkennari hans við Eðlisfræðiskor Raunvísindadeildar Háskólans (nú kölluð námsbraut í eðlisfræði, að mér skilst). Þá fyrst fékk ég að kynnast fyrir alvöru víðfeðmri þekkingu hans, skarpskyggni og víðsýni.
Þar til nýlega hafði ég ekki áttað mig fyllilega á því mikla og mikilvæga starfi, sem Magnús vann með Þorbirni Sigurgeirssyni og öðrum í aðdragandanum að stofnun Raunvísinda-stofnunar árið 1966. Annars vegar var um að ræða könnun og almenna kynningu á kjarnorkumálum á árunum 1955 til 1964 og hins vegar formlegan undirbúning að fastri rannsóknaraðstöðu í eðlisvísindum og stærðfræði við Háskóla Íslands, vinnu sem endaði með því, að draumurinn um Raunvísindastofnun Háskólans varð að veruleika.
Þrátt fyrir, að Magnús hafi sjálfur skrifað heilmikið um þessa merku sögu og eigin þátttöku í þróun mála, gætir fulls hlutleysis í skilmerkilegum frásögnum hans. Ég varð heldur aldrei var við, að hann flíkaði sérstaklega framlagi sínu eða eigin ágæti, hvort heldur var í samræðum við samstarfsmenn eða á opinberum vettvangi. Hógværð og kurteisi virtust honum í blóð borin.
Eins og sjá má í lok þessarar minningargreinar, á ég Magnúsi persónulega mikið að þakka. En áður en ég fjalla nánar um þau atriði, langar mig til að heiðra minningu þessa kennara míns og samstarfsmanns með því að bregða upp svipmyndum af þeim þáttum úr námsferli hans og starfsævi, sem ég þekki best og mér þykja hvað áhugaverðastir. Ég treysti því, að aðrir samstarfsmenn hans, vinir og vandamenn, taki að sér að fylla í eyðurnar.
Magnús stundaði nám við Menntaskólann í Reykjavík (MR) á stríðsárunum. Hann þótti framúrskarandi námsmaður og lauk stúdentsprófi frá stærðfræðideild sem dúx skólans, vorið 1945.
Magnús, fyrir miðri mynd, við verklegar æfingar í efnafræði í 5. bekk MR veturinn 1943-1944. Kennarinn, Sigurkarl Stefánsson stærðfræðingur, er annar frá hægri. Á stríðsárunum var Sigurkarl aðalkennari skólans í stærðfræði, stjörnufræði, eðlisfræði og efnafræði. Steinþór Sigurðsson stjörnufræðingur kenndi þar einnig stærðfræði á árunum 1941-1945, en hvort hann kenndi Magnúsi veit ég ekki. Myndin er úr Skólablaðinu, 1. apríl 1944, bls. 40.
Málfundur á vegum nemendafélagsins Framtíðarinnar í MR árið 1944. Fundarstjórinn, Magnús Magnússon, hallar sér aftur í sæti sínu, en hann var jafnframt formaður félagsins það ár. Mynd úr Skólablaðinu, 1. apríl 1944, bls. 47.
Góður árangur á stúdentsprófi hefur án efa tryggt Magnúsi skólavist við hinn þekkta Cambridgeháskóla í Englandi, haustið 1945. Þaðan lauk hann B.A. prófi í eðlisfræði og stærðfræði árið 1949 og síðan M.A. prófi 1952.
Ég hef því miður litlar upplýsingar um Cambridgedvöl Magnúsar, en fyrir nokkrum árum sagði hann mér þó, að í meistaranáminu hefði hann fyrst og fremst lagt stund á skammta-efnafræði. Sennilega hefur það verið í tengslum við rannsóknarhóp efnafræðingsins snjalla, S.F. Boys, þótt ég viti það ekki með vissu. Allavega notaði Magnús EDSAC I tölvuna frægu við reikninga á bylgjuföllum á árunum 1950-1953. Hann varð þannig sá Íslendingur, sem einna fyrstur kynntist tölvum og tölvureikningum. Eins og síðar verður komið að, varð hann í kjölfarið einn af frumkvöðlunum á því sviði við Háskóla Íslands.
EDSAC I tölvan í Cambridge, nýkomin í gagnið, árið 1949. Magnús notaði hana við tölulega reikninga á bylgjuföllum í skammtaefnafræði á árunum 1950-1953. Mynd: Wikipedia.
Þegar litið er til baka má sjá, að tímabilið frá 1953 til 1955 átti eftir að hafa mikil áhrif á framtíð Magnúsar sem eðlisfræðings hér á landi. Kjarneðlisfræðin var þá þegar orðin ein helsta tískugreinin í raunvísindum og kjarnorkumál og kjarnorkuógnin voru ofarlega í huga alls almennings. Ástæðan var kalda stríðið, kjarnorkuvopnakapphlaup stórveldanna og geislavirkni af völdum tilraunasprenginga í andrúmslofti.
Við þessar aðstæður flutti Magnús ásamt konu sinni, Helgu, og kornungum syni, Kjartani, frá Cambridge til Íslands sumarið 1953 og tók að sér að kenna annars og þriðja árs eðlisfræði við Verkfræðideild Háskólans, skólaárið 1953-1954.
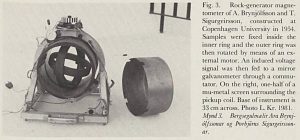
Svo merkilega vildi til, að sumarið 1953 kom Þorbjörn Sigurgeirsson einnig heim aftur, eftir árangursríka ársdvöl hjá CERN í Kaupmannahöfn. Ekki er að efa, að þeir Magnús hafi fljótlega mælt sér mót, meðal annars til að ræða kjarnorkumálin og framtíðarmöguleika eðlisfræðinnar á Íslandi. Í þessu sambandi má minna á, að í desember 1953 flutti Eisenhower Bandaríkjaforseti hina frægu Atoms for Peace ræðu á Allsherjarþingi Sameinuðu þjóðanna. Málfluttningur hans vakti strax miklar væntingar víða um heim, þar á meðal hér á landi, um betri tíma, byggða á friðsamlegri notkun kjarnorkunnar.
Um það leyti sem annað barn þeirra hjóna, Magnús Már, kom í heiminn sumarið 1954, fór Magnús með fjölskyldu sína til ársdvalar við Princetonháskóla. Þar mun hann hafa kynnt sér kjarneðlisfræði og hina almennu afstæðiskenningu Einsteins. Fyrir það, sem á eftir fer, er rétt að hafa í huga, að skömmu fyrir jólin 1954 náðu aðildarríki Sameinuðu þjóðanna loks samkomulagi um það, að haldin skyldi alþjóðleg ráðstefna um friðsamlega notkun kjarnorkunnar, haustið 1955.
Eftir námsdvölina í Princeton, flutti Magnús aftur heim með fjölskylduna og enn tók hann að sér að kenna annars og þriðja árs eðlisfræði við Verkfræðideildina, veturinn 1955 til 1956. Að auki kenndi hann þriðja árs nemum stærðfræði og kom einnig við í MR, þar sem hann kenndi bæði eðlisfræði og efnafræði. Það er jafnframt haustið 1955, sem hann fer að vinna að kjanorkumálum hér á landi ásamt Þorbirni Sigurgeirssyni og fleirum. Í þeirri baráttu miðri fæddist þriðja og síðasta barn þeirra hjóna, Margrét Þorbjörg.
Hér verður farið hratt yfir sögu, þar sem ég hef nýlega skrifað þrjár greinar um efnið, með talsverða áherslu á framlag þeirra Þorbjörns og Magnúsar:
Magnús benti okkur yngri mönnunum oftar en einu sinni á það, að Genfarfundurinn um friðsamlega notkun kjarnorkunnar í ágúst 1955 hefði markað ákveðin þáttaskil í sögu eðlisfræðinnar á Íslandi. Íslensku fulltrúarnir, þeir Þorbjörn og Magnús, komu heim af ráðstefnunni innblásnir af nýjum hugmyndum og með tvær tilbúnar tillögur í farteskinu um æskileg viðfangsefni.
Þorbjörn (til vinstri) og Magnús um það leyti, sem þeir sóttu Genfarráðstefnuna miklu í ágúst 1955, ásamt Kristjáni Albertssyni sendiráðunauti Íslands í París. Sjá nánari umfjöllun í viðtali við þá í Þjóðviljanum, 1. september 1955.
Tillögur þeirra félaga voru þessar: (1) „Að komið verði á fót rannsóknastofu, sem hafi með höndum geislamælingar og forgöngu og eftirlit með notkun geislavirkra efna í þágu læknavísinda og atvinnuvega“ og (2) „Að strax verði gerðar ráðstafanir til rækilegrar athugunar á möguleikunum til framleiðslu þungs vatns á Íslandi með notkun jarðhita.“
Báðar tillögurnar urðu að veruleika. Sú fyrri leiddi til þess, tveimur árum síðar, að Þorbjörn Sigurgeirsson var skipaður fyrsti prófessorinn í eðlisfræði við Verkfræðideild Háskóla Íslands og Eðlisfræðistofnun Háskólans var sett á laggirnar, nær samtímis. Löngu seinna lét Magnús þau orð falla, að Eðlisfræðistofnunin hefði verið „sá voldugi vísir, er Raunvísindastofnun óx upp af“.
Seinni tillagan leiddi strax í september 1955 til fundarhalda og umræðna um stofnun sérstakrar nefndar til að sjá um kjarnorkumál hér á landi. Voru þeir Þorbjörn og Magnús manna duglegastir að boða fagnaðarerindið á margskonar samkomum og í rituðu máli og viðtölum. Í janúar 1956 var Kjarnfræðanefnd Íslands svo stofnuð, með dyggum stuðningi flestra, ef ekki allra, þeirra íslensku stofnana, sem á þeim tíma komu með einhverjum hætti að tækni og raunvísindum.
Fljótlega var ákveðið að helstu umfjöllunaratriði nefndarinnar yrðu þungavatnsfram-leiðsla, orkumál, heilbrigðismál, landbúnaðarmál, iðnaðarmál og stofnun rannsóknastofu í geislamælingum. Frekari umfjöllun um þessi mál er að finna í ítarlegri grein Magnúsar um störf nefndarinnar, en hann var framkvæmdastjóri hennar árin 1956-1958 og aftur 1960-1961:
Fyrsta verkefni Kjarnfræðanefndarinnar var að hafa umsjón með fjölsóttri bandarískri farandsýningu, sem haldin var í Listamannaskálanum í febrúar 1956. Af því tilefni þýddu þeir Magnús og Þorbjörn fróðlegan bækling:
Sýningin hafði tilætluð áhrif og almenningur, ekki síst unga fólkið, sýndi henni mikinn áhuga. Mér þykir sennilegt, að hún hafi átt sinn þátt í að fjölga þeim íslensku námsmönnum, sem hófu nám í eðlisvísindum og stærðfræði á næstu árum og áratugum. Fleira kom þó til, einkum upphaf geimaldar með Spútnikævintýrinu 1957 og í kjölfarið stóraukin áhersla erlendis á raunvísindi.
Eins og fram kemur í fyrrnefndri grein Magnúsar, lagði Kjarnfræðanefndin, og ekki síst Magnús sjálfur, gífurlega vinnu í þungavatnsmálið svokallaða, en það var ítarleg könnun nefndarinnar og samstarfsmanna hennar á því, hvort arðbært væri að nota hér jarðhita til að framleiða þungt vatn til útflutnings. Niðurstaðan varð á endanum sú, að svo væri ekki, og mun ástæðan fyrst og fremst hafa verið óhagstæð þróun þungavatnsmála erlendis. En það gerðist ekki fyrr en nokkrum árum eftir að hópur erlendra kjarnorkusérfræðinga kom hingað á vegum Efnahagsstofnunar Evrópu (OEEC) vorið 1958 og taldi aðstæður til þungavatnsframleiðslu mjög hagstæðar hér á landi.
Hér má sjá erlendu kjarnorkufræðingana, sem hingað komu í apríl 1958 á vegum OEEC, ásamt íslenskum vísindamönnum. Frá vinstri: Magnús Magnússon framkvæmdastjóri Kjarnfræðanefndar, P. I. Walker (Harwell), L. Kowarski (CERN), P. Frank, aðstoðarmadur hans, C. W. Hart-Jones (Harwell), Guðmundur Pálmason verkfræðingur, G. Weiss (Pintsch-Bamag AG) og Gunnar Böðvarsson forstöðumadur Jarðborana ríkisins. Sjá nánar í grein í Mbl, 1. maí 1958: Sérfræðingar frá O.E.E.C. telja aðstæður hagstæðar til þungavatnsframleiðslu hér.
Norræna samskiptanefndin um kjarnorkumál (Nordisk Kontaktorgan for Atomenergispørgs-mål) hélt fund hér á landi sumarið 1967. Fundarmenn skruppu einnig í skoðunarferð til Þingvalla, þar sem myndin var tekin. Þarna þykist ég sjá Magnús Magnússon (fyrir miðri mynd, í tiltölulega ljósum frakka og með hatt) og Þorbjörn Sigurgeirsson (annan frá hægri). Á myndinni mun einnig mega þekkja (frá vinstri) Ilkka Mäkipentti (Finnlandi), Knut Gussgard (Noregi), Erkki Laurila (Finnlandi), Gert Vigh (Danmörku), Harry Brynielsson (Svíþjóð), Jens Chr. Hauge (Noregi), Hans v. Bülow (Danmörku) og Odd Gøthe (Noregi). Myndin er úr bókinni Half a Century of Nordic Nuclear co-operation: An Insider's Recollections, (1997) eftir F.R. Marcus, bls. 52. --- Magnús var jafnframt lengi fulltrúi Íslands í Stjórnarnefnd kjarnorkumála (Steering Committee for Nuclear Energy) hjá OECD og sömuleiðis hjá Alþjóða kjarnorkumálastofnuninni (International Atomic Energy Agency) í Vínarborg. Eftir að Kjarnfræðanefndin lagði sjálfa sig niður, árið 1964, varð Magnús ráðgjafi ríkisstjórnarinnar í kjarnorkumálum.
Greinar MM um kjarnorkumál:
Vorið 1958 var Magnús búinn að vinna baki brotnu sem framkvæmdastjóri Kjarnfræða-nefndarinnar í tæp tvö ár, auk þess sem hann hafði tekið að sér að vera fulltrúi Íslands í ýmsum norrænum og alþjóðlegum nefndum um kjarnorkumál. Þótt ekki hefði allt gengið samkvæmt upphaflegum áætlunum Kjarnfræðanefndar, virtust málin þó þokast í rétta átt. Með sameiginlegu átaki nefndarmanna og annarra hafði til dæmis tekist að koma rannsóknum í eðlisfræði í bærilegt skjól innan veggja Háskóla Íslands og eftir nýlega heimsókn OEEC hópsins virtist þungavatnsmálið loks við það að komast í réttan farveg.
Magnús mun því hafa farið að líta í kringum sig eftir nýjum tækifærum. Það endaði með því, að hann fékk styrk til að stunda rannsóknir í tvö ár við Norrænu stofnunina í kennilegri eðlisfræði (Nordita) við Blegdamsvej í Kaupmannahöfn. Þangað fór hann ásamt konu sinni og þremur börnum, haustið 1958.
Nordita hafði verið sett á laggirnar árið áður með þátttöku allra Norðurlandanna fimm. Þorbjörn var fulltrúi Íslands í stjórn stofnunarinnar frá upphafi til 1973, og Magnús var fyrsti íslenski styrkþeginn.
Þegar til Danmerkur kom, vildi svo til, að fjölskyldan fékk húsnæði við götu, þar sem kjarneðlisfræðingurinn Aage Bohr bjó. Aage var sonur Níelsar Bohr og þá nýorðinn prófessor í kennilegri eðlisfræði við Kaupmannahafnarháskóla. Hann átti þrjú börn eins og Magnús og á svipuðum aldri. Börnin léku sér talsvert saman og milli fjölskyldnanna myndaðist ævilöng vinátta. Þetta kom sér meðal annars vel áratugum síðar, þegar þeir Magnús og Aage sátu saman í stjórn Nordita.
Magnús hafði lengi haft mikinn áhuga á almennu afstæðiskenningunni og svo vel vildi til, að forstöðumaður Nordita og prófessor í kennilegri eðlisfræði við Kaupmannahafnar-háskóla, Christian Møller, hafði þá nokkrum árum fyrr hafið kennilegar rannsóknir á þyngdaraflinu. Hann hafði meira að segja gefið út þekkta kennslubók um efnið árið 1952, The Theory of Relativity, sem var víða notuð í háskólum í Evrópu og Bandaríkjunum.
Frá vinstri: Aage Bohr árið 1955 og Christian Møller árið 1971. Myndir: Wikipedia.
Þegar Magnús kom til Nordita, árið 1958, var Møller mjög upptekinn af vandamálinu um þyngdarorku í almennu afstæðiskenningunni. Þannig er, að svokallaður orku-skriðþunga þinur, sem gegnir lykilhlutverki í sviðsjöfnum Einsteins, tekur ekkert tillit til orkunnar, sem fólgin er í sveigðu tímarúmi (þyngdarsviðinu) og lýsir aðeins orkuástandi efnisins. Ein afleiðingin er sú, að kenningin getur ekki ákvarðað hvar í rúminu þyngdarorkan er staðsett. Ýmsir höfðu reynt að leysa þetta vandamál, þar á meðal Einstein sjálfur og þeir félagarnir Landau og Lifshitz, en án fullnægjandi árangurs. Þetta fannst Møller ótækt og árið 1958 kynnti hann til sögunnar svokallaðan orku-skriðþunga komplex, sem hann taldi geta leyst vandann.
Eftir nokkar viðræður við Møller tók Magnús að sér að kanna, hvort þessi komplex prófessorsins væri ótvírætt ákvarðaður í skilningi stærðfræðinnar. Eftir talsverða vinnu tókst honum að sýna fram á að svo væri, að gefnum tveimur tiltölulega vægum skilyrðum. Um þetta skrifaði hann ágæta grein, sem Vísindafélagið danska gaf út 1960, skömmu áður en fjölskyldan flutti aftur heim til Íslands:
Grein Magnúsar um orku-skriðþunga komplex Møllers: Further Properties of the Energy-Momentum Complex in General relativity.
Rétt er að geta þess, að hugmynd Møllers um orku-skriðþunga komplexinn hefur aldrei átt miklu fylgi að fagna meðal sérfræðinga í almennu afstæðiskenningunni. Enn í dag má þó sjá stöku grein um þessa gömlu kenningu hans í sérhæfðum fagtímaritum.
Hópmynd af starfsmönnum og gestum Nordita og NBI, tekin í nóvember 1959. Magnús situr í fremstu röð, annar frá hægri. Í sömu röð má sjá þá Ben Mottelsson (með skegg) og Aage Bohr (3. og 4. frá vinstri), Felix Bloch og Niels Bohr (8. og 9. frá vinstri) og Leon Rosenfeld (12. frá vinstri). Í efstu röð stendur Sheldon Glashow (hávaxinn og með gleraugu), níundi frá vinstri. Á myndina vantar Christian Møller. Ljósmynd: Niels Bohr Archive.
Grein Magnúsar um dvölina hjá Nordita:
Skömmu eftir komuna frá Kaupmannahöfn, árið 1960, var Magnús skipaður prófessor í eðlisfræði við Verkfræðideild Háskóla Íslands og tók þá aftur upp samvinnu við Þorbjörn Sigurgeirsson. Hann sinnti lengi mikilli kennslu, samhliða ýmsum nefndar- og stjórnunarstörfum, bæði innan Háskólans sem utan. Þá var hann forseti Verkfræðideildar 1965-1967 og Verkfræði- og raunvísindadeildar 1971-1973. Lögum samkvæmt fór hann sjötugur á eftirlaun, en var lengi eftir það með starfsaðstöðu á skrifstofu sinni í VR II.
Eins og áður sagði, hafði Magnús framkvæmt reikninga á bylgjuföllum í skammtaefna-fræði með EDSAC I tölvunni við Cambridgeháskóla á árunum 1950 til 1953. Einnig hafði hann haft nokkra viðkomu hjá Regnecentralen í Kaupmannahöfn á Norditaárunum.
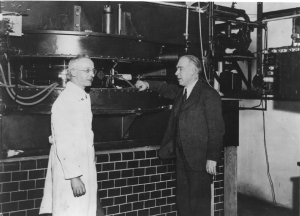
Vegna þessarar reynslu sinnar af tölvum og tölvureikningum, var Magnús fenginn til þess að hafa yfirumsjón með kaupum á fyrstu tölvu Háskólans, IBM 1620, og sjá um rekstur hennar í kjallara nýbyggingarinnar á Dunhaga 3. Þar hafði henni verið komið fyrir síðla árs 1964. Um sama leyti var Reiknistofnun Háskólans komið á fót og Magnús ráðinn sem forstöðumaður hennar. Því starfi sinnti hann til ársins 1972.
Hér er verið að taka innpakkaða IBM 1620 tölvu Háskólans af bílpalli og koma henni fyrir í kjallara nýbyggingar Raunvísindastofnunar við Dunhaga í október 1964. Á myndinni er Magnús Magnússon eini maðurinn, sem snýr andlitinu að myndavélinni. Ljósmynd: Þorsteinn Sæmundsson.
IBM 1620 tölva Háskólans tilbúin til notkunar í desember 1964. Á myndinni eru frá vinstri: Helgi Sigvaldason, Ragnar Ingimarsson, Oddur Benediktsson og Magnús.
Greinar Magnúsar um tölvur:
Fyrir rúmum sextíu árum var fyrsta skóflustungan tekin að húsi Raunvísindastofnunar að Dunhaga 3. Stofnunin var svo formlega opnuð, haustið 1966, þótt ýmis rannsóknarstrarf-semi hefði þá þegar verið stunduð um skeið í byggingunni. Magnús var skipaður forstjóri og sinnti hann því starfi í tíu ár, 1966-1976. Hann lét stundum þau orð falla, að eðlilegra hefði verið, að Þorbjörn yrði fyrsti skipstjórinn. Þorbjörn baðst hins vegar eindregið undan því á sínum tíma og tilnefndi Magnús í staðinn.
Sem flaggskip raunvísinda á Íslandi, á Raunvísindastofnun sér mikla og áhugverða sögu. Enn sem komið er, hefur sú saga þó aðeins verið skráð að hluta, því miður. Hún verður heldur ekki sögð hér, en í sárabætur birti ég örfáar svipmyndir frá síðustu sextíu árum. Þær eiga það sameiginlegt að tengjast allar Magnúsi heitnum með einum eða öðrum hætti.
Fyrsta skóflustungan tekin að Dunhagahúsinu í janúar 1964. Þriðji frá vinstri er Magnús (með hatt og í síðum ljósum frakka) að spjalla við þá Leif Ásgeirsson prófessor og dr. Gunnar Böðvarsson (falinn á bak við Gunnar er Páll Theodórsson eðlisfræðingur). Hægra megin við Magnús á standa þeir Loftur Þorsteinsson prófessor, Guðmundur K. Guðmundsson tryggingafræðingur, Steingrímur Jónsson fyrrum rafmagnsstjóri, óþekktur, Ármann Snævarr háskólarektor, J.K. Penfield sendiherra Bandaríkjanna, Þorbjörn Sigurgeirsson prófessor, óþekkur, Bragi Árnason efnafræðingur, Skarphéðinn Jóhannsson arkitekt, Jóhannes Zoëga hitaveitustjóri og Trausti Einarsson prófessor. Sjá einnig greinina Bygging Raunvísindastofnunar hafin í Vísi, 21. Janúar 1964.
Ármann Snævarr háskólarektor afhendir Magnúsi, forstjóra Raunvísindastofnunar, Dunhagahúsið til yfirráða, 14. október 1966. Sjá nánar í umfjöllun Mbl, daginn eftir.
Fyrsta stjórn Raunvísindastofnunar í fundaherberginu á Dunhaga 3, árið 1967. Frá vinstri: Steingrímur Baldursson efnafræðistofu, Þorbjörn Sigurgeirsson eðlisfræðistofu, Magnús Magnússon forstjóri, Þorsteinn Sæmundsson jarðeðlisfræðistofu og Leifur Ásgeirsson stærðfræðistofu.
Raunvísindastofnun Háskólans í október 1968.
Elísabet Guðjohnsen skrifstofustjóri og síðar framkvæmdastjóri Raunvísindastofnunar. Hún var helsta stoð og stytta forstöðumanna stofnunarinnar í áratugi, fyrst Magnúsar og síðan eftirmanna hans.
Nokkrir þátttakendur í Þórsmerkurferð Raunvísindastofnunar sumarið 1968. Á myndinni eru meðal annars (frá vinstri): Leó Kristjánsson (liggjandi) og Óskar Ágústsson (standandi). Sitjandi eru Jakob Yngvason, og Guðrún Kvaran (skrifandi í gestabók). Að baki Guðrúnar situr Loftur Þorsteinsson og lengra til hægri sitja í röð þau Guðrún Hannesdóttir, Inga Hersteinsdóttir, Margrét ellefu ára gömul dóttir Magnúsar og loks Magnús sjálfur. Lengst til hægri sést svo tæpur helmingurinn af Hjálmari Sveinssyni. Aðra á myndinni þekki ég ekki. Þetta er hluti af stærri ljósmynd, sem Þorsteinn Sæmundsson tók.
Magnús á Keflavíkurflugvelli í hópi vaskra manna eftir segulmælingaflug norður fyrir land, árið 1973. Hægra megin við Magnús eru leiðangursstjórinn Charles Gunn, Jón St. Ingason, Marteinn Sverrisson, Einar Pálsson, Páll Theodórsson, Leó Kristjánsson og Stefán Sæmundsson. Mælingarnar voru á vegum jarðsegulrannsóknadeildar bandaríska sjóhersins. Sjá nánar á bls. 221-222 í grein Leós Kristjánssonar, „Segulsviðsmælingar frá flugvélum og skipum við Ísland“, í bókinni Í hlutarins eðli: Afmælisrit til heiðurs Þorbirni Sigurgeirssyni prófessor, bls. 209-225.
Magnús innan um nokkra starfsmenn Raunvísinadastofnunar á efri hæð Dunhagahússins, árið 2003. Frá vinstri: Eggert Briem stærðfræðingur, Bragi Árnason efnafræðingur, Edda Benediktsdóttir lífefnafræðingur, Magnús, Þorkell Helgason stærðfræðingur, Már Björgvinsson efnafræðingur og Hermann Þórisson stærðfræðingur. Ljósmynd: Þorsteinn Sæmundsson.
Greinar Magnúsar um Raunvísindastofnun:
Þegar Þorbjörn Sigurgeirsson hætti, árið 1973, eftir 16 ára setu sem fulltrúi Íslands í stjórn Nordita, lá beint við að Magnús tæki við keflinu. Líkt og Þorbjörn, sinnti hann fulltrúa-starfinu í 16 ár, eða þar til reglugerð um stofnunina var breytt, árið 1989, og nýir menn tóku við.
Mér er kunnugt um, að Magnús var ákaflega vel liðinn meðal starfsmannanna á Blegdamsvej, sem og stjórnarfulltrúa hinna Norðurlandanna, enda átti hann marga vini í þeirra hópi. Hann þótti og góður talsmaður íslenskra eðlisfræðinga.
Hér heima var Magnús duglegur við að kynna starfsemi Nordita fyrir ungum íslenskum eðlisfræðingum og eðlisfræðinemum. Meðal annars hvatti hann þá til að sækja þar kynningarnámskeið og ráðstefnur. Einnig notaði hann oft þjónustu, sem Nordita bauð uppá, Íslendingum að kostnaðarlausu, að senda hingað framúrskarandi eðlisfræðinga til fyrirlestrahalds. Þar var bæði um að ræða vísindamenn stofnunarinnar sjálfrar og gesti þeirra.
Ég held það megi fullyrða, að þessar heimsóknir hafi haft tilætluð áhrif, nefnilega þau að kynna merk erlend rannsóknarverkefni fyrir mönnum hér í fásinninu og jafnframt að hvetja unga íslenska eðlisfræðinema til dáða. Sjálfur get ég nefnt, að fyrirlestrar þeirra Christians Møller (1972), Stirlings Colgate (1973), Gordons Baym (1975 og 1976), Chris Pethick (1976), Dons Lamb (1976) og Bengts Strömgren (1978), höfðu veruleg áhrif á mig og sýn mína á eðlisfræðirannsóknir.
Magnús á stjórnarfundi Nordita haustið 1977. Á myndinni eru, talið réttsælis frá neðra horninu hægra megin: H. Højgaard Jensen, K.-G. Fogel, P. Jauho, P. Tarjanne, A. Kallio, C. Cronström, S. Lundqvist, Magnús Magnússon (dökkklæddur, fyrir miðjum enda borðsins), J. Hansteen, M. Kolsrud, H. Olsen, L. Hulthén, Aa. Bohr, N.R. Nilsson skrifstofustjóri og L. Nielsen ritari. Mynd úr bókinni Nordita – The Copenhagen Years: A Scrapbook, bls. 32.
Magnús og leiðbeinandi minn í doktorsnámi, Chris Pethick prófessor við Nordita, slaka á að loknum fundarhöldum á vegum stofnunarinnar, vorið 1981. Myndin er tekin á þáverandi heimili mínu í Kaupmannahöfn.
Magnús í góðra vina hópi á Nordita-Landau ráðstefnunni, sumarið 1988. Með honum á myndinni eru frá vinstri: Nils Robert Nilsson, Alpo Kallio og Aage Winther. Mynd úr bókinni Nordita – The Copenhagen Years: A Scrapbook, bls. 36.
Grein Magnúsar um Nordita:
Eins og minnst var á í upphafi, kynntist ég Magnúsi fyrst fyrir alvöru, þegar ég tók hjá honum námskeið í aflfræðigreiningu, haustið 1972. Ég var þá í þeim hugleiðingum að fara til Bandaríkjanna í meistaranám í eðlisfræði og hafði ákveðið að ljúka áður BS-prófi frá Háskóla Íslands. Það var reyndar talsverð fyrirhöfn, því ég var í fullu kennarastarfi við Menntaskólann í Reykjavík.
Ásamt nokkrum öðrum námskeiðum taldi ég rétt að taka einnig svokallað sérverkefni við eðlisfræðiskor (nú líklega kallað BS-verkefni) og leitaði til Magnúsar í því sambandi í lok vormisseris 1973. Magnús tók mér vel og það var ákveðið að ég ynni verkefnið þá um sumarið, enda var ég þegar búinn að fá skólavist í Bandaríkjunum, og námið þar hófst í september. Ég man vel, að mér þótti það sérstaklega eftirtektarvert, að Magnús skyldi líta á það sem sjálfsagðan hlut að sinna mér að sumarlagi, þegar allir aðrir prófessorar voru fást við eitthvað annað en kennslu.
Eftir stutt spjall, teygði Magnús sig upp í hillu og dró fram vélritaðan blaðabunka og sagði þetta vera forprent að grein um tilvist svarthola, „Do Black Holes Exist in Nature?“, eftir Christian Møller. Hann hefði skilið ritsmíðina eftir í heimsókn sinni hingað, haustið 1972, og væri í vandræðum með að koma henni á prent. Verkefni mitt væri að lesa þessa óútgefnu grein og skrifa um hana ritgerð.
Ég maldaði örlítið í móinn og kvaðst ekkert kunna í almennu afstæðiskenningunni, þótt ég hefði að sjálfsögðu áhuga á henni. Magnús sagði það lítið mál, mér nægði að byrja á því að lesa kafla 10 til 12 í bókinni The Classical Theory of Fields eftir Landau og Lifshitz til að geta skilið grein Møllers. Þetta gerði ég og skilaði ritgerðinni á tilsettum tíma. Það er því Magnúsi að þakka, að ég fór að líta á þyngdarfræði Einsteins sem viðráðanlegt viðfangsefni fyrir venjulegan íslenskan eðlisfræðing.
Eftir að ég kom heim aftur, í árslok 1974, nú með MS-skírteini í farteskinu, endurnýjaði ég sambandið við Magnús og við hittumst reglulega, meðal annars í tengslum við áðurnefndar heimsóknir erlendu eðlisfræðinganna, sem hingað komu á vegum Nordita. Í hvert skipti, kynnti hann mig fyrir þessum merku mönnum og sá til þess, að ég fengi tækifæri til að ræða við þá um eðlisfræði, venjulega yfir kaffibolla eða í hádegis- og kvöldverðarboðum.
Á þessum tíma hélt hann mér, og öðrum ungum eðlisfræðingum hér heima, reglulega upplýstum um þróun helstu mála við Nordita og hvatti okkur til að fara þangað á ráðstefnur og námskeið. Fyrir hans tilstilli tók ég til dæmis þátt í haustskóla stofnunarinnar árið 1975. Sá atburður markaði þáttaskil í mínu lífi, því það var einmitt þá, sem ég ákvað að helga mig stjarneðlisfræði. Á endanum leiddi það til þess, að ég stundaði rannsóknir á nifteindastjörnum hjá Nordita á árunum 1978 til 1981 og lauk jafnframt doktorsprófi frá Kaupmannahafnarháskóla í nóvember 1981. Allan þann tíma naut ég stöðugt hvatningar og velvilja Magnúsar.
Af framansögðu má ráða, að Magnús hafði veruleg áhrif á fyrstu skrefin, sem ég fetaði á frekar óhefðbundnum ferli mínum sem eðlisfræðingur. Eftir að við fórum svo að vinna saman sem eðlisfræðikennarar við Háskóla Íslands, einkenndust öll okkar samskipti ávallt af vinsemd og gagnkvæmri virðingu.
Með heiðursmanninum Magnúsi Magnússyni er enn einn frumherjinn úr hópi íslenskra eðlisvísindamanna horfinn yfir móðuna miklu. Ég kveð hann með söknuði og þakklæti.
*
Eftirfarandi skrár fylgja færslum 4a1 og 4a2 um stjörnufræðinginn Steinþór Sigurðsson.
Steinþór Sigurðsson stjörnufræðingur (1904-1947). Brjóstmynd eftir Einar Jónsson myndhöggvara frá 1948.
* Stjarneðlisfræði og heimsfæði á Íslandi: Efnisyfirlit *
Frumherjinn Trausti Einarsson (1907-1984), er án efa þekktastur fyrir víðfeðmar og merkar jarðeðlisfræðirannsóknir hér á landi í hartnær hálfa öld. Í þessari færslu verður þó ekki rætt um þann mikilvæga þátt í ævistarfi hans. Hér er ætlunin að fjalla fyrst og fremst um Trausta sem stjarneðlisfræðing, en sem kunnugt er var hann doktor í stjörnufræði frá Háskólanum í Göttingen í Þýskalandi. Lesendum, sem vilja fræðast um afrek hans á sviði jarðvísinda, má benda á afmælis- og minningargreinarnar, sem tengt er á hér fyrir neðan. Æviatriði má einnig finna í æviskrám, eins og Verkfræðingatali og Kennaratali. Þá má nálgast frekari upplýsingar um prentuð verk Trausta í meðfylgjandi ritaskrá.
Það skal einnig tekið fram, að ég þekkti Trausta ekki persónulega og vegna eðlis þessa greinaflokks hef ég ekki haft fyrir því að kynna mér bréfaskipti hans við ættingja, vini eða aðra. Þá hef ég ekki heldur átt viðtöl við þá samferðamenn hans, sem enn eru á lífi. Þetta kann að valda því, að í textanum sem hér fer á eftir, kunni að leynast einhverjar staðreyndavillur um ævi hans og feril. Ef lesendur rekast á slíkt, bið ég þá vinsamlegast um að láta mig vita, svo ég geti gert viðeigandi ráðstafanir.
Trausti Einarsson á besta aldri.
Í framantöldum greinum er víða komið inn á viðhorf Trausta til raunvísinda, sem og skoðanir hans á ýmsum mikilvægum atriðum, er tengjast kennslu þeirra og iðkun. Í því sambandi má einnig benda á eftirfarandi ritsmíðar:
Trausti tók gagnfræðapróf utanskóla við Menntaskólann í Reykjavík árið 1924 og settist síðan í skólann, þaðan sem hann lauk stúdentsprófi vorið 1927. Þetta var í rektorstíð Geirs T. Zoëga og Trausti var í sjötta árganginum, sem útskrifaðist úr stærðfræðideild skólans. Aðalkennarar hans voru því frumherjarnir Ólafur Dan Daníelsson stærðfræðingur (1877-1957) og Þorkell Þorkelsson eðlisfræðingur (1876-1961). Stjörnufræðina lærði Trausti hins vegar hjá Jóhannesi Kjartanssyni byggingarverkfræðingi (1900-1928).
Stærðfræðideildarbekkurinn 6C í MR vorið 1927. Aðalkennari bekkjarins, Ólafur Dan Daníelsson, er lengst til hægri. Trausti stendur í sömu röð, þriðji frá vinstri. Á myndinni er einnig Björn Franzson (1906-1974), höfundur bókarinnar Efnisheimurinn, sitjandi lengst til vinstri. Trausti minntist Ólafs og kennslu hans í fallegri minningargrein, árið 1957. Ljósmynd: Jón Kaldal.
Háskólanám í Þýskalandi
Haustið 1927 hélt Trausti til Þýskalands, þar sem hann stefndi á nám í stjörnufræði við Háskólann í Göttingen. Með í för var vinur hans, Leifur Ásgeirsson (1903-1990), sem ætlaði að leggja stund á stærðfræði við sama skóla. Um dvöl þeirra í Göttingen má meðal annars lesa í áðurnefndri minningargrein Ágústs Guðmundssonar um Trausta (bls. 140) og á bls. 45-55 í grein Jóns Ragnars Stefánssonar „Saga stærðfræðings. Af ævi og starfi Leifs Ásgeirssonar“, sem birt var í bókinni Leifur Ásgeirsson – Minningarrit, árið 1998.
Margt er á huldu um það, hverjir kenndu þeim félögum fyrstu árin í Göttingen, en þó er vitað, að þeir tóku sömu grunnnámskeiðin í stærðfræði og raunvísindum. Eftir það hafa námsleiðir væntanlega skilið að mestu. Leifur lauk doktorsprófi í stærðfræði vorið 1933 hjá Richard Courant (1888-1972) með eðlisfræði og eðlisefnafræði sem hliðargreinar. Trausti tafðist hins vegar um eitt ár vegna veikinda og lauk doktorsprófi í stjörnufræði hjá Hans Kienle (1895-1975) vorið 1934, sennilega með eðlisfræði og stærðfræði sem aukagreinar. Í ljósi þess, að rannsóknir Trausta á Íslandi að loknu doktorsprófi snerust nær allar um verkefni í jarðvísindum, er eftirtektarvert, að á námsárunum í Þýskalandi tók hann ekki eitt einasta námskeið á því sviði.
Trausti (til vinstri) og Leifur Ásgeirsson í fríi frá náminu í Göttingen, sennilega árið 1928. Aldarfjórðungi síðar fjallaði Trausti um vin sinn í blaðagrein í tilefni af fimmtugsafmæli Leifs, árið 1953. Myndin er hins vegar úr minningargrein Ágústs Guðmundssonar um Trausta.
Raunvísindi í Göttingen á fyrstu áratugum tuttugustu aldar
Þegar þeir Trausti og Leifur komu til Göttingen haustið 1927, höfðu raunvísindi verið stunduð þar af miklum krafti, allt frá því á seinni hluta átjándu aldar. Felix Klein (1849-1925) og David Hilbert (1862-1943) höfðu gert stærðfræðideildina að stórveldi í heimi strærðfræðinnar á áratugunum í kringum 1900, og um og upp úr 1920 tókst þeim Max Born (1882-1970) og James Franck (1882-1964) að byggja þar upp eina af helstu miðstöðvum hinnar nýju skammtafræði. Efnafræðin lifði einnig góðu lífi í Göttingen og stjörnufræðideild skólans stóðst fyllilega samanburð við flestar aðrar deildir á því sviði í Þýskalandi. Stjörnufræðin átti sér og langa sögu við skólann og þar höfðu menn eins og Tobias Mayer (1723-1762), Carl Friedrich Gauss (1777-1855) og Karl Schwartzschild (1873-1916) gert garðinn frægan.
Stjörnuathugunarstöðin í Göttingen undir lok tuttugustu aldar. Útlit hennar hafði þá lítið breyst frá því á námsárum Trausta. Mynd: Wikipedia.
Hans Kienle, prófessor í stjörnufræði og forstöðumaður stjörnuturnsins. Hann var aðalkennari Trausta og leiðbeinandi hans í doktorsnámi. Mynd: Deutsches Museum, sennilega tekin í kringum 1950.
Fjórir af eðlisfræðikennurunum í Göttingen á námsárum þeirra Trausta og Leifs. Frá vinstri: Max Reich (1874-1941), Max Born, James Franck og Robert Pohl (1984-1976). Hér má lesa stutt minningabrot Borns um árin 1921 til 1933 í Göttingen. Myndin er tekin 1923.
Við valdatöku nazista, árið 1933, lauk blómatíma raunvísinda í Göttingen all hastarlega, og hið sama á reyndar við um vísindi í Þýskalandi öllu. Bæði Trausti og Leifur sluppu þó þaðan fullmenntaðir, áður en ógnarstjórn nazista hófst fyrir alvöru.
Bókabrenna nazista við Háskólann Göttingen, 10. maí 1933. Leifur Ásgeirsson var þá nýkominn heim, alfarinn frá Þýskalandi. Trausti var í veikindaleyfi á Íslandi, en þurfti að fara aftur út, haustið 1933, til að skrifa doktorsritgerðina, sem hann lauk svo við vorið 1934. Sjá viðtalið Nýr íslenzkur doktor í Alþýðublaðinu, 25. apríl 1934. Mynd: Cantor’s Paradise.
En snúum okkur nánar að Trausta Einarssyni og stjarnvísindanámi hans. Í því, sem á eftir fer, er ein helsta heimild mín um tíðarandann í Göttingen á þessum árum (einkum fyrir 1933) viðtal við hinn merka stjarneðlisfræðing Martin Schwarzscild (1912-1997), son Karls Schwarzschild, þess sem hin fræga lausn á sviðsjöfnum Einsteins er kennd við. Martin var við nám í Göttingen á svipuðum tíma og Trausti og hafði sama leiðbeinanda, Hans Kienle. Hann lauk doktorsprófi árið 1935, ári á eftir Trausta. Ætla má, að leiðir þeirra hafi legið saman í stjörnuathugunarstöðinni á lokaári Trausta í Göttingen, 1933-1934.
Martin Schwarzschild á dögum seinni heims-styrjaldarinnar. Hann varð bandarískur ríkisborgari árið 1942 og tók virkan þátt í baráttunni við nazista. Fjöldi stjarnvísindamanna, þar á meðal færslu-höfundur, lærðu aðferðirnar við útreiknga á stjörnulíkönum af bók hans, Structure and Evolution of the Stars, frá 1958.
Meðal þess, sem Schwarzschild nefnir í viðtalinu, er að „the Göttingen Observatory at that time was a very happy place.“
Um Kienle segir hann meðal annars:
He definitely looked at astronomy in the modern sense of astrophysics even though obviously he himself, to some level, trained us in classical astronomy. You still had to compute a comet orbit and learn the truly classical sort of astronomy. ... Actually Kienle closed all the meridian circles and went entirely into photometry. There was [only] one astrometric instrument preserved to train us students. ... Kienle was entirely a modern astronomer, but he was not a theoretician. ... It was an extremely lively place, and Kienle was extremely good – even though he himself maybe was not an outstanding astronomer – to recognize good young people. ... [The technician, Friedrichs] was essentially the head of a small shop and played a very important role in the laboratory for absolute photometry which Professor Kienle made the main program of the observatory.
Þótt Kienle væri aðal stjarnvísindakennarinn, komu ýmsir aðrir einnig að kennslunni. Þar á meðal var næstráðandi Kienles í stjörnuturninum, „hinn aldraði“ Bruno Meyermann (1876-1963), sem kenndi svo til alla sígilda stjörnufræði, þar á meðal himinkúlufræði, tímareikning og útreikninga á brautum himintungla. Um hann segir Schwarzschild í viðtalinu: „Observator, Meyermann - Geheimrat Meyermann was quite an old man - He was an extremely kind and friendly man and did all the classical teaching.“
Á Göttingenárum Trausta voru þar einnig Otto Heckmann (1901-1983), helsti aðstoðarmaður Kienle frá árinu 1927 og síðan prófessor frá 1935. Hann varð síðar þekktur heimsfræðingur og fyrsti forstöðumaður Stjörnuathugunarstöðvar Evrópu á suðurhveli (ESO). Þá kom Rupert Wildt (1905-1976), aðstoðarmaður Kienle á árunum 1930-33, jafnframt að kennslunni.
Meðal þeirra, sem kenndu stjarnvísindi með Kienle á dögum Trausta í Göttingen, voru (frá vinstri): Bruno Meyermann, Otto Heckmann og Rupert Wildt.
Eitt af því, sem kemur fram í viðtalinu, er að Kienle ráðlagði nemendum sínum eindregið að taka hið þekkta ljósfræðinámskeið, sem Max Born kenndi, allt þar til hann þurfti að yfirgefa Þýskaland vegna ofsókna nazista árið 1933. Sá sem tók við kennslunni af Born var Otto Heckmann. Hvort Trausti sat þetta námskeið hjá Born, veit ég ekki. Hins vegar hlýtur hann að hafa fengið einhverja tilsögn í skammtafræði í námskeiðum hjá honum eða samstarfsmönnum hans.
Akademískt ár í München, 1930-1931
Að loknu þriggja ára grunnnámi í stjörnufræði í Göttingen, árið 1930, var Trausti í eitt ár við Háskólann í München. Það var í samræmi við hefð, sem lengi hafði tíðskast meðal þýskra háskólastúdenta, og gerir kannski enn. Stjörnuathugunarstöðin í München var reyndar í niðurníðslu á þessum tíma, svo leiða má getum að því, að Trausti hafi fyrst og fremst farið til borgarinnar til að sækja tíma hjá sjálfum „skammta-páfanum“, Arnold Sommerfeld (1868-1951) eða samstarfsmönnum hans. Meðal stjarneðlisfræðinga var Sommerfeld einkum þekkur fyrir bók sína, Atombau und Spektrallinien, „biblíu“ þeirra, sem unnu við litrófsgreiningu, hvort heldur var í stjarneðlisfræði, eða á öðrum sviðum.
Sommerfeld heldur fyrirlestur um litróf, árið 1937.
Um áhrif litrófsmælinga á þróun stjarnvísinda má meðal annars lesa í eftirtöldum greinum:
Fyrsta prentaða ritsmíð Trausta, Um byggingu stjarnanna, var sennilega skrifuð sumarið eða haustið 1931, annað hvort í München, eða eftir að hann var aftur kominn til Göttingen til að hefja vinnu við doktorsverkefnið. Greinin birtist í Eimreiðinni í vetrarbyrjun 1931 og er fyrsta umfjöllunin um þetta mikilvæga efni eftir Íslending með sérmenntun í stjarnvísindum. Hún gefur fjörlegt og gott yfirlit yfir ríkjandi hugmyndir vísindamanna um sólina og aðrar sólstjörnur á árunum í kringum 1930.
Bakgrunnur
Á millistríðsárunum voru rannsóknir á yfirborðseiginleikum sólstjarna, innri gerð þeirra og þróun, eitt heitasta viðfangsefni stjarneðlisfræðinnar. Margir mætir vísindamenn komu þar við sögu, en þeirra áhrifamestir voru lengi vel Bandaríkjamaðurinn Henry N. Russell (1877-1957) og Englendingurinn Arthur S. Eddington (1882-1944). Meginástæðan fyrir hinni öru þróun voru framfarir í eðlisfræði á árunum 1860 til 1930, meðal annars í ljós- og litrófsmælingum, hreyfifræði gasa og hinni nýju atómeðlisfræði, sem grundvölluð var á skammtafræði.
Í dag er Russell einna þekktastur fyrir hið svokallaða Hertzsprung-Russell (HR) línurit, sem gegnir afar mikilvægu hlutverki í fræðunum um eiginleika og þróun sólstjarna. Auk Russells er línuritið kennt við danska efnaverkfræðinginn Ejnar Hertzsprung (1873-1967).
Stjarnvísindamennirnir Ejnar Hertzsprung til vinstri og Henry N. Russell.
Hér fyrir neðan má sjá hina frægu „frumútgáfu“ HR-línuritsins, sem Russell birti árið 1914 (sjá t.d. greinina Relations Between the Spectra and Other Characteristics of the Stars, bls. 285 og 288). Skömmu áður, árið 1911, hafði Hertzsprung birt svipaðar niðurstöður í grein sinni „Über die Verwendung Photographischer Effektiver Wellenlaengen zur Bestimmung von Farbenaequivalenten“ í ritinu Publikationen des Astrophysikalischen Observatoriums zu Potsdam (22. Bd., 1. Stuck = Nr.63). Vegna þess, hversu fáir lásu Potsdam-frréttabréfið að staðaldri, voru línurit af þessu tagi eingöngu kennd við Russell allt fram til 1933. Það var hinn þekkti danski stjarneðlisfræðingur, Bengt Srömgren (1908-1987), sem fyrstur innleiddi nafnið sem við notum í dag, þ.e. Hertzsprung-Russell línuritið (sjá Strömgren, B., 1933: On the Interpretation of the Hertzsprung-Russell-Diagram.)
Línurit Russels frá 1914: Lárétti ásinn sýnir litrófsflokka nálægra sólstjarna og sá lóðrétti reyndarbirtustig þeirra. Punktar og hringir á myndinni tákna mismunandi stjörnur. Efst eru bjartar risastjörnur, en neðst daufar dvergstjörnur. Heildarútgeislun stjarnanna fer því vaxandi eftir því sem ofar dregur og yfirborðshiti þeirra fer hækkandi frá hægri til vinstri. Hallandi línurnar tvær afmarka svokallaða meginröð. Ofarlega, lengst til hægri, fyrir ofan meginröðina, eru rauðir risar, en lengst til vinstri, langt fyrir neðan hana, má sjá tvo hvíta dverga. Mikilvægt er að hafa í huga, að reyndarbirtustigið gefur ljósafl stjörnunnar, L, og litrófsflokkurinn tengist yfirborðshitastigi hennar, T.
Árið 1926 gaf Eddington út vandað yfirlitsrit um kennilegar rannsóknir sínar á eiginleikum og þróun sólstjarna. Bókin varð fljótlega ómissandi grundvallarrit á fræðasviðinu. Sem dæmi má nefna, að Kienle, leiðbeinandi Trausta, hvatti alla nemendur sína í Göttingen til að lesa bók Eddingtons vandlega. Ekki er að efa, að Trausti var í hópi þeirra fjölmörgu, sem urðu fyrir miklum áhrifum frá þessu tímamótaverki Eddingtons.
Hér heima höfðu alþýðufræðarar haft fréttir af því, að eitthvað áhugavert væri að gerast á þessu sviði erlendis. Það varð meðal annars til þess, að fjallað var um efnið í eftirfarandi ritsmíðum:
Framangreind verk eru misjafnlega góðar þýðingar og/eða endursagnir á misgömlum erlendum alþýðuritum, enda hafði enginn af íslensku höfundunum fengið nægjanlega tilsögn í stjarneðlisfræði til að skila verki sínu hnökralaust. Því kann vel að vera, að Trausta hafi þótt mikilvægt að láta í sér heyra hér heima, einkum til að upplýsa íslenskan almenning um raunverulega stöðu þessara fræða erlendis. Um þetta er að sjálfsögðu ekkert hægt að fullyrða, en ljóst er að Trausti hafði fylgst vel með umræðum um þetta efni í fyrirlestrum, bókum og tímaritum, bæði í Göttingen og München.
Grein Trausta
Í upphafi Eimreiðar-greinarinnar frá 1931 minnir Trausti lesendur á fullyrðingar hins merka franska heimspekings Augustes Compte árið 1835 (reyndar án þess að nefna hann), þess efnis að „menn myndu aldrei geta fengið neina vitneskju um efnin og ástand þeirra á stjörnunum“. Í framhaldinu fjallar hann svo um það, hvernig litrófsfræðin færði mönnum lykilinn að núverandi þekkingu á fastastjörnunum, gerð þeirra og þróun (bls. 343):
Við notkun litrófsins í þágu stjörnufræðinnar er stigið eins stórt spor í þekkingaráttina og þá er Newton notaði fyrst aflfræðina til þess að skýra hreyfingar reikistjarna og tungla.
Fjórum árum síðar, 1935, skrifaði Trausti ítarlega grein í Tímarit Verkfræðingafélags Íslands um notkun litrófsmælinga í stjarneðlisfræði. Sú grein verður tekin til umfjöllunar í lok þessarar færslu.
Spurningarnar, sem Trausti leitast einkum við að svara í grein sinni, eru tvær: Hvað eru stjörnurnar? og Hvernig er ástandið á þeim?
Þegar grein Trausta er lesin, ber að hafa í huga, að hún er meira en 90 ára gömul og gífurlegar framfarir hafa orðið í stjarnvísindum á þeim tíma, sem síðan er liðinn. Þeim sem vilja kynna sér hugmyndir fræðimanna um stjörnurnar fyrir daga Trausta, er bent á fyrri færslur í þessum greinaflokki. Um núverandi þekkingu á gerð og þróun sólstjarna má hins vegar lesa í nýlegum kennslubókum í stjörnufræði og stjarneðlisfræði. Sjá einnig eftirfarandi heimildir:
Trausti notar talsvert rúm til að fjalla um það, hvernig nota má litróf til að ákvarða efnasamsetningu, hitastig, þéttni og þrýsting á yfirborði stjarnanna og skipa þeim í litrófsflokka. Jafnframt leggur hann áherslu á það, að litrófsmælingar hafi sýnt að sömu frumefni sé alls staðar að finna í sýnilegum alheimi og að þar gildi einnig sömu náttúrulögmál og í sólkerfinu.
Þá gefur Trausti dágóða lýsingu á sólinni, yfirborði hennar og virkni. Einnig fjallar hann um áhrif sólar á heimkynni okkar jarðarbúa og nefnir sem dæmi þyngdarkraftinn, sem heldur jörðinni á braut sinni, rafsegulgeislunina sem gerir hana lífvænlega og agnastreymið, sem meðal annars veldur norðurljósum.
Í lýsingu sinni á innri gerð sólstjarna fylgir Trausti í meginatriðum viðteknum hugmyndum samtímans, ekki síst eins og þær eru fram settar í bók Eddingtons, þar sem gert er ráð fyrir að „stjörnurnar [séu] hnattlöguð samsöfn af glóandi lofttegundum“. Þó fjallar hann með velþóknum um harða gagnrýni Edwards A. Milnes (1896-1950) á verk Eddingtons, og þótt hann nefni það ekki greininni, hefur hann án efa vitað af tengdri gagnrýni James Jeans (1877-1946) á Eddington og aðferðafræði hans. Rétt er að geta þess hér, að bæði Milne og Jeans höfðu margt til síns máls, einkum þó hinn síðarnefndi. En á endanum var það samt Eddington, sem í öllum meginatriðum stóð uppi sem sigurvegari í þessum deilum fræðimannanna.
Eddington brást hins vegar illilega bogalistin skömmu síðar (1935), þegar hann dæmdi kennilegar niðurstöður hins snjalla Indverja, Subrahmanyan Chandrasekhar (1910-1995), um hámarksmassa hvítra dverga opinberlega dauðar og ómerkar.
Þrír úr hópi þekktustu stjarnvísindamanna Breta á fyrri hluta tuttugustu aldar. Frá vinstri: Arthur S. Eddington, James Jeans og Edward A. Milne. Bæði Jeans og Eddington gáfu einnig út bækur og greinar um raunvísindi fyrir almenning, sem þýddar voru á mörg tungumál. Meðal annars birtust nokkrar ritsmíðar þeirra í íslenskum blöðum og tímaritum.
Þegar Trausti fjallar í grein sinni um eiginleika sólstjarna almennt, raðar hann þeim eftir stærð (þ.e. radíus, R) og lýsir því, hverrnig þyngdin (þ.e massinn, M) og ljósmagnið (þ.e. ljósaflið, L) breytist með R. Það er ljóst, að í þeirri umfjöllun styðst hann annars vegar við áðurnefnt HR-línurit og hins vegar við hið þekkta ML-línurit, sem sýnt er hér fyrir neðan. Ef bæði L og T eru þekktar stærðir, má fá gildið á R út frá formúlunni L = 4πR2σT4, þar sem σ er svokallaður Stefan-Boltzmann fasti.
Hið fræga massa-ljósafls (ML-) línurit Eddingtons um samband reyndarbirtustigs sólstjarna (á lóðrétta ásnum) og massa þeirra (á lárétta ásnum). Sjá grein hans frá 1924: On the relation between the masses and luminosities of the stars. Um sögu rannsókna á þessum venslum má t.d. lesa hjá Kuiper, G.P., 1938: The Empirical Mass-Luminosity Relation (sjá einkum bls. 473-74). Á þessum tíma var eingöngu hægt að fá mælikvarða á massa fjarlægra sólstjarna, ef þær voru í tvístirnum.
Í greininni fylgir Trausti í öllum meginatriðum kenningu Russels frá 1925 um þróun stjarna. Henni er lýst í skýringartextanum við næstu mynd. Kenningin byggir að hluta á eldri hugmyndum Englendingsins J. Normans Lockyer (1836-1920), en varð fljótlega úrelt.
Myndin lýsir kenningu Russells um þróun sólstjarna, eins og hún birtist í greinunum The Problem of Stellar Evolution og The Theory of Stellar Evolution Modified frá 1925. Skv. henni hefja stjörnurnar ævi sína sem rauðir risar við lágt hitastig (efst til hægri) og dragast síðan saman og hitna þar til þær ná stöðugu jafnvægi á meginröðinni. Við það breytist hluti af massa þeirra í orku (sbr. E = mc2) og geislar í burtu. Stjörnurnar kólna því hægt og rólega og ferðast við það niður eftir meginröðinni. Þegar þær eru orðnar nægjanlega kaldar og rauðar falla þær saman, losa sig við yfirborðslögin í sprengingum (nýstirni?), hitna við það á nýjan leik og verða loks að heitum hvítum dvergum (neðst til vinstri) og síðan svörtum. Þessari þróun er lýst í HR-línuritinu með ferlinum, sem er eins og S í laginu. Vart þarf að taka fram, að þessi þróunarkenning er röng.
Trausti vissi ekki, frekar en aðrir stjarnvísindamenn á þessum tíma, hvaðan sólstjörnurnar fengu hina gífurlegu orku, sem frá þeim geislaði. Hann bendir þó á, að sennilega sé uppsprettuna að finna í þeirri uppgötvun Einsteins, „að efni og orka séu tvær myndir sama hlutar“ (þ.e. E = mc2) og „að hugsanlegt [sé] að breyta megi einu í annað.“ Ýmsar hugmyndir höfðu þá þegar komið fram um það, hvernig slíkt gæti gerst í stjörnunum, en það var ekki fyrr en átta árum eftir að grein Trausta kom út, sem endanleg lausn fannst á vandamálinu. Þar var að verki hin þekkti þýsk-bandaríski eðlisfræðingur Hans Bethe (1906-2005). Um sögu þessa flókna viðfangsefnis og lausn þess má lesa í eftirfarandi heimildum:
Alveg í lok greinarinnar kemur Trausti svo inn á vandamál, sem átti eftir að vera honum hugleikið það sem eftir var ævinnar, nefnlega spurninguna um uppruna sólkerfisins. Þar segir:
Stjörnurnar eru dreifðar víðsvegar um geiminn, að meðaltali í órafjarlægð hver frá annari. En komi það fyrir, að saman beri fundum tveggja þeirra, sem sjaldgæft mun vera, getur það orðið uppruni nýrra stjarna og nýs lífs. Því ef stjörnurnar þjóta hvor fram hjá annari, myndast á þeim flóðbylgjur, eins og á jörðu af völdum tunglsins. Þessar flóðbylgjur geta risið svo hátt, að þæri losni við stjörnuna og fari að hringsóla í kringum hana. Á þann hátt verða til reikistjörnur. Þær kólna fyrr en sólin sjálf, og skapast þannig skilyrði fyrir líf, slíkt sem við mennirnir njótum góðs af.
Þetta er greinilega lýsing á hinni svokölluðu flóðbylgjukenninu James Jeans frá 1919. Hún var mjög vinsæl um tíma, en stóðst ekki tímans tönn. Henni er ágætlega lýst í bók hans, The Universe Around Us frá 1929 (bls. 232-236). Til gamans má geta þess, að í bókinni Heimsmynd vísindanna (1931) notar Ágúst H. Bjarnason þetta verk Jeans sem heimild um myndun sólkerfisins (bls. 97-99) og fleiri vísindaleg viðfangsefni.
Eins og áður er getið, kom Trausti nokkrum sinnum aftur að vandmálinu um uppruna stjarnfræðilegra fyrirbæra. Fyrst 1937 í lok greinarinnar Tunglið. Síðan 1944 í Ágripi af stjörnufræði handa menntaskólum, þar sem hann setur fram sömu kenningarnar og 1931 um myndun reikistjarna (bls. 33) og þróun sólstjarna (bls. 45). Síðar á ferlinum fjallaði hann þó um nýrri hugmyndir, meðal annars í greininni Uppruni tungls og innri gerð frá 1969, í öðrum kafla inngangs að bókinni Eðlisþættir jarðarinnar og jarðsaga Íslands frá 1972 (bls. 13-20) og loks í greininni Stjörnuhröp og vígahnettir frá 1976.
Í fyrstu neðanmálsgreininni í doktorsritgerð Trausta, Über die Möglichkeit fortlaufender Koronabeobachtungen (Um möguleikann á samfelldum athugunum á kórónu sólar), sem hann varði í Göttingen í apríl 1934, segir hann, að rannsóknarvinnan hafi að mestu farið fram árið 1932, en vegna veikinda hafi hann ekki lokið endanlegri úrvinnslu fyrr en í árslok 1933.
Efni ritgerðarinnar mætti flokka sem tilraunaljósfræði í tengslum við athuganir á nánasta umhverfi hinnar gífurlega björtu sólkringlu. Þar er hin fallega kóróna, sem er um það bil milljón sinnum daufari en sjálf kringlan. Trausti hefur sennilega byrjað að undirbúa mælingarnar fljótlega eftir komuna frá München 1931 og framkvæmt þær veturinn 1931-32. Hann var óvinnufær vegna veikinda veturinn 1932-33 og dvaldist þá heima á Íslandi. Haustið 1933 fór hann svo aftur til Göttingen til að ljúka verkinu.
Í framhjáhlaupi má nefna það hér, að á aðfangadag 1933 birti Trausti athyglisverða alþýðlega grein í Alþýðublaðinu undir fyrirsögninni Stjörnukíkir fyrir almenning. Ekki er ósennilegt, að hvatinn að henni hafi verið heimsókn í hið fræga stjörnuver í München á sínum tíma. Í greininni færir hann sterk menningarleg rök fyrir því, að æskilegt væri að efna til samskota til kaupa á „20-25 cm víðum spegilkíki ... með nauðsynlegum aukahlutum“ og koma honum fyrir í „all rambyggilegu skýli ... á heppilegu húsþaki“ í höfuðborginni. Bendir hann á „þak Austurbæjarskólans, sem hentugt kíkissvæði“. Þessi tillaga Trausta virðist ekki hafa hlotið miklar undirtektir meðal borgarbúa og það var ekki fyrr en rúmum fjörutíu árum síðar, sem draumur hans varð að veruleika. Þá kom Stjörnuskoðunarfélag Seltjarnarness fyrir 35 cm víðum spegilsjónauka á þaki Valhúsaskóla. – En snúum okkur nú aftur að doktorsverkefninu.
Bakgrunnur
Kóróna sólar hefur eflaust verið þekkt frá ómunatíð, þótt hún hafi, allt fram til 1930, eingöngu birst þeim, sem horfðu á almyrkva á sólu, og þá aðeins stuttan tíma í einu. Elstu rituðu heimildina um kórónuna er að finna í verki gríska heimspekingsins og sagnaritarans Plútarks (45-120), De Facie in Orbe Lunae (Um andlitið á tunglinu) og talið er, að það hafi ekki verið fyrr en í lok fyrsta fjórðungs átjándu aldar, sem menn áttuðu sig á því, að kórónan tilheyrði sólinni en ekki tunglinu.
Sjá einnig:
Áhugi vísindamanna á eðliseiginleikum sólarinnar fór ekki að vaxa fyrir alvöru fyrr en um og upp úr miðri nítjándu öld og þá einkum vegna tilkomu fræðigreina eins og varmafræði og rafsegulfræði og tækjabúnaðar til ljósmyndatöku, ljósmælinga, litrófsmælinga og skautunarmælinga (sjá í þessu sambandi færslu 3a eftir Einar H. Guðmundsson frá 2021: Nýja stjörnufræðin berst til landsins og heimildir, sem þar er bent á). Mikilvægi almyrkva við notkun slíks búnaðar við rannsóknir á kórónunni og næsta nágrenni sólar varð og til þess, að stjarnvísindamenn lögðu á sig ómælda vinnu og erfiðleika til að elta myrkvana uppi. Um nokkra slíka vísindaleiðangra má lesa hér:
Sólvísindamenn dreymdi að sjálfsögðu um, að losna við hina umfangsmiklu og kostnaðarsömu myrkvaleiðangra til afskekktra staða og geta í staðinn gert nauðsynlegar mælingar á kórónunni og umhverfi hennar í hefðbundnum stjörnuathugunarstöðvum, helst á heimaslóðum. Langflestir voru þess þó fullvissir, að slíkt væri ekki mögulegt af ýmsum tæknilegum ástæðum.
Sem dæmi má nefna að Kienle, aðalkennari Trausta Einarssonar, taldi að þetta væri algjörlega óframkvæmanlegt vegna birtu himinsins í nágrenni sólarinnar og byggði þar á eigin athugunum í Göttingen og víðar í lok þriðja áratugsins:
Hér fyrir neðan er nýlegt línurit, sem sýnir hvað Kienle átti við með fullyrðingu sinni.
Hlutfallsleg birta (B/B☉) mismunandi þátta sólkórónunnar (K, F, E) sem fall af fjarlægðinni frá sólarmiðju (r/R☉). Hér er B☉ birta sólkringlunnar og R☉ er radíus hennar (athugið að ☉ er hefðbundið tákn fyrir sólina). Eins og sjá má, er birta himinsins í kringum 10-6 B☉ við bestu hugsanlegu skilyrði að degi til. Kórónan er almennt mun daufari. Um miðjan almyrkva er birta heiðskírs himins hins vegar nær þremur tugaþrepum minni og kórónan kemur greinilega í ljós. Línuritið er fengið að láni úr grein A. Liberatore og félaga frá 2022: Sky Brightness Evaluation at Concordia Station, Dome C, Antarctica, for Ground-Based Observations of the Solar Corona.
Einn vísindamaður vildi þó ekki játa sig sigraðan. Það var franski stjörnufræðingurinn Bernard Lyot (1897-1952), sem var viss um, að mögulegt væri að ljósmynda kórónuna í dagsbirtu með því að skyggja á sólina með þar til gerðum búnaði inni í sjónaukanum.
Sumarið 1930 tókst honum, með eigin frumgerð svokallaðrar kórónusjár, að sjá sólstróka með berum augum að degi til og bæði skautun og sterkustu ljómlínur kórónunnar með eigin mælitækjum. Athuganirnar gerði hann í 2,9 km hæð við stjörnuathugunarstöðina á Pic du Midi, sem er eitt af Pýreneafjöllunum.
Lyot tókst einnig að ljósmynda ljómlínurnar og sólstrókana, og sjálfur sá hann votta fyrir kórónunni á ljósmyndaplötunum. Hún var þó svo dauf, að það tók hann nokkurn tíma að sannfæra aðra um tilvist hennar á myndunum (sjá m.a. greinar hans L'Étude de la couronne solaire en dehors des eclipses (1931) og Étude de la couronne solaire en dehors des éclipses (1932)).
Til dæmis var Kienle ekki sannfærðari en svo, að sumarið 1931 gerði hann sér sérstaka ferð upp á fjallið Groẞglockner í Austurríki með sjónauka, ljósmyndabúnað, litrófsmæli og skautunarmæli í farteskinu, til að sannreyna mælingar Lyots. Hinn merki þýski litrófsfræðingur, Walter Grotrian (1890-1954), kom í heimsókn til Kienle á fjallinu til að fylgjast með mælingum hans, sem staðfestu niðurstöður Frakkans, Þjóðverjunum til mikillar undrunar (um þennan rannsóknarleiðangur má lesa í grein Kienles frá 1932: Astronomische Beobachtungen auf der Adlersruhe am Großglockner).
Lyot og fleiri áttu síðan eftir að endurbæta kórónusjána verulega, og í dag er hún talin í flokki mikilvægustu tækja til sólarrannsókna.
Bernard Lyot (til vinstri) ásamt mynd af kórónu sólar og sólstrókum, sem tekin var með kórónusjá hans, sumarið 1936.
Verk Lyots, ásamt mælingum Kienle og annarra Göttingen-manna, þar á meðal Trausta Einarssonar, urðu til þess vorið 1932, að Kienle neyddist til að endurskoða fyrri fullyrðingar sínar um að birta himinsins kæmi í veg fyrir myndatökur af kórónunni að degi til. Hann viðurkenndi jafnframt, að fyrri mælingar hans hefðu mistekist vegna of mikillar ljósdreifingar inni í sjónaukunum, sem notaðir voru.
Doktorsritgerðin
Það virðist nokkuð ljóst, að niðurstöður Lyots og áhugi Kienle á kórónurannsóknum réði mestu um það, hvaða viðfangsefni Trausti fékk úthlutað sem doktorsverkefni. Í því sambandi má benda á, að einu heimildirnar, sem Trausti vísar í um fyrri kórónurannsóknir, eru áðurnefndar greinar Kienles (frá 1928) og Lyots (frá 1932). Að auki vitnar hann í þrjá aðra höfunda og þá um rannsóknir á ljósbeygju. Þeir eru: L.G. Gouy, E. Maey og H. Nagaoka. Einnig má nefna, að í greininni þakkar Trausti aðeins tveimur mönnum fyrir aðstoð: Leiðbeinanda sínum, Kienle, fyrir að hafa stungið upp á verkefninu og samstúdent sínum, Johann Wempe, fyrir tæknilega aðstoð.
Verkefni Trausta snerist um það að kanna og bera saman dreifingu sólarljóss, annars vegar í lofthjúpi jarðar að degi til og hins vegar inni í sjónpípu kórónusjár. Eins og þegar hefur verið nefnt, gerði Trausti flestar tilraunirnar árið 1932, en missti síðan úr heilt ár vegna veikinda, þannig að ritgerðin var ekki skrifuð fyrr en haustið 1933 og loks varin í vetrarlok 1934. Hún birtist í tímaritinu Zeitschrift für Astrophysik undir heitinu Über die Möglichkeit fortlaufender Koronabeobachtungen. Verkið er all tæknilegt og því verður ekki farið í smáatriði hér, en áhugasömum er vísað á greinina sjálfa.
Til að mæla ljósdreifinguna inni í sjónpípunni notaði Trausti ljósið frá gervisól (500 W ljósgjafa) og margskonar linsur og ljóssíur til að fá sem bestar niðurstöður (sjá myndina Abb. 2. hér fyrir neðan). Tilraunirnar voru allar gerðar á rannsóknarstofu stjörnufræðideildarinnar.
Þessi mynd úr doktorsritgerð Trausta (Abb. 2., bls. 211) sýnir optíkina, sem hann notaði við mælingar á dreifingu ljóss frá gervisólinni inni í sjónaukanum. – L er ljósgjafinn (gervisólin) lengst til hægri og PL er ljósmyndaplatan lengst til vinstri. Bl3 er ógagnsæ látúnsskífa. Nánari skýringar er að finna í grein Trausta.
Mælingar á ljósdreifingu í lofthjúpnum í nágrenni sólkringlunnar á hvelfingunni framkvæmdi Trausti á þaki Hainberg-stjörnuathugunarstöðvar Göttingenháskóla, sem þá var tiltölulega ný.
Hainberg athugunarstöðin var reist á vegum Göttingenháskóla árið 1929. Á dögum Trausta var þar einn 14 þumlunga kíkir, sem eingöngu var notaður til ljósmyndatöku, ásamt 10 þumlunga Fraunhofer linsusjónauka til að beina honum í rétta átt. Síðar bættust við fleiri mælitæki og annar stjörnuturn var reistur í næsta nágrenni. Athugunarstöðin er nú í umsjá Stjörnuskoðunarfélagsins í Göttingen.
Myndin (Abb. 7., bls. 217 hjá Trausta) sýnir optíkina í mælingum á dreifingu ljóssins í lofthjúpi jarðar frá nánasta umhverfi sólkringlunnar. Ljósið kemur inn í sjónpípuna frá hægri, en lengst til vinstri er ljósmyndaplatan, P. Nánari skýringar eru í grein Trausta.
Eftir því, sem ég best fæ séð, voru helstu niðurstöðurnar úr þessum ljósdreifingarannsóknum Trausta þær, að inni í sjónpípu einfaldrar kórónusjár er dreifingin almennt mun meiri en flestir, þar á meðal Kienle, höfðu áður gert ráð fyrir. Jafnframt að hún yfirgnæfi ljósdreifinguna í lofthjúpnum, nema gerðar séu sérstakar ráðstafinir til að draga verulega úr henni, líkar þeim sem Lyot hafði þegar gert. Athuganir á kórónunni með vel hannaðri kórónusjá á háu fjalli séu því mögulegar, ef sólin er nógu hátt á lofti og himinninn heiðskír.
Segja má, að með þessum vönduðu mælingum hafi Trausti lítið annað gert en sannreynt hugmyndir Lyots og jafnframt sannfært Kienle og aðra þýska efasemdarmenn um mikilvægi rannsókna hins franska stjarnvísindamanns. Það er sennilega ástæða þess, að lítið virðist hafa verið vitnað í grein Trausta á sínum tíma. Þó getur hinn þekkti þýski stjarneðlisfræðingur, Albrecht Unsöld, um hana í hinu mikla yfirlitsriti sínu, Physik der Sternatmosphären: Mit besonderer Berücksichtigung der Sonne, frá 1938.
Að lokinni doktorsvörninni kvaddi Trausti Göttingen og hélt til Íslands. Fljótlega eftir heimkomuna með Goðafossi tók Alþýðublaðið við hann viðtal og birti, 25. apríl 1934, undir heitinu Nýr íslenzkur doktor. Nokkrum dögum síðar kom svo um hann stutt grein, Nýr doktor, í Vestmannaeyjablaðinu Víði.
Þessi mynd af Trausta birtist með viðtali Alþýðublaðsins við hann í apríl 1934.
Í minningargrein Ágústs Guðmundssonar frá árinu 1985 segir frá því á síðu 140, að vegna mikillar óvissu um starf á Íslandi, hafi Trausti, skömmu fyrir brottförina frá Göttingen, gengið á fund svissnesk-norska jarðefnafræðingsins Victors Goldschmidt (1888-1947), prófessors í steindafræði, og spurt hann ráða. Goldschmidt mun hafa svarað, „að ef hann fengi ekki rannsóknarstöðu í stjörnufræði á Íslandi, þá gæti hann margt verra gert en að sinna jarðfræðirannsóknum, og lagði áherslu á þá miklu möguleika sem Ísland hefði upp á að bjóða á því sviði“. Þess skal geta, að fram að þessum tíma hafði Trausti ekki sýnt jarðvísindum hinn minnsta áhuga.
Victor Goldschmidt er stundum kallaður „faðir jarðefnafræðinnar“. Myndin, sem fengin er að láni hjá Wikipediu, er tekin 1935, um það leyti sem hann yfirgaf Göttingen vegna yfirgangs nazista og flutti til Noregs.
Störf Trausta á Íslandi á sviði stjarnvísinda verða rædd nánar í annarri færslu (4b2), en hér er ætlunin að ljúka þessum hluta með umfjöllun um grein, sem hann birti í Tímariti Verkfræðingafélags Íslands haustið 1935 og er byggð á efni, sem hann hefur greinilega kynnt sér vel á námsárunum í Þýskalandi.
Grein Trausta ber heitið Litrofin og þýðing þeirra fyrir rannsóknir á sólinni. Hún er að öllum líkindum að mestu byggð á erlendum heimildum, og þá helst þýskum, þótt þeirra sé ekki getið í greininni. Um er að ræða metnaðarfulla og tæknilega grein, sem greinilega er ekki ætluð sem alþýðufræðsla, enda birt í tímariti verkfræðinga. Strax í upphafi vekur það sérstaka athygli, að Trausti notar orðið litrof í staðinn fyrir litróf, sem flestir nútímalesendur kannast betur við. Það sem kannski skiptir þó meira máli er að þetta er fyrsta „alvöru“ greinin, sem rituð er á íslensku um vísindalegar rannsóknir á sólinni.
Þeim, sem hafa áhuga á að kynna sér sögu sólarrannsókna ítarlegar en hér er gert, má benda á eftirfarandi yfirlitsgreinar í bókinni History of Astronomy: An Encyclopedia (ritstj. J. Lankford) frá 1997:
Grein Trausta er skipt í þrjá kafla með fyrirsögnunum Litrofin, Atommyndin og Sólin og er sá síðasti þeirra lengstur.
Litróf og atóm
Í byrjun er almenn umfjöllun um litróf, með áherslu á svokölluð línuróf. Rætt er um rafeindir í atómum, líkindasvæði (svigrúm) þeirra og Rydberg-formúluna frægu fyrir litrófsraðir þeirra Lymans, Balmers, Paschens og Bracketts. Jafnframt er því lýst, hvernig hægt er að útvíkka hana fyrir alkalímálmana, en ekki jarðalkalímálma. Síðar fjallar Trausti einnig um muninn á bogalitrófum og neistalitrófum. og minnist á hina svokölluðu Sommerfeld-Kossel reglu. Öll er umræðan tengd lotukerfi frumefnanna.
Mynd ásamt tilheyrandi texta úr grein Trausta Einarssonar frá 1935. Þarna má sjá bæði gleypilínur (í litrófi sólarinnar) og ljómlínur frumefnanna vetnis, helíns og neons.
Trausti fjallar einnig um mögulegar færslur rafeinda milli orkustiga inni í atómum og birtir svokölluð Grotrian línurit fyrir bæði natrín og kalsín. Hann notar og erlenda orðið „term“ fyrir það sem við nú köllum venjulega fjölstig á íslensku.
Til hægri er þýski litrófsfræðingurinn Walter Grotrian (1890-1954), sá sem fyrstur setti fram orkulínurit af því taginu, sem notuð eru í atómeðlisfræði dag. Til vinstri er Grotrian línurit (orkulínurit) af fjölstigum ójónaðs natríns (úr grein Trausta, bls. 32). Það er greinilega fengið að láni úr bók Grotrians frá 1928: Graphische Darstellung der Spektren von Atomen und Ionen mit ein, zwei und drei Valenzelektronen II, bls. 21. Sennilega er um að ræða fyrstu myndina af þessu tagi, sem birtist í íslensku riti.
Það er eftirtektarvert, að í umfjöllun sinni um atóm og litróf styðst Trausti nær eingöngu við gömlu skammtafræðina, sem byggir á hinum byltingarkenndu hugmyndum Níelsar Bohr um innri gerð atóma frá 1913. Í grein sinni segir hann meðal annars (bls. 35):
Vér höfum séð hér að framan að Bohr getur skýrt með fyllstu nákvæmni litrof vetnisins og aðaldrættina í litrofi alkalimálmanna. Atommyndin hefir og reynst ágætlega nothæf til að skýra flóknari litrof í stórum dráttum, enda þótt nákvæmum reikningi verði sjaldnast við komið. Þess vegna hefir ekki verið hikað við að byggja ályktanir um ástand efnanna á öðrum hnöttum á atommynd Bohrs.
Á seinni árum hafa „quantamekanik“ Heisenbergs og bylgju-„mekanik“ Schrödingers komið til sögunnar og gefið miklu fyllri skýringu á litrofunum en Bohr.
En það sem Heisenberg og Schrödinger hafa fram yfir Bohr við grundvöllun aðferðanna og nákvæmni reikningsins, vinnur Bohr upp með glöggri mynd af atominu, sem gefur réttar niðurstöður í aðalatriðum. Verður því hér á eftir ávallt gengið út frá hugmyndum Bohrs.
Til gamans má skjóta hér inn, að það var frændi Trausta og nafni, Trausti Ólafsson efnaverkfræðingur, sem birti fyrstu íslensku greinina um hina nýju atómeðlisfræði Bohrs árið 1925: Um atomkenningu Bohr’s.
Sólin
Meira en helmingur greinar Trausta fjallar um það, hvernig nota má litófsmælingar til að ákvarða efnasamsetninguna í ystu lögum sólarinnar og eðlisástand efnisins þar, meðal annars hitastig þess, þrýsting og jónunarstig.
Samkvæmt lögmáli Wiens er yfirborðshiti sólarinnar í kringum 6000 Kelvinstig. Trausti bendir einnig á, að reikningar Eddingtons sýni að miðjuhitinn sé um 40 milljón gráður. Atómin hljóti því að vera jónuð og efnið í ástandi rafgass, allt frá miðju til yfirborðs. Sjá megi greinileg merki þess í litrófi sólarinnar. Slíkt sé einnig hægt að reikna út með hjálp hinnar svonefndu Saha-jöfnu, sem Indverjinn Meghnad Saha leiddi út fyrstur manna árið 1920.
Meghnad Saha ásamt hinni frægu jöfnu sem við hann er kennd. Ljósmyndin af Saha, sem fengin er að láni hjá Wikipediu, var tekin í kringum 1934. Myndin af jöfnu hans og meðfylgjandi skýringum er hins vegar úr greininni Hundred years of the Saha equation and astrophysics eftir G. Gangopadhyay frá 2021, bls. 497. Þar má t.d. sjá, að jafnan á myndinni er jafngild þeirri, sem Trausti birtir á bls. 35 í grein sinni. – Jafna Saha er reyndar ekkert lamb að leika sér við, þegar rekina skal jónunarstig þungra frumefna við framandi aðstæður. Því fékk höfundur þessara orða að kynnast í rannsóknum sínum á árunum í kringum 1980, þegar hann var að basla við, að ákvarða eðlisástand ystu laganna í ósegulmögnuðum nifteindastjörnum (sjá greinina Structure of neutron star envelopes).
Trausti ræðir tiltölulega lítið um yfirborðsvirkni sólar og einbeitir sér frekar að undirliggjandi stöðugleika hennar. Eftir skemmtilega lýsingu á ástandi efnis og orku inni í þessum gífurlega heita miðhnetti sólkerfisins, segir Trausti (bls. 36):
Sólin er þannig geisilegt haf af glóandi lofttegundum; ofan á synda heillegustu atomin, en neðar finnum við aðeins atomhluta. Þar er eins og komið sé nær og nær sjálfri sköpuninni – þar eru frumefnin sjálf í smíðum.
Eðlislýsing sólarinnar nær vitanlega einkum til yfirborðsins, því enda þótt fara megi nærri um ásigkomulagið í undirdjúpunum, hlýtur sú vitneskja að byggjast á athugun yfirborðslaganna. Sólinni má frá þessu sjónarmiði skipta í tvo aðgreinda hluta, gufuhvolfið, sem er gagnsætt og hinn ógagnsæa glóandi hnött, sem gufuhvolfið hvílir á. Frá yfirborði þessa hnattar stígur samfellt ljós upp í gegnum gufuhvolfið, en atomin, sem verða á vegi ljóssins, sía úr því þær bylgjulengdir, sem þeim eru eiginlegar, setja m.ö.o. sinn stimpil á sólarljósið. Litrof sólarinnar verður þannig samfellt, með fjölda absorptionslína.
Trausti ræðir síðan all ítarlega um litróf, hvernig þau eru ljósmynduð og síðan rannsökuð. Þessu næst tekur hann gufuhvolf sólar til umræðu, en það er ysti hluti hennar eða hjúpurinn, sem umlykur hið sýnilega „sólaryfirborð“, ljóshvolfið.
Eins og Trausti lýsir (bls. 38) er hjúpurinn lagskiptur. Hafa ber í huga, að grein hans er nær 90 ára gömul og því er eðlilegt, að lýsingu hans beri ekki fullkomlega saman við það, sem best er vitað í dag (sjá yfirlitsmyndina hér fyrir neðan).
Línuritið sýnir hvernig hitastig breytist með hæð í yfirborðslögum sólarinnar. Fyrir ofan ljóshvolfið er lithvolfið, þá svokölluð hvolfahvörf og yst er kórónan. Mynd: Wikipedia.
Trausti fjallar í nokkrum smáatriðum um öll yfirborðslögin. Áhugaverðust finnst mér þó umfjöllun hans um kórónuna, sem hann kynnir til sögunnar undir lok greinarinnar (bls. 39):
Þrátt fyrir hina feikna loftþynningu efst í chromospherunni … er langt frá því að þar sé komið út í tómt rúm. Að minnsta kosti 700.000 næstu kílómetrarnir eru „íklæddir“ efni, sem að vísu er óskiljanlega þunnt, en gerir þó vart við sig með hinu daufa skini kórónunnar. Kórónan sést aðeins við almyrkva á sólu, þegar tunglið byrgir fyrir sólkringluna sjálfa og birta himinsins er orðin nægilega dauf.
Þrátt fyrir að nota síðan eina og hálfa síðu til að ræða kórónuna og eiginleika hennar, er eftirtektarvert, að Trausti minnist ekki einu orði á hina mikilvægu kórónusjá Lyots, hvað þá eigin rannsóknir henni tengdar. Áhugi hans á kórónunni leynir sér þó ekki.
Trausti ræðir meðal annars, hvernig „litsjáin skiptir kórónunni í þrjú belti“, sem við í dag köllum K kórónu, E kórónu og F kórónu (sjá línurit hér að framan). Á þessum tíma olli E kórónan mestum heilabrotum, þar sem ekki var hægt að tengja ljómlínur hennar við þekkt atóm með hefðbundnum aðferðum litrófsfræðinnar.
Eins og aðrir á þessum tíma, gerði Trausti ráð fyrir, að kórónan hlyti að vera kaldari en sólaryfirborðið (sem er um 6000 gráður). Hinar sérkennilegu ljómlínur E kórónunnar kæmu ekki frá nýju frumefni, kórónín, eins og áður hafi verið haldið fram, heldur hlytu þær að tengjast „óþekktu ionisationsástandi einhvers hinna gömlu frumefna“. Hann færir rök fyrir því, að kórónulínurnar séu svokallaðar „bannaðar línur“ frá þess konar efnum, en „mörg þeirra [séu] of lítið rannsökuð til að hægt sé að segja fyrir um [slíkar] línur.“
Gátan um hinar undarlegu ljómlínur E kórónunnar var ekki leyst fyrr en nokkrum árum eftir að grein Trausta kom út. Þá sýndi sænski eðlisfræðingurinn Bengt Edlén (1906-1993) fram á, eftir ábendingu frá Walter Grotrian árið 1939, að sterkustu línurnar koma frá margjónuðum járnatómum, Fe XIV (þ.e. Fe13+) og Fe X (þ.e. Fe9+) og það benti til þess, að kórónan væri nær þúsund sinnum heitari en sólaryfirborðið. Sú niðurstaða var fljótlega staðfest.
Hinn merki sænski verkfræðingur og eðlisfræðingur Hannes Alfvén (1908-1995) greip fréttirnar af uppgötvun Edléns strax á lofti og átti verulegan þátt í að gera þær heyrum kunnar, enda féllu þær vel að hugmyndum hans um mikilvægi rafgass í alheimi. Niðurstöður Edléns þóttu mikið afrek á sínum tíma, þótt nafn hans beri ekki oft á góma nú á dögum.
Til vinstri má sjá Bengt Edlén á skrifstofu sinni við Háskólann í Lundi, árið 1940. Myndin er tekin um það leyti, sem hann uppgöðvaði, að hiti sólkórónunnar er mældur í milljónum Kelvinstiga. Lengst til hægri á skrifborðinu má sjá reiknivélina, sem hann notaði við vinnu sína. – Til hægri er Hannes Alfvén, árið 1942. Myndir: Wikipedia.
Litróf sólkórónunnar, tekið við almyrkva á sólu, 11. júlí 2010. Járnlínurnar (Fe XIV = Fe13+ og Fe X = Fe9+) sjást greinilega, Allar vetnis-, helín- og magnesínlínurnar koma hins vegar frá efsta hluta lithvolfsins og sólstrókum. Mynd úr grein M. Druckmüllers: Slitless spectrum of the solar corona.
Greinin um litrófin og sólina er líklega skrifuð einhvern tímann á tímabilinu frá því Trausti kom heim frá Þýskalandi, vorið 1934, og þar til hann fór að kenna við Menntaskólann á Akureyri, haustið 1935. Færsluhöfundur veit lítið um ferðir hans á þessu eina og hálfa ári, fyrir utan það, að í maí 1935 fór hann í Vatnajökulsleiðangur með Jóhannesi Áskelssyni jarðfræðingi og í júlí sama ár endurvakti hann goshverinn Geysi og varð landsfrægur fyrir. Meira um það í komandi yfirliti (færslu 4b2). Þess ber þó að geta, að í þeirri færslu er megináhersla lögð á þau störf Trausta á Íslandi, sem á einhvern hátt tengdust stjarnvísindum, meðal annars kennslu, alþýðufræðslu og almanaksreikningum.
Trausti Einarsson haustið 1935. Myndin er fengin að láni úr minningargrein Ágústs Guðmundssonar.
* Stjarneðlisfræði og heimsfræði á Íslandi: Efnisyfirlit *
Ég man ekki til þess að hafa heyrt minnst á Þorstein Sæmundsson fyrr en á fögrum vordegi árið 1967. Þann dag gengum við bekkjarfélagarnir í 6S undir munnlegt stúdentspróf í stjörnufræði í MR. Kennari okkar í þeirri grein var Skarphéðinn Pálmason stærðfræðingur og prófdómarinn var Þorsteinn. Snemma um morguninn hafði það kvisast út meðal nemenda, að prófdómarinn væri ekki aðeins hámenntaður stjörnufræðingur við Háskóla Íslands, heldur jafnframt einstaklega nákvæmur og strangur í öllum sínum dómum. Ég var því með örlítinn hnút í maganum, þegar ég gekk að grændúkuðu prófborðinu og hef sjálfsagt ekki verið einn um það í nemendahópnum.
Prófið gekk þó ágætlega, þangað til ég var beðinn um að reikna út, hvað það tæki jörðina langan tíma að falla inn í sólina, ef hún staðnæmdist skyndilega á braut sinni. Ég notaði trikk, sem Skarphéðinn hafði minnst á í kennslustund (markgildið af brautarsporbaugi jarðar um sólu, þegar miðskekkjan stefnir á einn, er bein lína milli jarðar og sólar) og beitti síðan þriðja lögmáli Keplers til að finna falltímann (65 daga). Þorsteinn kvað svarið rétt, en vildi ekki samþykkja lausnaraðferðina. Af þessu spunnust nokkrar deilur, þar sem við Skarphéðinn stóðum saman gegn Þorsteini. Hann lét þó undan að lokum, með semingi þó.
Eg tel, að þessi litla dæmisaga lýsi nokkuð vel fyrstu kynnum margra af Þorsteini. Hann átti það til, ekki síst á yngri árum, að vera nokkuð snöggur upp á lagið, enda reglufastur og siðavandur maður með ákveðnar skoðanir og ófrávíkjanleg prinsipp. Á stundum gátu óvænt viðbrögð hans komið viðmælendum úr jafnvægi og jafnvel gert þá honum fráhverfa. Það var ekki fyrr en menn kynntust Þorsteini nánar, sem þeir áttuðu sig á, að þar fór í raun hinn vænsti maður með ríka réttlætiskennd og sterka sannleiksást. Hann var skarpgáfaður, rökvís og einbeittur heiðursmaður, sem vann öll sín verk af mikilli vandvirkni og var jafnframt ráðagóður með afbrigðum. Minnisgóður var hann og nákvæmni var honum í blóð borin. Hann var sannur vinur vina sinna og taldi það ávallt skyldu sína að upplýsa þá, sem og aðra samferðamenn, um vísindi sín, stjörnufræði, háloftafræði og tímatalsfræði. Þrátt fyrir að vera bæði viðkvæmur og hlédrægur að eðlisfari, var hann óhræddur við að tjá sig um margvísleg samfélagsmál, hvort heldur var í ræðu eða riti. Allar ritsmíðar hans, og þær eru margar, eru gagnorðar og fágaðar og bera þess glögg merki, að þar hélt einstaklega ritfær maður á penna.
Ég átti því láni að fagna að vinna í nánasta starfsumhverfi Þorsteins í um það bil fjóra áratugi og mat hann mikils, bæði sem starfsfélaga og vin. Þær svipmyndir, sem brugðið er upp hér á eftir eru þó, rúmsins vegna, lítið annað en stutt og gloppótt upptalning á nokkrum atriðum, sem mér hafa þótt hvað áhugaverðust á ferli hans. Aðrir eiga eflaust eftir að fjalla um fleiri mikilvæga þætti í lífi hans og starfi. Í þessu sambandi má einnig benda á vandaða vefsíðu Þorsteins sjálfs, þar sem meðal annars má finna ritaskrá hans, ferilskrá og minningar.
Háskólanám
Þorsteinn Sæmundsson var afburða námsmaður og margverðlaunaður sem slíkur. Frá átta ára aldri var stjörnufræði hans helsta áhugamál og þegar kom að háskólanámi, valdi hann að stunda hana sem aðalgrein til B.Sc. Honorsprófs við St. Andrews háskóla í Skotlandi, með stærðfræði, eðlisfræði og jarðfræði sem aukagreinar. Hann lauk því námi 1958 með ritgerðinni The Earth and the Sun – Solar-Terrestrial Relations.
Myndin sýnir Þorstein á skrifstofu aðalkennara síns, E.F. Freundlichs (sitjandi til hægri), í stjörnuturni St. Andrews háskóla. Freundlich var vel þekktur stjörnufræðingur á fyrri hluta tuttugustu aldar og hafði meðal annars, að beiðni Einsteins, gert (árangurslausar) tilraunir til að nema þyngdarlinsuhrif. Með þeim á myndinni er samstúdent Þorsteins, Elaine Williams. Á veggnum að baki Þorsteins hanga myndir af sólmyrkvanum fræga í Sobral árið 1919. Um stjörnufræðina í St. Andrews má lesa í grein A. Battens frá 2014: The Beginnings of Modern Astronomy at the University of St Andrews. Myndin er úr safni ÞS.
Að loknu B.Sc. prófi hóf Þorsteinn doktorsnám við Háskólann í London og fékk vinnuaðstöðu við stjörnuturn skólans í Mill Hill, þar sem leiðbeinandi hans, Clabon W. Allen, starfaði (sjá einnig hér). Doktorsritgerðin fjallaði um áhrif sólar á jörð, nánar tiltekið uppruna svokallaðra raðbundinna segulstorma, sem Þorsteinn varði árið 1962. Sama ár komu út eftir hann tvær vísindagreinar, sem talsvert var vitnað í á sínum tíma:
Þorsteinn við svokallaðan Radcliffe linsusjónauka í stjörnuturninum í Mill Hill árið 1959. Sjá í þessu sambandi eftirfarandi grein hans: Þegar ég fann Plútó. Mynd úr safni ÞS.
Norðurljósarannsóknir
Að loknu doktorsprófi kaus Þorsteinn að halda heim til Íslands í stað þess að stunda vísindarannsóknir erlendis. Hann hóf því störf við Eðlisfræðistofnun Háskólans árið 1963 með það fyrir augum að halda áfram rannsóknum á segulstormum og norðurljósum, eins og fram kemur í viðtali við hann í Morgunblaðinu í janúar 1963. Að beiðni Þorbjörns Sigurgeirssonar eðlisfræðings, tók hann jafnframt að sér rekstur segulmælingastöðvar-innar í Leirvogi, sem Þorbjörn hafði komið upp árið 1957 í tilefni Alþjóða-jarðeðlisfræðiársins.
Til að safna sem mestum upplýsingum um norðurljósin og hvetja Íslendinga til að fylgjast vel með þeim, gaf Þorsteinn út gagnlegan bækling um norðurljósaathuganir. Jafnframt sá hann lengi um rekstur norðurljósamyndavéla á Rjúpnahæð og við Egilsstaði.
Hér má lesa norðurljósakver Þorsteins í heild sinni.
Í þessu sambandi langar mig til að minnast á, að árið 1968 var ég í sumarvinnu hjá Þorsteini við talningar á mismunandi gerðum norðurljósa á myndskeiðum frá kvikmyndavélinni á Rjúpnahæð. Ég skrifaði forrit til að vinna úr mælingunum og skilaði niðurstöðunum til Þorsteins. Löngu seinna, árið 2012, birti hann eitt af línuritunum, sem ég hafði lagt drög að sumarið 1968, mér til mikilar ánægju:
Línuritið, sem Þorsteinn birti í greininni Norðurljós árið 2012. Sjá einnig grein hans Norðurljós - fróðleiksbrot frá sama ári.
Þorsteinn átti samvinnu við ýmsa erlenda rannsóknarhópa um háloftarannsóknir, þar á meðal á norðurljósum. Þeirra viðamest var rannsóknarsamvinna við japanska vísindamenn, sem komu hingað til lands af þeirri ástæðu, að Ísland var við endann á segulsviðslínu, sem náði alla leið til mælistöðva Japana á Suðurskautslandinu. Þorsteinn aðstoðaði Japanina meðal annars við að koma upp þremur mælistöðvum hér á landi og túlka með þeim mælingarnar. Úr þessu samstarfi kom fjöldi vísindagreina, sem taldar eru upp í ritaskrá Þorsteins.
Þorsteinn og Guðný, kona hans, ásamt nokkrum japönskum samstarfsmönnum, snæða kvöldverð að japönskum sið í Tokyo árið 1987. Sjá nánar hér. Mynd úr safni ÞS.
Leirvogsstöðin
Stór hluti af vinnutíma Þorsteins fór í að sinna segulmælingastöðinni í Leirvogi. Stöðin gekk í gegnum miklar breytingar eftir að Þorsteinn tók að sér umsjón hennar árið 1963, eins og sjá má í annál, sem hann tók saman árið 2022. Þegar hann fór á eftirlaun 2005 tók Gunnlaugur Björnsson stjarneðlisfræðingur við rekstrinum.
Þorsteinn við mælingar í Leirvogsstöðinni 1986.
Einn helsti aðstoðarmaður Þorsteins var hin indæla Þorgerður Sigurgeirsdóttir, sem hér sést við vinnu sína árið 1986. Allir, sem hana þekktu, minnast hennar með söknuði.
Hluti mælitækjanna í Leirvogsstöðinni í lok sjöunda áratugs tuttugustu aldar. Sjá nánar á vefsíðu ljósmyndarans, Ágústs H. Bjarnasonar verkfræðings.
Þorsteinn og aðstoðarmaður hans, Pálmi Ingólfsson rafmagnstæknifræðingur, við vinnu í Leirvogsstöðinni í upphafi tuttugustu og fyrstu aldar.
Leirvogsstöðin, um það leyti sem Þorsteinn lét formlega af störfum árið 2005.
Íslandsalmanakið
Útreikningur og umsjón Almanaksins var veigamikill hluti af starfi Þorsteins, allt frá árinu 1963, fyrst í samvinnu við Trausta Einarsson stjörnufræðing, síðan einn og óstuddur í fjörutíu ár og undir lokin í samvinnu við Gunnlaug Björnsson stjarneðlisfræðing. Í ritstjórnartíð Þorsteins óx Almanakið og dafnaði, eins og sjá má með samanburði á fyrstu og síðustu útgáfunni, sem hann kom að.
Fyrsta almanakið, sem Þorsteinn hafði umsjón með, var fyrir árið 1964 og hið síðasta fyrir árið 2023. Nánar má lesa um sögu almanaksins hér.
Þorsteinn með eintak af Almanaki um árið 2014.
Þorsteinn ásamt Jóni Atla Benediktssyni rektor Háskóla Íslands (lengst til vinstri) og eftirmanni sínum Gunnlaugi Björnssyni. Þetta er líklega ein síðasta myndinn, sem tekin var af Þorsteini. Hún er frá því í mars 2023 og tilefnið var, að veittur hafði verið styrkur úr Almanakssjóði til að festa kaup á safni Íslandsalmanaksins frá upphafsárinu 1837 til ársins 1874. Þess má geta hér, að á veraldarvefnum má skoða öll almanökin frá árunum 1875-2000.
Tímatalsfræði
Eitt helsta viðfangsefni Þorsteins var tímatalsfræði, ekki síst sá hluti, sem snýr að íslensku tímatali, bæði fyrr og síðar. Skýrasta dæmið um störf hans á því sviði var umsjón hans með Íslandsalmanakinu, sem áður var minnst á. Árið 1972 gaf hann einnig út merkt uppsláttarrit, Stjörnufræði – Rímfræði, sem á sínum tíma kom eins og sending af himnum ofan til allra þeirra, sem þá kenndu stjörnufræði (og rímfræði) í íslenskum framhaldsskólum.
Forsíða bókar Þorsteins, Stjörnufræði – Rímfræði, frá 1972. Um útgáfuna má lesa hér.
Í ritaskrá Þorsteins er talinn upp fjöldi annarra ritsmíða um tímatalsfræði. Sem dæmi má nefna eftirtaldar greinar:
Klukkan á Íslandi
Árið 1968 hafði Þorsteinn forgöngu um breyttan tímareikning á Íslandi í þá veru, að hér eftir ættu klukkur á landinu að vera stilltar eftir miðtíma Greenwich allt árið. Þetta var samþykkt á Alþingi og fyrirkomulagið hefur haldist óbreytt síðan. Óhætt mun þó að fullyrða, að aldrei hafi ríkt fullt sátt um málið meðal landsmanna. Það hefur því gerst oftar en einu sinni, að komið hafa fram lagafrumvörp og þingsályktunartillögur ásamt meðfylgjandi greinargerðum um breytingar á núverandi fyrirkomulagi. Eins og flestum mun kunnugt, hafa forsvarsmenn slíkra breytinga ekki enn haft erindi sem erfiði. Yfirlit um þessa sögu má finna hér.
Þessi skopmynd birtist í Morgunblaðinu, 12. apríl 1984, og sýnir Vilhjálm Egilsson, þá alþingismann, vekja Þorstein af værum blundi. Tilefnið var grein eftir Þorstein með titlinum Sumartíð í blómagarði Alþingis, þar sem fjallað var um þingsályktunartillögu Vilhjálms og fylgismanna hans.
Stjörnuskoðunarfélag Seltjarnarness
Vorið 1976 var Stjörnuskoðunarfélag Seltjarnarness stofnað og með því fengu íslenskir áhugamenn um stjörnufræði loksins vettvang til þess að koma saman og ræða hugðarefni sín. Frumkvæðið að þessu átaki átti þúsundþjalasmiðurinn Sigurður Kr. Árnason og naut hann þar mikilvægrar aðstoðar Þorsteins, sem sá um að velja bæði sjónauka og hvolfþak fyrir félagið. Jafnframt tók Þorsteinn að sér formensku í félaginu fyrsu árin (sjá meira um þau ár hér og hér). Hann sagði einnig frá stofnun félagsins í Raunvísi, skammlífu fréttabréfi Raunvísindastofnunar Háskólans, sem hann ritstýrði.
Stjörnuturn Stjörnuskoðunarfélagsins á þaki Valhúsaskóla á Seltjarnarnesi. Mynd: Snævarr Guðmundsson.
Fyrsta stjórn Stjörnuskoðunarfélags Seltjarnarness á fjörutíu ára afmæli félagsins árið 2016. Frá vinstri: Sigfús Thorarensen, Sigurður Kr. Árnason og Þorsteinn Sæmundsson. Sjá nánar hér.
Ljósbrot í lofthjúpi jarðar
Ein af þekktustu greinum Þorsteins á alþjóðavettvangi var birt í tímaritinu Sky and Telescope árið 1986. Þar setti Þorsteinn fram einfalda og nákvæma nálgunarformúlu fyrir ljósbrotið í andrúmsloftinu. Formúlan er talsvert notuð og er við hann kennd.
Sjá nánari umfjöllun um ljósbrotsformúlu Þorsteins í grein hans frá 1986: Atmospheric Refraction.
Sólmyrkvar
Frá fyrstu tíð lagði Þorsteinn mikla áherslu á að fylgjast með áhugaverðum fyrirbærum á himni, bæði að nóttu sem degi. Þar má meðal annars nefna fyrirbæri eins og norðurljós, vígahnetti og loftsteinadrífur, en ekki síður stjörnumyrkva og tunglmykva, svo ekki sé talað um hina tilkomumiklu sólmyrkva.
Myndin sýnir Þorstein í hópi bandarískra áhugamanna um sólmyrkva í frægri flugferð yfir hafið milli Íslands og Grænlands. Hann skipulagði ferðina til að geta fylgst með sólmyrkvanum 3. október 1986. Sjá nánari umfjöllun um þetta mikla ævintýri hér og hér.
Síðasti sólmyrkvi tuttugustu aldarinnar varð 12. ágúst 1999. Hann sást meðan annars í Reykjavík sem deildarmyrkvi og af því tilefni setti Þorsteinn upp aðstöðu fyrir starfsmenn Raunvísindastofnunar Háskólans til að fylgjast með uppákomunni. Myndin sýnir Þorstein og samstarfsmann hans, Jón Sveinsson rafmagnstæknifræðing, sitt hvoru meginn við öflugan linsusjónauka í eigu Þorsteins. Í bakgrunni standa nokkrir af starfsmönnum stofnunarinnar. Frásögn Morgunblaðsins af myrkvanum má finna hér, hér og hér.
Vorið 2003 kom bandaríski stjörnufræðingurinn, Jay M. Pasachoff, hingað til lands til að fylgjast með hringmyrkva á sólu, sem varð að morgni 31. maí 2003 (sjá hér og hér). Þessa mynd af okkur Þorsteini tók Pasachoff að loknum hádegisverðarfundi, sem haldinn var á Hótel Sögu í tilefni heimsóknarinnar. Í bakgrunni má sjá grilla í hvolfþak kennslusjónauka Háskóla Íslands á þaki Árnagarðs.
Stjarnvísindafélag Íslands
Þorsteinn var einn af stofnfélögum Stjarnvísindafélags Íslands og sótti hvern einasta fund þess, allt þar til hann fór á eftirlaun. Hann tók að sér ýmis mikilvæg verkefni fyrir félagið og var formaður þess um tíma.
Aðalfundur Stjarnvísindafélagsins í Norræna húsinu í desember árið 1998. Í gluggaröðinni á efri myndinni sitja frá vinstri: Vilhelm S. Sigmundsson, Þórir Sigurðsson, ósýnilegi maðurinn, Þorsteinn Vilhjálmsson og Gunnlaugur Björnsson. - Á neðri myndinni eru frá vinstri talið: Þorvaldur Ólafsson, Karl H. Jósafatsson, Lárus Thorlacius, Þorsteinn Sæmundsson og Margrét Ó. Björnsdóttir. Fyrir framan þau, yfir kollinum á Vilhelm, má sjá leifarnar af einkennistákni aðalfunda félagsins, hinni gómsætu rjómatertu. Ljósmyndari: Einar H. Guðmundsson.
Norræni stjörnusjónaukinn
Segja má, að straumhvörf hafi orðið í stjarnvísindarannsóknum Íslendinga í júlí árið 1997, en þá gerðist Háskóli Íslands aðili að norrænu samstarfi um stjörnusjónauka á La Palma, einni af Kanaríeyjunum. Stjarnvísindafélag Íslands hafði frumkvæði að aðildarumsókninni og meðal þeirra, sem áttu hvað mestan þátt í að koma málinu farsællega í höfn, var Þorsteinn Sæmundsson. Hann var jafnframt fullrúi Íslands í stjórn sjónaukans í nokkur ár.
Norræni sjónaukinn á La Palma
Björn Bjarnason menntamálaráðherra, Sveinbjörn Björnsson rektor Háskóla Íslands og Johannes Andersen stjórnarformaður NOTSA undirrita samninginn um aðild Íslands að samstarfinu um Norræna stjörnusjónaukann í júlí 1997. Að baki ráðamönnunum standa þeir Gunnlaugur Björnsson, Einar H. Guðmundsson og Þorsteinn Sæmundsson.
Nýyrðasmíð og orðanefndarstörf
Þorsteinn hafði alla tíð mikinn áhuga á íslensku máli og eftir hann liggur fjöldi nýyrða, einkum í stjörnufræði og tölvunarfræði. Þá smíðaði hann ýmis nýyrði í eðlisfræði og efnafræði. Hann var mikilvirkur meðlimur í orðanefnd Skýrslutæknifélags Íslands á árunum 1978 til 2013 og einn af höfundum Tölvuorðasafns félagsins. Hann sat og í Íslenskri málnefnd árin 2002-2005.
Mörg nýyrða hans í stjarnvísindum komu fyrst á prenti í bókinni Stjörnufræði – Rímfræði árið 1972. Hann var formaður orðanefndar Stjarnvísindafélags Íslands frá upphafi (1990) til dauðadags, og sá um prentútgáfu Orðaskrár úr stjörnufræði árið 1996.
Frá því hún fyrst kom út, hefur orðaskráin verið uppfærð reglulega, og nú má ávallt finna nýjustu útgáfuna á vef almanaksins: Orðaskrár úr stjörnufræði: Ensk-íslensk & Íslensk-ensk.
Alþýðufræðsla
Þorsteinn Sæmundsson var tvímælalaust fremsti alþýðufræðari Íslands í stjörnufræði, háloftafræði og tímatalsfræði á seinni hluta tuttugustu aldar. Afköst hans á því sviði voru sannast sagt ótrúleg, eins og sjá má með því að renna í gegnum ritaskrá hans á vefnum. Þótt ýmsar ritsmíðarnar séu stuttar og fyrst og fremst ætlaðar lesendum almanaksins til fróðleiks, þá eru þær, eins og hinar lengri, einstaklega vel unnar, hnitmiðaðar og auðlesnar.
Fyrir mína parta held ég mest upp á tvö af alþýðuritum Þorsteins. Annað er stjörnufræðihlutinn í Stjörnufræði – Rímfræði frá 1972, hitt er ritgerðin Drög að heimsmynd nútímans, sem kom út árið 1966, rétt um það leyti, sem byltingin mikla í vísindalegri heimsfræði var að hefjast fyrir alvöru. Aldur verkanna dregur þó ekki úr gildi þeirra í mínum huga. Bæði gefa þau einstaklega gott yfirlit yfir stöðu stjörnufræði og heimsfræði á þeim mikilvægu árum í lok sjöunda áratugs síðustu aldar, þegar ég var að hefja háskólanám mitt í raunvísindum.
Að lokum tek ég mér það bessaleyfi, að birta afrit af skemmtilegri ljósmynd, sem Ragnar Axelsson ljósmyndari tók af Þorsteini og Morgunblaðið birti með greininni Undur og ógnir himingeimsins í nóvember 1992.
Þorsteinn Sæmundsson og stjörnuhiminninn, veturinn1992.
Þessi ritsmíð inniheldur lítið annað en fátæklega heimildaskrá, ásamt mislöngum minnispunktum. Viðfangsefnið er saga efnafræðinnar á Íslandi á tímabilinu frá síðmiðöldum til loka sjöunda áratugs tuttugustu aldar. Árið 1970 er hér valið sem endapunkur, því segja má, að þá hafi runnið upp nýir tímar í rannsóknum og kennslu í efnafræði við Háskóla Íslands. Aðrir munu væntanlega gera þeirri sögu skil, þótt síðar verði.
Efnið er tekið saman í nokkrum flýti og er fyrst og fremst ætlað mér sjálfum til minnis. Öðrum er þó heimilt að nota skrána eftir þörfum. Í staðinn þigg ég með þökkum allar ábendingar um villur og æskilegar viðbætur, auk upplýsinga um frekari heimildir og annað efni, er tengist sögu efnafræði hér á landi, einkum fyrir 1970.
Fjallað er stuttlega um þetta tímabil í færslunni
þar sem jafnframt má finna ýmsar heimildir.
Myndin að ofan sýnir O. Borch (1626-1690), fyrsta prófessorinn í efnafræði við Háskólann í Kaupmannahöfn. Hann var jafnframt prófessor í læknisfræði, grasafræði og textafræði og líflæknir konungs. Vitað er um að minnsta kosti þrjá Íslendinga, sem námu í Kaupmanna-höfn og áttu samskipti við Borch með einum eða öðrum hætti. Fyrst skal nefna þá Þorkel Arngrímsson Vídalín (1629-1677) og Vísa-Gísla Magnússon (1621-1696), sem báðir voru vel að sér í efnafræði þess tíma. Eftir Kaupmannahafnardvölina stunduðu þeir báðir frekara nám í efnafræði/efnaspeki sem og námufræði, Þorkell í Hollandi og Noregi, Gísli í Hollandi og á Englandi. Þá mun Gísli Vigfússon skólameistari og bóndi (1637-1673) einnig hafa átt talsverð samskipti við Borch.
Eins og tíðkast hafði frá siðaskiptum, sóttu lærðir Íslendingar á átjándu og nítjándu öld menntun sína fyrst og fremst til Háskólans í Kaupmannahöfn.
Náttúruspeki (eðlisfræði og efnafræði) upplýsingartímans barst fyrst til skólans með hinum fjölhæfa þýska náttúruspekingi og lækni, C.G. Kratzenstein, sem varð prófessor við Hafnarháskóla árið 1753. Hann innleiddi sýnitilraunir í náttúruspekikennsluna og gaf fljótlega út áhrifamikla kennsluók á latínu. Upp úr henni skrifaði hann síðar einfaldara yfirlitsrit á þýsku, Vorlesungen über die experimental Physik, sem kom í mörgum útgáfum á seinni hluta átjándu aldar og að lokum í danskri þýðingu, árið 1791. Með „experimental Physik“ var bæði átt við eðlisfræði og efnafræði. Frekari efnafræðikennsla á þessum tíma tengdist einkum læknisfræði og lyfjagerð og var hún ætluð læknanemum og verðandi apótekurum.
Kratzenstein kenndi nemendum, að efnið væri samsett úr atómum (sjá t.d. bls. 14 í Vorlesungen), en notaði þá hugmynd lítið sem ekkert í verkum sínum eða kennslu. Hann aðhylltist hina gömlu efnafræði G.E. Stahls (1659-1734), þar sem eldefnið (flogiston) kemur víða við sögu. Kratzenstein var þess vegna fullur efasemda um byltingakenndar hugmyndir hins franska A.L- Lavoisiers (1743-1794), þegar fréttir af þeim bárust til Kaupmannahafnar á níunda áratug átjándu aldar. Ekki leið þó á löngu þar til hin nýja efnafræði hafði rutt sér til rúms í Danmörku.
Meðal Íslendinga, sem lærðu hjá Kratzenstein, voru þeir Bjarni Pálsson (1719-1779), sem árið 1760 varð fyrsti landlæknir á Íslandi, og Jón Sveinsson (1753-1803), sem tók við af Bjarna 1780. Sennilega hefur fyrsti íslenski apótekarinn, Björn Jónsson (1738-1798) einnig sótt tíma hjá Kratzenstein. Eins og áðurnefndir læknar, notaðist hann við verkið Pharmacopoea Danica, sem þeir Kratzenstein og apótekarinn J.D. Cappel (1717-1784) áttu mestan heiðurinn af og kom út 1772. Enn einn nemandi Kratzensteins var dansk-íslenski læknirinn Pétur Thorstensen (1752-1792), síðar fyrsti prófessorin í náttúruspeki við námuskólann á Kóngsbergi í Noregi.
Magnús Stephensen og Sveinn Pálsson
Meðal annarra þekktra íslenskra nemenda Kratzensteins voru þeir Magnús Stephensen (1762-1833) og Sveinn Pálsson (1762-1840). Sá síðarnefndi á heiðurinn af fyrstu prentuðu greininni um efnafræði á íslensku, Um kalkverkun af jörðu og steinum, sem birtist árið 1788 í 9. bindinu af ársriti, sem Hið (konunglega) íslenska lærdómslistafélag gaf út á árunum 1781-1798. Sem kunnugt er markaði þessi mikilvæga útgáfustarfsemi tímamót í íslenskri menningarsögu, ekki síst á sviði alþýðufræðslu.
Örlítið brot úr ritgerðinni um kalkverkun (bls. 96). Þar notar Sveinn orðið bræðslufræði (Chemie) fyrir þá grein, sem við nú köllum efnafræði. Með hughtakinu elementjarðtegund (Terra elementaris) er vísað til hugmyndarinnar um hinna fornu höfuðskepnu, jörð.
Grein Sveins byggir meðal annars á gömlu eldefniskenningunni, enda vitnar hann oft í verk Kratzensteins. Einnig notast hann víða í greininni við yfirlitsrit Danans N. Tychsens (1751-1804) frá 1784, Chemisk Haandbog I, II & III, sem mun hafa verið til í mörgum eintökum hér á landi og talsvert notuð. Þá vitnar hann í ýmsa aðra höfunda, svo sem danska steindafræðinginn M.T. Brünnich (1737-1827) og hina þekktu sænsku efnafræðinga A.F. Cronstedt (1722-1765) og T. Bergman (1735-1784). Þess má geta hér, að Danir stóðu Svíum talsvert að baki í efnafræði á átjándu öld, enda var Svíþjóð þá stórveldi á því sviði, meðal annars vegna mikillar námuvinnslu í landinu.
Sveinn skrifaði fleiri greinar í Rit lærdómslistafélagsins, þar á meðal Um husblas eða sundmagalím (9. bindi, 1788) og Um sápusuðu (10. bindi, 1789).
Magnús Stephensen lærði náttúrspeki hjá Kratzenstein á undan Sveini, en síðar átti hann einnig eftir að sækja fyrirlestra hjá H.C. Örsted (1777-1851) í heimsóknum sínum til Kaupmannahafnar.
C.G. Kratzenstein (1723-1795) til vinstri og H.C. Örsted (1777-1851).
Sveinn Pálsson (1762-1840) til vinstri og Magnús Stephensen (1762-1833).
Í greinum sínum um náttúruspeki kýs Magnús að nota heitið samblandsfræði (í stað bræðslufræði) yfir það, sem við nú köllum efnafræði. Hann rökstyður valið í greininni Um járn og stál frá 1818, þannig (bls. 105):
Chemie [...], þess[i] dýrðleg[a] grein náttúrufræðinnar, án hverrar enginn má náttúrufróður nefnast. Hún kennir að þekkja allra hluta undirstöðuefni, að rannsaka þeirra sambland, og að aðskilja þeirra parta í einföld upprunaefni (element). Líka að blanda mörgum þeirra saman aftur til líkrar veru, og ummynda þá í aðrar með þeirri samblöndun. Þar þetta skeður oft án hita eða bræðslu, með samblandi ýmislegra hluta, jafnvel kaldra, eða svo, að ekki megi bræðsla nefnast, finnst mér orðið samblandsfræði næst koma að lýsa eðli þessarar lærdómsgreinar.
Ári síðar skrifaði Magnús aðra ágætis grein, Helztu lopt-tegundir I, II & III í hið þekkta mánaðarrit sitt, Klausturpóstinn.
Björn Gunnlaugsson (1788-1876) hóf kennslu í eðlisfræði við Reykjavíkurskóla, strax við stofnun hans, árið 1846, og Benedikt Sveinbjarnarson Gröndal (1826-1907) mun hafa kennt einhverja efnafræði þar í náttúrufræðitímum á árunum 1876-1893. Eftir því sem ég veit best, var þetta í fyrsta sinn, sem þessar greinar voru kenndar við latínuskóla á Íslandi. Formleg kennsla í efnafræði mun þó ekki hafa hafist í Reykjavíkurskóla fyrr en 1907 og var þá notast við bókina Kemi for Mellemskolen eftir Danann Th. Sundorph (1863-1926). Efnafræðikennsla jókst svo til muna í stærðfræðideild skólans, fljótlega eftir að hún var stofnuð, árið 1919.
Árið 1842 gaf Björn Gunnlaugsson, þá við Bessastaðaskóla, út hið fræga ljóð Njólu, þar sem hann setur meðal annars fram kenningu sína um frumagnir. Kenningin var byggð á hugmyndafræði hinnar svokölluðu kraftahyggju, sem kennari Björns í náttúruspeki við Háskólann í Kaupmannahöfn, H.C. Örsted, aðhylltist. Björn mun og hafa haldið þessum hugmyndum að nemendum sínum við Reykjavíkurskóla.
Tilraun færsluhöfundar til að gefa myndræna lýsingu á frumögn Björns Gunnlaugssonar. Sjá nánari umfjöllun hjá Einari H. Guðmundssyni, 2022, Kenning Björns Gunnlaugssonar um innsta eðli efnisins.
Læknaskólinn í Reykjavík 1876-1911
Efnafræði var kennd við Læknaskólann frá upphafi, 1876, og munu læknar hafa séð um kennsluna, þar til efnaverkfræðingurinn Ásgeir Torfason kom að skólanum, árið 1905. Meira um það hér á eftir.
Íslenskar kennslubækur og alþýðurit um efnafræði
Náttúrufræðingurinn og skáldið, Benedikt Sveinbjarnarson Gröndal, varð fyrstur til að semja og þýða bækur um efnafræði á íslensku. Þar skal fyrst telja Steinafræði og jarðarfræði frá 1878, sem inniheldur meðal annars ágæta umfjöllun um litrófsgreiningu (bls. 36-37). Árið eftir þýddi hann alþýðuritið Efnafræði, eftir hinn þekkta breska efnafræðing, H. E. Roscoe (1833-1915). Þá samdi hann sjálfur læsilegt rit, Efnafræði, og gaf út árið 1886. Aftast í þeirri bók er skemmtilegt yfirlit yfir sögu greinarinnar (bls. 67-76).
Mikið vatn hefur til sjávar runnið, frá því Benedikt skrifaði um efnafræði fyrir landa sína og fjöldi annarra bóka um þau fræði hafa síðan komið út á íslensku. Skrá yfir nokkrar slíkar má finna hér:
Í stað inngangsorða sjá:
Talsvert var fjallað um geislavirk efni hér á landi í byrjun tuttugustu aldarinnar, bæði af læknum og Þorkatli Þorkelssyni eðlisfræðingi. Um er að ræða ritsmíðar, sem ég hef lítið kannað, og verða þær því ekki ræddar frekar hér.
Fyrstu háskólamenntuðu efnafræðingarnir
Til skamms tíma hefur ekki verið gerður mikill greinarmunur á efnafræðingum og efnaverkfræðingum hér á landi. Fyrstu íslensku efnafræðingarnir voru nær allir efnaverkfræðingar og lengi vel lærðu flestir þeirra við Fjöllistaskólann (Den Polytekniske Læreanstalt) í Kaupmannahöfn. Hann var settur á laggirnar árið 1829 og hélt nafninu til 1933, þegar því var breytt í Danmarks Tekniske Højskole (DTH). Frá 1994 hefur stofnunin svo borið heitið Danmarks Tekniske Universitet (DTU). Helstu efnafræðikennarar Fjöllistaskólans kenndu einnig flestir, ef ekki allir, við Háskólann í Kaupmannahöfn.
Sjá einnig:
Verkleg kennsla í efnafræði við danska Fjöllistaskólann í kringum 1900. Sjá nánar hér.
Fyrstu íslensku efnaverkfræðingarnir voru þeir Ásgeir Torfason (1871-1916), sem lauk námi 1903, og Trausti Ólafsson (1891-1961), sem útskrifaðist 1921.
Efnaverkfræðingarnir Ásgeir Torfason (1871-1916) til vinstri og Trausti Ólafsson (1891-1961). Ljósmyndarar óþekktir.
Efnarannsóknastofa ríkisins
Fyrsta opinbera efnarannsóknarstofa Íslendinga tók til starfa árið 1906. Frumkvæðið áttu Björn Jensson kennari (1852-1904), læknarnir Guðmundur Björnsson (1864-1937) og Guðmundur Magnússon (1863-1924) og Michael Lund lyfsali (1873-1949).
Fyrsta aðstaða Efnarannsókastofu ríkisins var í húsi Búnaðarfélags Íslands í Lækjargötu, þar sem Iðnskólinn var einnig staðsettur um tíma. Mynd: C. Nielsen, 1906.
Ásgeir Torfason var fyrsti forstöðumaður stofunnar. Hann dó árið 1916 og við stjórninni tók Gísli Guðmundsson gerlafræðingur (1884-1928). Hann var forstöðumaður, þar til Trausti Ólafsson tók við árið 1921. Efnarannsóknastofa ríkisins varð á endanum hluti af Iðnaðardeild Atvinnudeildar Háskólans, þegar hún var sett á laggirnar árið 1937.
Nokkrar aðrar stofnanir með áherslu á efnafræði
Sjá eftirfarandi heimildir:
Efnafræðikennsla í Læknadeild Háskólans
Við stofnun Háskóla Íslands, árið 1911, varð Læknaskólinn í Reykjavík að Læknadeild, einni af fjórum deildum Háskólans. Kennararnir fylgdu með, þar á meðal Ásgeir Torfason, sem hélt áfram að sjá um efnafræðina. Eins og tíðkast hafði frá 1906, fóru verklegu æfingarnar fram í húsakynnum Efnarannsóknastofu ríkisins og í kennslunni notaði Ásgeir sömu kennslubækurnar og áður, Grundtræk Af Den Uorganiske Kemi og Grundtræk Af Den Organiske Kemi eftir danska efnafræðinginn O.T. Christensen (1851-1914). Þessar bækur þóttu greinilega svo góðar, að þær voru notaðar áfram eftir að Ásgeir lést, haustið 1916. Ekki var breytt um kennslubækur fyrr en Trausti Ólafsson tók við efnafræðikennslunni í Læknadeild, árið 1921.
Vert er að hafa í huga í þessu sambandi, að Christensen var á margan hátt merkur efnafræðingur. Hann var til dæmis fyrsti danski kennslubókahöfundurinn, sem kynnti dönskulesandi háskólanemum lotukerfi Mendelejevs (1834-1907) og útskýrði mikilvægi þess í efnafræði.
Trausti Ólafsson (fremstur fyrir miðju, í aðeins dekkri slopp en hinir) ásamt læknanemum fyrir framan Efnarannsóknastofu ríkisins, sennilega árið 1926. Rannsóknastofan var þá í bakhúsi við Hverfisgötu 44 og þar fór efnafræðikennslan í Læknadeild Háskólans jafnframt fram. Sjá nánari umfjöllun hér.
Frekari heimildir um efnafræðikennsluna í Læknadeild er að finna í Árbókum Háskóla Íslands. Sjá einnig: Elín Ólafsdóttir og Baldur Símonarson, 1987: Biochemistry in Iceland.
Efnafræðikennsla á Verkfræði- og náttúruvísindasviði Háskólans
Helstu heimildir, sem ég þekki, um upphaf og sögu formlegs náms í verkfræði og náttúruvísindum við Háskóla Íslands, eru eftirfarandi greinar:
Heimildir um efnafræðikennslu í verkfræði- og náttúruvísindanámi við HÍ er að finna í Árbókum Háskóla Íslands. Sjá einnig: Elín Ólafsdóttir og Baldur Símonarson, 1987: Biochemistry in Iceland.
Svipmyndir frá árunum 1930 til 1970
Jón E. Vestdal efnaverkfræðingur (1908-1979) í Dresden í Þýskalandi, árið 1933. Sjá nánar hjá Jóni E. Vestdal (viðtal), 1933: Efnilegur efnafræðingur.
Guðmundur Arnlaugsson stærðfræðingur (1913-1996) við efnafræðikennslu í MA, veturinn 1938-39. Sjá nánar í Sögu Menntaskólans á Akureyri 1880-1980, 2. bindi, bls. 147.
Sigurkarl Stefánsson stærðfræðingur (1902-1995) við verklega kennslu í MR á árunum 1943-44. Á myndinni er hann annar frá hægri. Sjá nánar hér.
Mynd af efnarannsóknarstofu Atvinnudeildar Háskólans árið 1955. Mynd úr grein Valtýs Steánssonar: Heimsókn í iðnaðardeild Atvinnudeildarinnar.
Geir Arnesen efnaverkfræðingur (1919-1991) við vinnu sína á Rannsóknastofu Fiskifélags Íslands í kringum 1960. Sjá nánar hjá Páli Ólafssyni, 1984: Hálfrar aldar starf: Rannsóknastofa Fiskifélags Íslands og Rannsóknastofnun fiskiðnaðarins, bls. 400.
Sigurður Steinþórsson jarðfræðingur (f. 1940) með tæki til snefilefnamælinga í Atvinnudeildarhúsinu, árið 1964. Sjá nánar hjá Guðmundi Sigvaldasyni (viðtal, 1964): Deild til jarðefnafræðirannsókna komið upp
Hús Raunvísindastofnunar Háskólans á opnunarárinu 1966. Frá þeim tíma hafa þar verið aðalstöðvar efnafræðinnar við verkfræði- og raunvísindadeildir Háskóla Íslands. Ljósmyndari óþekktur.
Þrír brautryðjendur í efnafræði við Háskóla Íslands
Steingrímur Baldursson
Steingrímur við kennslu á efstu hæð íþróttahúss Háskóla Íslands, sennilega árið 1961. Sjá nánar hér.
Steingrímur Baldursson (1930-2020) lauk BS-prófi í efnafræði frá Kaliforníuháskóla í Berkeley, árið 1952, og MS-prófi í sömu grein við Chicagoháskóla 1953. Hann var við nám og störf í kennilegri lághita-safneðlisfræði við Enrico Fermi Institute for Nuclear Studies við Chicago-háskóla á árunum 1953-1958. Þaðan lauk hann doktorsprófi í efnaeðlisfræði árið 1958 með ritgerðinni The Lambda-transition in Liquid Helium. Leiðbeinandi hans var prófessor J.E. Mayer. Ári síðar birtist eftir Steingrím greinin Theory of Bose‐Einstein Fluids, Statistical Mechanical Treatment of the Thermodynamic Properties of Liquid Helium.
Steingrímur kenndi efnafræði við Læknadeild Háskólans frá 1960, þar sem hann var prófessor. Árið 1974 var embætti hans fært til Verkfræði- og raunvísindadeildar, þar sem hann innleiddi meðal annars kennslu í eðlisefnafræði við efnafræðiskor.
Bragi Árnason
Bragi við massagreini Eðlisfræðistofnunar Háskólans, um miðjan sjöunda áratug tuttugustu aldar. Ljósmyndari óþekktur.
Bragi Árnason efnaverkfræðingur (1935-2017) lauk Dipl. Chem.-prófi frá Technische Hochschule í München í Þýskalandi árið 1961. Hann var sérfræðingur við Eðlisfræðistofnun Háskólans 1962-66 og við Raunvísindastofnun 1966-70. Dósent í efnafræði við HÍ 1970-76 og prófessor 1976-2005. Dr. scient frá HÍ 1976.
Sigmundur Guðbjarnason
Sigmundur við kennslu, árið 1973. Ljósmyndari óþekktur.
Sigmundur Guðbjarnason lífefnafræðingur (f. 1931) varð prófessor í lífrænni efnafræði og lífefnafræði við Háskóla Íslands árið 1970. Sem kunnugt er, var það upphafið að þróttmiklu uppbyggingarstarfi á sviði kennslu og rannsókna í efnafræði við skólann.
